- #1
BreezyLeaf
- 7
- 0
I've seen it often taught that the combination of two vectors is their graphical sum, but I think that there is a problem with this, in that if the vectors have opposite-facing components, then there are portions of the vectors completely neglected by the calculation. The idea seems to only be valid for parallel vectors, a case so narrow-in-applicability as to often be irrelevant.
Here's what I think is flawed about conventional vector combinations:
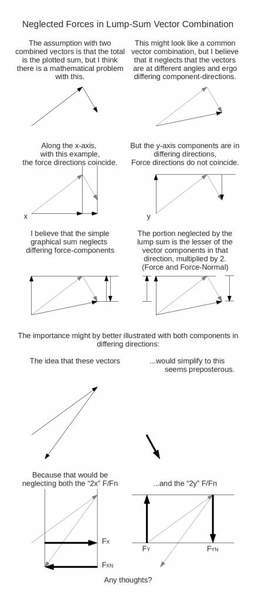
Please let me know your impression. Thank you.
Here's what I think is flawed about conventional vector combinations:
Please let me know your impression. Thank you.