I would like to know the calculation process of this power spectrum

[arcTomato]

TL;DR Summary

Derive the formula of power spectrum from Discrete Fourier Transform.

Summary: Derive the formula of power spectrum from Discrete Fourier Transform.

Hi all
I don't know where should I post this, so if I am wrong, I apologize.(But this is almost math problem so )

I would like to know the calculation process when derive Eq(6.3) in this paper.

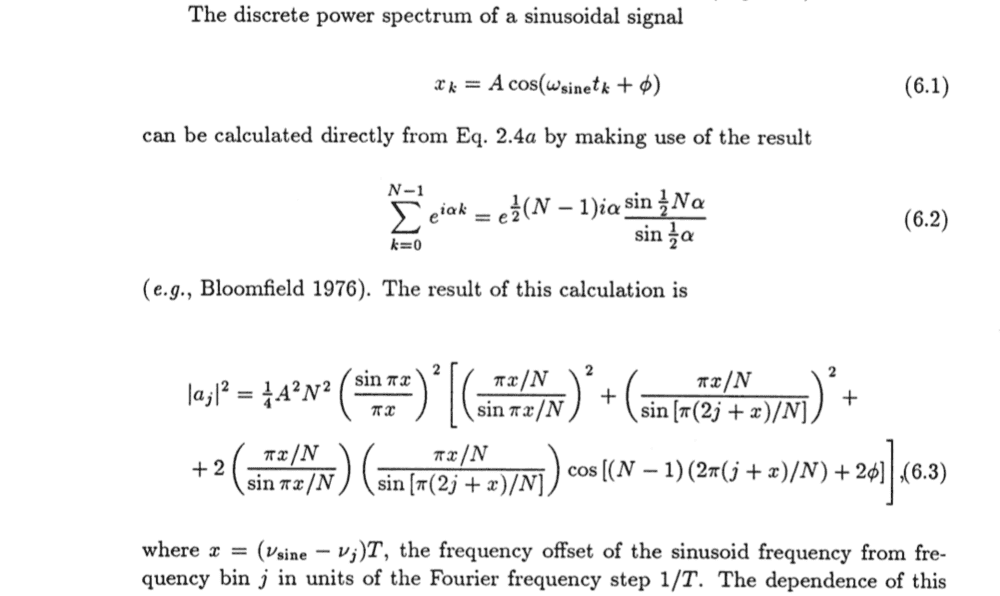Eq 2.4a is $a_{j}=\sum_{k=0}^{N-1} x_{k} e^{2 \pi i j k / N} \quad j=-\frac{N}{2}, \ldots, \frac{N}{2}-1$ and $t_k={kT/N}$, $ω_{sine}=2πν_{sine}$

I think these are the all tool to derive Eq.(6.3). But I don't have much calculation power to derive. (I spent two weeks for this.)
This will be a tough calculation process but if you can do this(and I know PF's teachers can do this :D), PLEASE HELP ME!

Thank you.

 

[jedishrfu]

Sadly, we can’t help you at all unless you show us your work in attempting to solve this.

 

[arcTomato]

Thank you for replying, @jedishrfu!
ok, at first. I should calculate $a_{j}=\sum_{k=0}^{n-1} A \cos \left(\omega_{sin} \frac{k T}{N}+\phi\right) e^{2 \pi i k / N}$.
I think I will use Eq(6.2),but I don't know how. I am already stuck here

 

[jedishrfu]

First, I have no idea how to solve this. However, looking at the summation I see the e function and the $x_k$ factors.

the x’s are defined in terms of cos() so have you tried representing the cos() in terms of the e function. Doing that might allow you to split the summation into two summations both of which could use the hint they provided at 6.2.

https://webhome.phy.duke.edu/~rgb/Class/phy51/phy51/node15.html

 

[arcTomato]

thank you @jedishrfu!
I have already tried before, and become so messy.
but I will try again!

 

[jedishrfu]

Calling @fresh_42 do you have any thoughts to help here?

The derivation looks quite daunting aka messy.

 

[fresh_42]

I have already tried before, and become so messy.

I guess you can't avoid this. It looks as if a big, big blackboard would be helpful.

2.4. gives the structure $a_j=\sum_k x_k e^{f(k)}$.
6.1. resolves the $x_k$.
To apply 6.2. we need to put every non constant coefficient into a power of $e$, which requires $\cos g= \frac{1}{2}\left(e^{ig}+e^{-ig}\right)$ where $g=g(t_k,\phi,\omega)$.
Now we have two sums and the form which is necessary to apply 6.2 and should be done, i.e. have only some algebra to do to finish it.

Finally we have 6.3. which has again a cosine term, which is a bit disturbing. I do not see how it could be saved without resolving the cosine into powers of $e$. I don't even see, whether 6.3. is true at all.

I would start and calculate some examples with e.g. N=3 and multiples of $\pi$ for the angles to see whether I can get 6.3. at all.

 

[arcTomato]

I guess you can't avoid this. It looks as if a big, big blackboard would be helpful.

Thanks for your helping guys! @jedishrfu ,@fresh_42
Finally, I finished it!
I have used 3 A4 papers

 

[jedishrfu]

You know in addition to a big blackboard youd also need hagoromo chalk. Mathematicians claim you can't make a mistake when using it.

Glad you figured it out.