Image appears outside of q -- Lens Maker's Equation confusion

[Albertgauss]

Summary: Does the image distance q in the lens-maker's equation mean where real images form or where any image at all forms? If the former, what separates the real image from all other reflections?

Hi all,

I attached a series of images taken with a camera for a concave mirror, the Pasco SE-7573.

Demonstration Mirror, Concave - SE-7573 - Products | PASCO

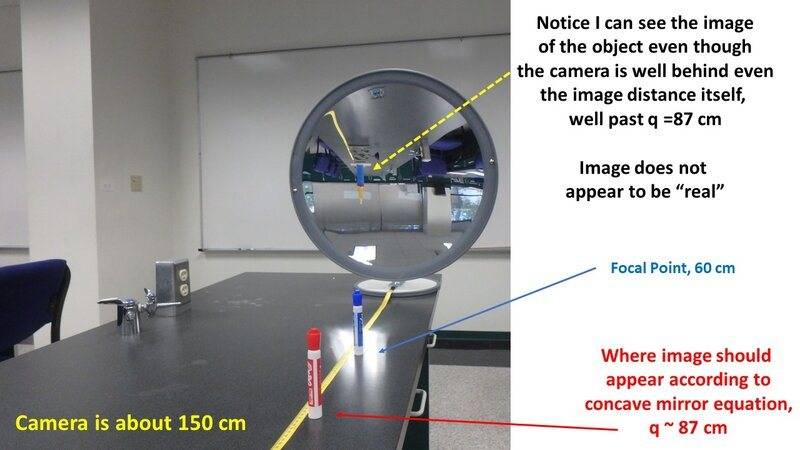
The parameters are f = 60 cm, p = 200 cm, and q should be ~ 87 cm, as calculated from the standard lens makers equation in a second semester university of college physics course. I measured the focal length crudely by moving the object back and forth and noting where it transitions from upside-down to right-side-up.

My question is: based on the lens maker’s equation, shouldn’t I see the image ONLY at q = 87 cm and at no other distance from the mirror? According to second semester ray diagrams, the ray lines do not converge at any other location except where the real image is supposed to form, and, not converging, it seems that no other image should be possible to be seen anywhere else.

Yet, no matter how close or far I go to the mirror, I can see an image of the object wherever I go, at the various distances from the mirror as shown below.

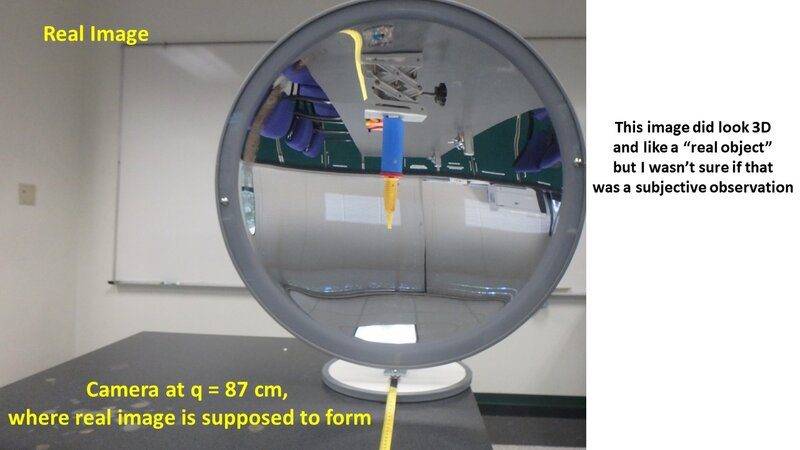
I do think that the real image that forms at 87 cm below does look different and three-dimensional in contrast to the other images I see when I move close to and away from the mirror; I include a picture taken at this distance, but I don’t know if I am stating this because I am supposed to feel that I see something different where the real image should be (based on prior knowledge) or if I really am seeing a different image at 87 cm as opposed to the images at all the other distances. I feel like "something" should be different for the reflection where I look at 87 cm compared to all the images formed everywhere else, but I am not sure what that "something different" is.

Why do clear images form of the object that are not at where the real image should form? I feel like no other image should form, except at the "q", and therefore the object should be invisible (not reflect), except at "q". If the rays don't focus at any other location, how are the images (reflections) at all distances from the mirror able to form so that you see a reflection of the object no matter how far you are from the mirror?

 

[kuruman]

You have to think a little bit and consider exactly what you see when you say you "see" the image. It is true that you always see an inverted image if you place your eye at any distance greater than the focal length. At 87 cm and image is formed that can be projected on a screen. If you don't put a screen there, you still see the image because the lens in your eye has a variable focal length that bends the rays and focuses them on your retina. You can redraw your ray diagram with a lens past the focal length and see how it works.

 

[Albertgauss]

Excellent, I am going to try that tomorrow. I'll let you know how it goes. That's a really useful criteria.

 

[Albertgauss]

Hi all,

The first image below shows the object at 75 cm for a concave mirror focal length of 50 cm. According to the lens makers equation, the real image should appear at 150 cm and the magnification should be 2. I replaced the object of the previous images with a nice, shiny light bulb (that I hoped would serve as a point-light) because I could not get the previous object to project on any screen, lights on or off.

The "splotch" you see on the board is actually the magnified interior of the light bulb. The projection happens at 90 cm, certainly not at the 150 cm it is supposed to be at. Also, the magnification is enormous, well above double, which is why you can see so much of the light bulb filament. The image is many times magnified above what it should be to see that kind of the light bulb detail.

Below is what you see when I placed at screen where the real image of the light bulb should be.

The real image of the light bulb focuses at only one location, the 90 cm of the previous image and all other images of all other distances are unfocused and magnify the farther back I move the screen. So, at least it seems consistent that there is only one location where the real image appears, but I don't understand why that image is not appearing where it should be, nor have the magnification it should have.

A few things:

Why doesn't the lens maker's equation work for this kind of mirror? It is supposed to be a concave mirror and should easily conform to the lens maker's equation.

Does anyone know of a mirror that really does work well with the lens makers equation? I would really like to work with it. Is the material a factor? Does anyone know something from engineering that they actually worked with that behaved as a concave mirror normally should? Is it possible this concave mirror is not spherical?

This is my progress so far.

 

[Mister T]

Is the focal length 50 cm or 60 cm? And how do you know? You can form a real inverted image located at twice the focal length if you place the object at twice the focal length. In other words, the image can be seen on a screen at the same location as the object if you place them at the center of curvature of the mirror. This technique can be used to verify or establish the focal length.

 

[Albertgauss]

Pretty sure the focal length was 60 cm, I can check again. I thought the images above were a typo when I looked at them just now in putting 50 cm where I meant to write 60 cm. Now I am not sure if my typo is not the correct focal length.

Its hard to see from the images but I am pretty sure that the object is not at twice the focal length (100-120 cm). Its at 75 cm. When you say "twice the focal length", do you meant the center of curvature, C, of the mirror? Unless of course my focal length is 30-40 cm; I'm pretty sure its not, less sure after this reply, though.

I measured the focal length crudely; where the image flips from upside down beyond the focal length to where it switches to right side up, closer to the mirror within the focal length. I did that as carefully as I could and should be within a few centimeters.

"You can form a real inverted image located at twice the focal length if you place the object at twice the focal length."

Yup, just verified it theoretically from the lens maker's equation. However, the magnification comes out to "1", that is, the image should neither have shrunk nor magnified with respect to the original object, and the image here is clearly well magnified.

"In other words, the image can be seen on a screen at the same location as the object if you place them at the center of curvature of the mirror."

The image and object are pretty close together, 75 compared to 90 cm. I'm not sure if this verifies the above statement or if the discrepancy is enough that this is what is not happening here.

I will check on Monday the focal lengths and see where I can get the object and image to appear at the same distance from the mirror. I think that's what's needed here.

 

[Mister T]

When you say "twice the focal length", do you meant the center of curvature, C, of the mirror?

Yes.

"You can form a real inverted image located at twice the focal length if you place the object at twice the focal length."

Yup, just verified it theoretically from the lens maker's equation. However, the magnification comes out to "1", that is, the image should neither have shrunk nor magnified with respect to the original object, and the image here is clearly well magnified.

The magnification is $-1$. The reason your image is larger than your object is because you don't have the object and image each located the same distance ($2f$) from the mirror.

I will check on Monday the focal lengths and see where I can get the object and image to appear at the same distance from the mirror. I think that's what's needed here.

That is what you need to do. You can also call PASCO and ask them what the focal length is.

 

[Albertgauss]

Here is the test I did. I moved the object and projector screen to as close to the same distance as I could.
The image is still magnified much more than the object, but I did all I could with a considerable amount of effort on this. Based on the below, I measured the Center of Curvature to be about 78 cm for about 39 cm focal length, certainly less than what I mentioned above with the more crude method of watching to see when the image flips from upside-down to right-side. The image is inverted with respect to the object.

I have not yet got an answer back from Pasco about what they think the focal length should be, or even if this mirror is a spherical mirror (I have been thinking that if this particular mirror is not spherical, then the len's makers equation might not apply).

 

[Mister T]

The image should be the same size as the object, and inverted. I suggest you use something like a small frosted lamp as the object. From your picture, I don't see evidence of an image, inverted or otherwise; and I can't tell if what I see is enlarged or reduced. What are you using for an object? What are you using for a screen?

Another experiment you can perform is to stand in a long hallway that has a glass doorway to the outside at one end. Hold the mirror such that you form an image of the doorway on a piece of paper held in a vertical plane parallel to the closed door. Now see if you can adjust the distance between the paper and the mirror such that distant objects located outside the doorway are in focus on the paper. The distance from the paper to the mirror is the focal length.

 

[Albertgauss]

"From your picture, I don't see evidence of an image, inverted or otherwise; and I can't tell if what I see is enlarged or reduced. What are you using for an object? What are you using for a screen?"

The object is the light bulb below, tuned to about 5 volts and glowing brightly. The light bulb is also placed on top of a lab stand jack. I chose a bright glowing light bulb because it would be easy to see (and hence its image easy to find). When I used the object of the previous post, the blue-yellow balloon, steeple-looking thing, I could not focus its real image on any screen. Can you upload a picture of the frosted lamp you have in mind?---I am certainly willing to try another object.

The screen in which to capture the light bulb image is white paper on the corkboard; that's the top blob of light in the top image. If I should use something different for a projector screen, by all means let me know. I use what is available to me, but would certainly be excited to know that another kind projector screen would make this much easier. I had not considered the material of the projector screen detail before. A part number would be great.

Also, what the camera and what the eye capture are very different. Neither the image or object are nearly as bright and spherical as what the camera captured above. The object, the light bulb, the lower blob of light in the image, does not look this way in person; it is a point-source light bulb as seen with the human eye. The upper image, the blob of light on my makeshift projector--that is the best and sharpest the image could focus at. Any other distance and the image gets larger, less bright, and, by human eye, much less focused. I think you would have to see this in person to be convinced.

Good idea on the other method; I will give that a try.

 

[Mister T]

It sounds like you have a proper object, screen, and technique. So I would suggest moving the object a bit further away and the screen a bit closer until you get a sharp image with object and image distances equal.

A frosted bulb is just the normal type of incandescent bulb we'd put in a reading lamp. It gives a more even glow with less glare than a bare bulb. But it sounds like you don't need that because you are seeing a clear image of your bare bulb.

I doubt you have any kind of aberration from the mirror's shape. If you suspect that it's not spherical make sure it's in a vertical plane parallel to the vertical plane of the screen, and that the object lies along the principal axis of the mirror. Or better yet get another mirror for testing.

 

[Drakkith]

Another experiment you can perform is to stand in a long hallway that has a glass doorway to the outside at one end. Hold the mirror such that you form an image of the doorway on a piece of paper held in a vertical plane parallel to the closed door. Now see if you can adjust the distance between the paper and the mirror such that distant objects located outside the doorway are in focus on the paper. The distance from the paper to the mirror is the focal length.

I 2nd this idea. Finding the focal length of the mirror is critical to this issue, as without it you can't use any equations properly. If you can't take the mirror out of the room, try to get a light source placed as far away from the mirror inside the room as possible. Assuming the focal length of the mirror is much smaller than the distance across the room, this will give you a fairly accurate measurement of the focal length. You might be able to use your cellphone screen as a light source if the room is totally dark. That should make it easy to place the source and the mirror far apart since a cell phone is small, lightweight, and doesn't require electrical connections.

 

[hutchphd]

If you look up this mirror Pasco 7573 concave I believe the diameter d=60cm is the size of the mirror (like for a telescope) and not the curvature.
Problem solved ?

 

[Mister T]

If you look up this mirror Pasco 7573 concave I believe the diameter d=60cm is the size of the mirror (like for a telescope) and not the curvature.
Problem solved ?

OP is looking for the focal length, which is independent of diameter.

 

[hutchphd]

The OP quoted 60cm as the focal length and then got very confusing. I have no idea what the issue is, but somebody is badly mistaken

 

[Albertgauss]

Sorry for the late reply, my email did not alert me there were any new responses.

Yes, I originally thought the focal length was 60 cm, but as I did the experiments above, I wasn't getting that focal length, at least, not according to the numbers and lens-maker's equation of this post. The test of where the image and object are at the center of curvature seem to yield a focal length closer to < 40 cm but the test of where the image and object transition from right-side-up to upside-down suggested a focal length of 60 cm. I was not able to complete the test of having the mirror down a long hallway because I don't have that, but I can do a dark room with my light bulb far away. I will have time tomorrow for that, so I will try this third test.

Yes, the diameter of the mirror is 60 cm. By coincidence, the focal length and diameter of the mirror were initially thought to be the same; I am aware that these are two different numbers. The test of the object a large distance from the mirror will reveal more information when I do that test.

 

[hutchphd]

I would measure the Sagitta of the mirror to calculate the radius of the sphere and thereby determine the focus. Sanity check. Then the rest should be pretty simple.

Clarification: when I say measure I mean get a straight edge and lay it across the mirror and measure the sagital depth with a ruler. Should be good to 1%

 

[Albertgauss]

I did the check where the object is much farther (~ 5 meters, 15 feet) from the mirror. The real image showed up at about 38 cm. Thus, it seems that the focal length really is about 38 cm and not the originally 60 cm I posted earlier (and my first test of flipping upside-down to right-side up was much more subjective). From the two tests of q=p and the long-distance test, the focal length is thus really ~ 38 cm.

You can ignore the Oct 5 posting I did now in light of this recent measurement; I didn't even know which image was the "real image" when I posted that, which was the main point of that post. Posting here sorted that out for me.

I will redo the measurement of Oct 7; in that post I erroneously had the focal length at 50 or 60 cm (in two different posts), which has since turned out to be wrong by the better techniques suggested in this post. For a focal length of 38 cm and an object at 75 cm, the image is predicted to appear at 77 cm by the lens maker equation, which is not so far off from the 90 cm in that post; nevertheless, I think I can measure this better having done this a bunch of times. I will attempt on Monday. This does feel like its converging; I just don't have access everyday to my measurements.