What happens when entangled photons are split into different paths?

[JamesPaylow]

TL;DR Summary

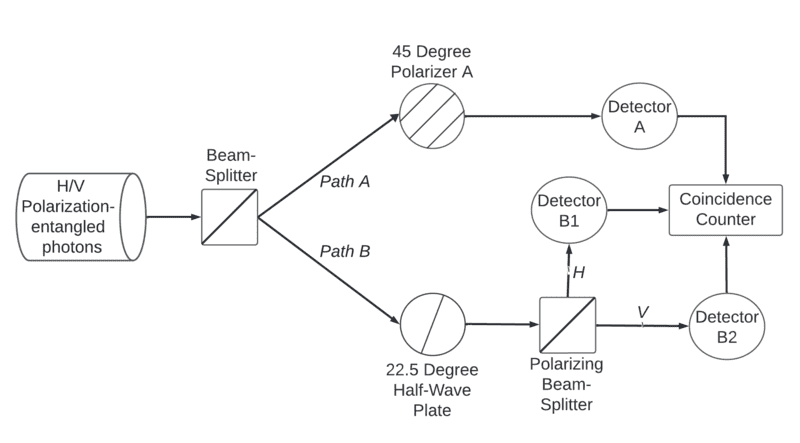
A hypothetical experimental setup is depicted, can you help me understand what would happen in the scenarios described here?

Please consider the depicted setup. We assume that the source is producing only H/V polarization entangled photon pairs (which is separately confirmed prior to the experiment).

Scenario 1) First suppose that the depicted 45 Degree Linear Polarizer A is absent. Photon B's polarization is in H/V superposition to start, then after going through the half wave plate oriented at 22.5 degrees -> (V)ertical polarization becomes (D)iagonal and (H)orizontal polarization becomes (A)ntidiagonal. The Polarizing Beam Splitter transmits V and reflects H. If A and D encounter the PBS they are each randomly converted and routed towards either the H or V path. Detectors B1 and B2 should register roughly the same number of photons after reducing to coincidences with Detector A.

Scenario 2) As depicted, the 45 Degree Linear Polarizer A is present. Photon A encounters the diagonally (45 degree) oriented linear polarizer in Path A. Since the photons in Path A and Path B are orthogonally polarized and entangled, when Photon A goes through the diagonal polarizer then we know that Photon B is (A)ntidiagonally polarized, and the particles are thereafter no longer entangled. If Photon B is A polarized when approaching the HWP oriented at 22.5 degrees, then it becomes (H)orizontally polarized. H polarization is reflected through the PBS. As a result, only Detector B1 registers impact (after filtering to coincides along Path A using the coincidence counter).

Have I described this correctly? If so, could the length of Path B change the outcome?

I'm familiar with the Quantum Eraser experiment and how in that experiment they showed that the length of the paths did not matter, but I think that post-selection filtering used to reduce the diffraction pattern to an interference pattern could possibly explain what seems to be retrocasual effect. Since the setup depicted here doesn't use interference patterns I think the same explanation would not apply.

@vanhees71 @gentzen an extension of my previous question :) Thanks in advance!

 

[DrClaude]

I don't think the figure is correct because you have pairs of photons, so they shouldn't go through a beam splitter. Path A and Path B should start independently from the source in opposite directions. But that doesn't really matter.

Let's write it out. The single-photon states are
$$
\ket{H},\quad \ket{V},\quad \ket{D} = \frac{1}{\sqrt{2}} \Big( \ket{H} + \ket{V} \Big),\quad \ket{A} = \frac{1}{\sqrt{2}} \Big( \ket{H} - \ket{V} \Big)
$$
The initial two-photon state is $\ket{\psi} = \frac{1}{\sqrt{2}} \Big( \ket{HV} - \ket{VH} \Big)$
The action of the HWP on single-photon states is
$$
\hat{U} \ket{V} = \ket{D}, \quad \hat{U} \ket{H} = \ket{A}
$$
therefore
$$
\begin{align*}
\hat{U} \ket{A} &= \hat{U} \frac{1}{\sqrt{2}} \Big( \ket{H} - \ket{V} \Big) \\
&= \frac{1}{\sqrt{2}} \Big(\hat{U}\ket{H} - \hat{U}\ket{V} \Big) \\
&= \frac{1}{\sqrt{2}} \Big(\ket{A} - \ket{D} \Big) \\
&= - \ket{V}
\end{align*}
$$
(so there is something wrong in what you wrote for scenario 2, but again it is not a big deal).

Scenario 1:
After the HWP, the two-photon state is
$$
\frac{1}{2} \Big( \ket{HH} + \ket{HV} - \ket{VH} + \ket{VV} \Big)
$$
That gives us equal probabilities for all cases, hence equal probabilities that detectors B1 and B2 will click (Detector A will click each time). So your result is correct.

For Scenario 2, lets divide it in two cases:

1st case: Path A is longer than path B

Photon B encounters the HWP before anything happens to photon A, so we have the same state as above,
$$
\frac{1}{2} \Big( \ket{HH} + \ket{HV} - \ket{VH} + \ket{VV} \Big)
$$
Photon A then reaches the polarizer. We are interested in the cases where it passes through it, resulting in a click in detector A. This corresponds to measuring photon A in state $\ket{D}$, so we have
$$
\begin{align*}
{}_A\bra{D} \frac{1}{2} \Big( \ket{HH} + \ket{HV} - \ket{VH} + \ket{VV} \Big) &\propto
\Big( {}_A\bra{H} + {}_A\bra{V} \Big) \Big( \ket{HH} + \ket{HV} - \ket{VH} + \ket{VV} \Big) \\
&= \Big( \ket{H}_B + \ket{V}_B - \ket{H}_B + \ket{V}_B \Big) \\
&= \ket{V}_B
\end{align*}
$$
Photon B is now in V polarization (not H as you write), so only detector B2 will click when detector A clicks.

2nd case: Path B is longer than path A

Photon A encounters the polarizer before anything happens to photon B, so we have a detection event similar to above, but applied to the initial two-photon state:
$$
\begin{align*}
{}_A\bra{D} \frac{1}{\sqrt{2}} \Big( \ket{HV} - \ket{VH} \Big) &\propto
\Big( {}_A\bra{H} + {}_A\bra{V} \Big) \Big( \ket{HV} - \ket{VH} \Big)\\
&= \Big(\ket{H}_B - \ket{V}_B \Big) \\
&\propto \ket{A}_B
\end{align*}
$$
Photon B now has polarization A. As shown above, the HWP will transform this polarization into V, so again only detector B2 will click when detector A clicks.

As you can see, the order of the operations have no influence on the result.

 

[vanhees71]

I'd rather write for the latter state (ignoring the normalization factors)
$$|D \rangle \langle D| \otimes \hat{1} (|HV-VH \rangle) \propto |D V \rangle-|D H \rangle \propto |DA \rangle,$$
because for the first photon going through the polarizer, i.e., for detector A clicking you have again a two-photon state after the first photon is going through the polarizer, and this is the right state, because you consider only when you have coincidence count of both photons.

Of course, this doesn't change your argument, which is correct, as far as I can see.

 

[JamesPaylow]

@DrClaude Wow thanks for all the great detail! I have a couple follow-up questions if you don't mind please. Both relate to the first case in scenario 2.

1. If I'm correctly following you, as a result of Photon A becoming |D>, Photon B becomes |H>, because Photon B has gone through the 22.5 degree HWP. I had thought, perhaps incorrectly, that since they were orthogonally polarized then it wouldn't matter that Photon B had gone through the HWP, Photon B would become |A> upon Photon A becoming |D>. But, that doesn't happen?

2. In the first case for scenario 2, with Path A being longer, the sequence of operations you've outlined still has Photon A encountering the polarizer prior to Photon B encountering the PBS. If Photon B encounters the PBS prior to Photon A encountering the polarizer, does the outcome change?

Many many thanks for your time and help here!

 

[DrChinese]

To be clear about path length: changing length will never change the quantum statistical prediction. The coincidence probability depends on the relationship between the A and B pairs, but does not depend in any way on time ordering or path length. Consequently, as @DrClaude stated, lengthening one path to make it appear that one photon is measured before the other does not change anything.

The effect you refer to as retrocausal is an artifact of trying to describe what happens as if one element of the setup is the cause of something else in the setup. To do that, you must rely on a particular interpretation of QM. Experimentally, you cannot distinguish cause from effect when asking: does measurement of A cause/change the outcome of B, or does measurement of B cause/change the outcome of A? These scenarios cannot be distinguished.

 

[JamesPaylow]

@DrClaude @DrChinese I would be very grateful if you were able to answer my two follow-up questions above. Thanks in advance!

 

[vanhees71]

@DrClaude Wow thanks for all the great detail! I have a couple follow-up questions if you don't mind please. Both relate to the first case in scenario 2.

1. If I'm correctly following you, as a result of Photon A becoming |D>, Photon B becomes |H>, because Photon B has gone through the 22.5 degree HWP. I had thought, perhaps incorrectly, that since they were orthogonally polarized then it wouldn't matter that Photon B had gone through the HWP, Photon B would become |A> upon Photon A becoming |D>. But, that doesn't happen?

2. In the first case for scenario 2, with Path A being longer, the sequence of operations you've outlined still has Photon A encountering the polarizer prior to Photon B encountering the PBS. If Photon B encounters the PBS prior to Photon A encountering the polarizer, does the outcome change?

Many many thanks for your time and help here!

The temporal order of the measurements is completely irrelevant. There's no causal influence of one measurement on the other photon. All there is are the correlations described by the entanglement of the photons, which are due to their preparation in that state before any manipulations are done with these photons.

 

[DrClaude]

@DrClaude Wow thanks for all the great detail! I have a couple follow-up questions if you don't mind please. Both relate to the first case in scenario 2.

1. If I'm correctly following you, as a result of Photon A becoming |D>, Photon B becomes |H>, because Photon B has gone through the 22.5 degree HWP. I had thought, perhaps incorrectly, that since they were orthogonally polarized then it wouldn't matter that Photon B had gone through the HWP, Photon B would become |A> upon Photon A becoming |D>. But, that doesn't happen?

No. It does matter that photon B has been through the HWP. I wrote above the two-photon state in terms of H and V for both photons, but we can also write it as
$$
\begin{align*}
\frac{1}{2} \Big( \ket{HH} + \ket{HV} - \ket{VH} + \ket{VV} \Big) &= \frac{1}{\sqrt{2}} \Big( \ket{HD} - \ket{VA} \Big) \\
&= \frac{1}{\sqrt{2}} \Big( \ket{AH} + \ket{DV} \Big)
\end{align*}
$$
So there is now a correlation between A and D for photon A and H and V for photon B.

2. In the first case for scenario 2, with Path A being longer, the sequence of operations you've outlined still has Photon A encountering the polarizer prior to Photon B encountering the PBS. If Photon B encounters the PBS prior to Photon A encountering the polarizer, does the outcome change?

No, it doesn't change. When you write it as $\frac{1}{\sqrt{2}} \Big( \ket{AH} + \ket{DV} \Big)$, you see that you get photon A in state A if detector B1 has clicked, and photon A in state D if detector B2 has clicked. Therefore, joint clicks will only happen between detector A and detector B2.

 

[JamesPaylow]

No. It does matter that photon B has been through the HWP. I wrote above the two-photon state in terms of H and V for both photons, but we can also write it as
$$
\begin{align*}
\frac{1}{2} \Big( \ket{HH} + \ket{HV} - \ket{VH} + \ket{VV} \Big) &= \frac{1}{\sqrt{2}} \Big( \ket{HD} - \ket{VA} \Big) \\
&= \frac{1}{\sqrt{2}} \Big( \ket{AH} + \ket{DV} \Big)
\end{align*}
$$
So there is now a correlation between A and D for photon A and H and V for photon B.No, it doesn't change. When you write it as $\frac{1}{\sqrt{2}} \Big( \ket{AH} + \ket{DV} \Big)$, you see that you get photon A in state A if detector B1 has clicked, and photon A in state D if detector B2 has clicked. Therefore, joint clicks will only happen between detector A and detector B2.

Sorry but I still have a question. What you are saying is that regardless of whether Photon A encounters the polarizer in Path A before or after Photon B encounters the HWP+PBS is irrelevant, and that what we see at detectors will always behave as if Path A polarizer impact happened first. Why is that? It seems arbitrary. Meaning, why would this setup always act as if Path A encounters happened first rather than Path B? Why not favor Path B? The Path A polarizer breaks entangled in the same way that the path B PBS does. Shouldn't which happens first matter?

 

[PeterDonis]

Shouldn't which happens first matter?

The short answer to this is that if the measurements commute, then no, which happens first does not matter.

In this case, the two measurements involved are on different photons, and measurements on disjoint degrees of freedom always commute. If it helps, you can think of the two measurement operators involved, acting on the joint Hilbert space of both photon polarizations, as being something like $M_A \otimes I_B$ and $I_A \otimes M_B$, where $M_A$ and $M_B$ are the measurements as you've described them, operating on the $A$ and $B$ photons respectively, and $I_A$ and $I_B$ are the identity operators on the photons that each measurement does not operate on. It is straightforward to verify that these operations commute.

 

[JamesPaylow]

The short answer to this is that if the measurements commute, then no, which happens first does not matter.

In this case, the two measurements involved are on different photons, and measurements on disjoint degrees of freedom always commute. If it helps, you can think of the two measurement operators involved, acting on the joint Hilbert space of both photon polarizations, as being something like $M_A \otimes I_B$ and $I_A \otimes M_B$, where $M_A$ and $M_B$ are the measurements as you've described them, operating on the $A$ and $B$ photons respectively, and $I_A$ and $I_B$ are the identity operators on the photons that each measurement does not operate on. It is straightforward to verify that these operations commute.

Thanks for your response. In this setup, as I'm understanding, the measurements on Photon A and Photon B are not on "disjoint degrees of freedom" in the strict sense because they are measurements on the same property (polarization) of two entangled particles. As such, the order of these measurements can theoretically lead to different outcomes.

 

[PeterDonis]

In this setup, as I'm understanding, the measurements on Photon A and Photon B are not on "disjoint degrees of freedom"

Yes, they are. The fact that the photons are entangled does not mean they are the same degrees of freedom. They aren't. They are disjoint degrees of freedom because, as my expressions for the measurements show, you can make a measurement that only acts non-trivially on one of the two photons--its action on the other is the identity, i.e., no change.

As such, the order of these measurements can theoretically lead to different outcomes.

No, it can't. That is the key point of the math that @DrClaude showed you earlier in the thread: his analysis is simply showing how the fact that the measurements commute works itself out in this particular case.

 

[JamesPaylow]

Sorry but I still have a question. What you are saying is that regardless of whether Photon A encounters the polarizer in Path A before or after Photon B encounters the HWP+PBS is irrelevant, and that what we see at detectors will always behave as if Path A polarizer impact happened first. Why is that? It seems arbitrary. Meaning, why would this setup always act as if Path A encounters happened first rather than Path B? Why not favor Path B? The Path A polarizer breaks entangled in the same way that the path B PBS does. Shouldn't which happens first matter?

@DrClaude If you can please. Thanks so much for your ongoing and very useful responses.

 

[DrClaude]

Sorry but I still have a question. What you are saying is that regardless of whether Photon A encounters the polarizer in Path A before or after Photon B encounters the HWP+PBS is irrelevant, and that what we see at detectors will always behave as if Path A polarizer impact happened first. Why is that?

@PeterDonis has answered that above.

It seems arbitrary. Meaning, why would this setup always act as if Path A encounters happened first rather than Path B? Why not favor Path B?

The thing is that the result is symmetric. It doesn't matter if A or B happens first. If we "favor path B," the same thing happens! I think this will be fundamental to your understanding: nothing that happens to photon A changes photon B, and vice versa. It is only when you compare the results of both photons (correlations) that any effect of entanglement can appear.

The Path A polarizer breaks entangled in the same way that the path B PBS does. Shouldn't which happens first matter?

Nope. It doesn't matter which photon is measured first. Note that this is essential for QM to be compatible with SR, has different observers will disagree as to which measurement occurred first.

 

[vanhees71]

What we discuss here are manipulations with single photons in an entangled photon pair. Here we are interested only in the polarization states of the photons. The polarization is described in some basis, e.g., the basis $|H \rangle$, $|V \rangle$. It refers ot photons that are linearly polarized in $x$ or $y$ direction (if the photon momentum is in $z$ direction). An arbitrary polarization state is then given as a superposition of these two states,
$$|\psi \rangle=c_1 |H \rangle + c_2 |V \rangle,$$
where $c_1$ and $c_2$ are complex numbers such that
$$\langle \psi|\psi \rangle=|c_1|^2+ |c_2|^2=1.$$
Their meaning is probabilistic, i.e., if you let the photon go through a polarization filter oriented in $x$-direction. This filter is described by the Operator
$$P_0=|H \rangle \langle H|,$$
and the probability that a photon goes through is
$$|\langle H|\psi \rangle|^2=|c_1|^2.$$

Now you have two photons. You have to give the polarization of both photons, and this is described by socalled product states. Using a basis for the polarization states of both photons, which are distinguished by their momenta, you have $|H \rangle \otimes |H \rangle$, $|V \rangle \otimes |H \rangle$, $|H \rangle \otimes V \rangle$, $|V \rangle \otimes |V \rangle$, which for convenience one also writes as $|H,H \rangl$, etc.

Now to describe a HWP on a single photon you have an operator, which acts on the basis like this
$$\hat{U}_{\text{HWP}}(0) |H \rangle=|H \rangle, \quad \hat{U}_{\text{HWP}} |V \rangle=-V \rangle.$$
Physically it's a uniaxial birefringent crystal cut such that its axis is parallel to two of its surfaces. Light falling in perpendicular to these surface that is polarized parallel to the optical axis (defining $|H \rangle$) has one index of refraction, one that's polarized perpendicular to it (defining $|V \rangle$) another. The length of the plate is chosen such that the phase difference for the polarization states exiting the crystal is $\pi$, which leads to the behavior on the polarization states described above.

For an arbitrary polarization state this implies that after the wave plate you get
$$\hat{U}_{\text{HWP}}(0)|\psi \rangle=c_1 |H \rangle -c_2 |V \rangle.$$
If you have a linear polarization state in an angle $\varphi$ to the $x$-axis, it's given by
$$|\psi(\varphi) \rangle=\cos(\varphi) |H \rangle + \sin \varphi |V \rangle.$$
Then you get
$$\hat{U}_{\text{HWP}}(0) |\psi \rangle =\cos(-\varphi) |H \rangle + \sin(-\varphi) |V \rangle,$$
i.e., your polarization direction is just mirrored at the $x$ axis. You can also describe it as rotating the polarization vector by an angle $\Theta=-2 \varphi$.
That's the same as rotating the HWP by an angle $\varphi$. So acting on $|H \rangle$ with the so oriented filter means to rotate it by an angle $=-2 \varphi$. For $\varphi=\pi/8$ the rotation is by $-\pi/4$. So you get
$$\hat{U}_{\text{HWP}}(\pi/8) |H \rangle=\cos(-\pi/4) |H |rangle + \sin(-\pi/4) |V \rangle=\frac{1}{\sqrt{2}}(|H \rangle-|V \rangle)=|A \rangle$$
and
$$\hat{U}_{\text{HWP}}(\pi/8) |V \rangle =\cos(\pi/2-\pi/4) |H \rangle + \sin(\pi/2-\pi/4) |V \rangle=\frac{1}{\sqrt{2}} (|H \rangle + |V \rangle)=|D \rangle,$$
as written in #2.

the $\pi/4$ polarizer is described by the projector, i.e., it's a filter
$$\hat{P}(\pi/4)=|D \rangle \langle D|$$
leading to
$$\hat{P}(\pi/4) |H \rangle=\hat{P}(\pi/4) |V \rangle=\frac{1}{2} |D \rangle.$$
So with the full setup as depicted in #1, after photon A went through the polarizer and photon B through the HWP you get the ket
$$|\psi' \rangle=\hat{U}_{\text{HWP}}(\pi/8) \otimes \hat{P}(\pi/4) \frac{1}{\sqrt{2}} (|HV \rangle-VH \rangle)=\frac{1}{2} \frac{1}{\sqrt{2}} (|DD \rangle-|D A \rangle)=\frac{1}{2 \sqrt{2}} |D \rangle \otimes(|D \rangle-|A \rangle)=\frac{1}{2 \sqrt{2}} D\rangle \otimes (2 V \rangle) = \frac{1}{\sqrt{2}} |DV \rangle.$$
That means if detector A clicks, then for sure B2 must also click (taking into account the action of the polarizing beam splitter too). This happens with a probabiility $\langle \psi'|\psi' \rangle=1/2$ (which is clear, because if a H- or V-polarized photon enters the polarizer it goes through with probability 1/2 and thus only with probability 1/2 detector A clicks).

Now the order of how photons A and B run through the polarizer and the HWP, respectively, doesn't matter, because the polarizer only acts on photon A and the HWP only on photon B. If you make the path between the source and the HWP of photon B much longer than the path for A from the source to the polarizer, you get the ket
$$(\hat{P} \otimes \hat{1}) (\hat{1} \otimes \hat{U}_{\text{HWP}}(\pi/8)) \frac{1}{\sqrt{2}} (|HV \rangle-VH \rangle) = \hat{P} \otimes \hat{U}_{\text{HWP}}(\pi/8)) \frac{1}{\sqrt{2}} (|HV \rangle-VH \rangle)=|\psi' \rangle.$$
If the path lengths are just the opposite it's
$$(\hat{1} \otimes \hat{U}_{\text{HWP}}(\pi/8))(\hat{P} \otimes \hat{1})\frac{1}{\sqrt{2}} (|HV \rangle-VH \rangle) = \hat{P} \otimes \hat{U}_{\text{HWP}}(\pi/8)) \frac{1}{\sqrt{2}} (|HV \rangle-VH \rangle)=|\psi' \rangle.$$
The order simply doesn't matter because the polarizer acts only on photon A and the HWP only on photon B. The corresponding operators thus commute, and there cannot be any causal influence by the polarizer on photon B nor any causal influence of the HWP on photon A. So the order the photon run through these optical elements doesn't matter.

 

[JamesPaylow]

@DrClaude @vanhees71 I really appreciate your continued engagement. Just to reiterate the Scenario 2 first case, in whidh Path A is longer, we should consider that both the HWP and PBS (in path B) are interacting before the polarizer (in path A). I worry that your initial responses are instead assuming HWP -> polarizer -> PBS, which is a different sequence.

With sequences as I describe here, as far as I can tell, the math actually supports the idea that interaction order does matter, because if the polarizer acts before either the HWP or PBS (Scenario 2, case 2) then Photon A becomes (D). This breaks entanglement and tells us that Photon B is (A). Since we know that Photon B is (A) before encountering the HWP then at the HWP Photon B becomes (H) (and doesn't do anything to Photon A since entanglement was broken), and then is reflected by the PBS. Result: only detection at Detector B1 (when considering coincidences with Detector A).

Alternatively, if we assume that the polarizer interaction occurs after both the HWP and PBS (Scenario 2, case 1) then Photon B was H/V superposition when approaching the HWP, gets converted to A/D superposition, and at the PBS is reflected or transmitted roughly 50% of the time while being converted to either H or V in the process. The PBS breaks entanglement. When Photon A subsequently encounters the polarizer it's in either H or V, either way gets through 50% of the time while being converted to D (which doesn't matter anymore for Photon B since entanglement has been broken). Result: Detection at Detectors both B1 and B2 (when considering coincidences with Detector A).

Results are different based on order of interaction.
@DrClaude to respond to your last chat, you wrote, "When you write it as $\frac{1}{\sqrt{2}}(|AH\rangle + |DV\rangle)$ you see that you get photon A in state A if detector B1 has clicked, and photon A in state D if detector B2 has clicked."

This seems to be a problematic step. The statement implies that the states $|AH\rangle$ and $|DV\rangle$ correspond to detections at B1 and B2, respectively. However, this is not necessarily the case when considering the action of the PBS on Photon B. Both the $|A\rangle$ and $|D\rangle$ states of Photon B have a 50% probability of leading to clicks at either B1 or B2 because of their superposition of $|H\rangle$ and $|V\rangle$.

The state $\frac{1}{\sqrt{2}}(|AH\rangle + |DV\rangle)$ does not inherently specify which detector (B1 or B2) will click. To determine that, you would need to further analyze the action of the PBS on the $|A\rangle$ and $|D\rangle$ states. Both states have a 50% chance of resulting in a click at either detector.

It seems that the conclusion that "joint clicks will only happen between detector A and detector B2" is not supported by the provided math. The math, in fact, seems to support the idea that both B1 and B2 have an equal chance of registering a photon when Photon A is measured in the $|D\rangle$ state. This is contrary to the conclusion drawn.

Greatly appreciate any clarity you all can provide. I'm genuinely trying to understand this better. For the purpose of the experiment I want to execute I actually want to be wrong about this (and want it to work the way you are describing), but need to understand it well enough to be confident. Thanks for your patience and assitance!

 

[PeterDonis]

Results are different based on order of interaction.

No, they are not. @DrClaude has already shown you the logic and the math in several posts. Your arguments about the two different results contradict what he said. I strongly suggest that you go through his post #2 in detail, step by step, matching up your steps in this post with his, until you see where you are going wrong.

The statement implies that the states $|AH\rangle$ and $|DV\rangle$ correspond to detections at B1 and B2, respectively.

Yes, that's because in those two states, photon B is in state $\ket{H}$ and $\ket{V}$ respectively, which correspond to those detections.

This is not necessarily the case when considering the action of the PBS on Photon B. Both the $|A\rangle$ and $|D\rangle$ states of Photon B

Are irrelevant to the states you mentioned, because photon B is not in those states. Photon A is. Photon A in state $\ket{A}$ in the state $\ket{AH}$, and photon A is in state $\ket{D}$ in the state $\ket{DV}$.

It seems that the conclusion that "joint clicks will only happen between detector A and detector B2" is not supported by the provided math.

Yes, it is. You're just reading the math wrong. See above.

 

[JamesPaylow]

@PeterDonis Please allow me to try using a couple different approaches to articulate my points better. First, with AI (I know this forum does not approve of using AI but I believe the text below can help clarify).

1. Forum Member's Claim: "No, they are not. @DrClaude has already shown you the logic and the math in several posts. Your arguments about the two different results contradict what he said. I strongly suggest that you go through his post #2 in detail, step by step, matching up your steps in this post with his, until you see where you are going wrong."
   Response: While @DrClaude provided mathematical representations, the interpretation of these representations in the context of the physical experiment is where there seems to be disagreement. Specifically, when considering the sequence where Photon B encounters the HWP and PBS before Photon A interacts with the polarizer, it is clear that Photon B will be in an ∣A⟩/∣D⟩ superposition when hitting the PBS. This leads to probabilistic outcomes at detectors B1 and B2.
2. Forum Member's Assertion: "Yes, that's because in those two states, photon B is in state ∣H⟩ and ∣V⟩ respectively, which correspond to those detections."
   Response: This is an accurate description of the states, but it doesn't necessarily determine the outcome at detectors B1 and B2. Specifically, when Photon B encounters the PBS before Photon A interacts with the polarizer, Photon B's state will be in a superposition of ∣A⟩ and ∣D⟩. Thus, it does not have a definitive polarization state of ∣H⟩ or ∣V⟩ when hitting the PBS.
3. Forum Member's Assertion: "Are irrelevant to the states you mentioned, because photon B is not in those states. Photon A is. Photon A is in state ∣H⟩ in the state ∣AH⟩, and photon A is in state ∣D⟩ in the state ∣DV⟩."
   Response: The forum member is correct in stating that the ∣AH⟩ and ∣DV⟩ states describe Photon A's polarization. However, the important point is what happens to Photon B based on the sequence of interactions. If Photon B encounters the PBS prior to Photon A hitting the polarizer, then Photon B's state will be in a superposition of ∣A⟩ and ∣D⟩ when it reaches the PBS. This leads to probabilistic outcomes at detectors B1 and B2.
4. Forum Member's Claim: "Yes, it is. You're just reading the math wrong. See above."
   Response: The math provided is a representation of the states, but the interpretation in terms of the sequence of interactions and subsequent outcomes at the detectors is where the disagreement lies. Given the sequence where Photon B encounters the HWP and PBS prior to Photon A interacting with the polarizer, it is clear that outcomes at detectors B1 and B2 will be different than if the polarizer acted first.

In essence, while the forum member has provided accurate descriptions of the states, the main point of contention is the interpretation of these states in terms of the sequence of interactions and the outcomes at the detectors. The sequence indeed matters, and different sequences lead to distinct outcomes at detectors B1 and B2.
Alternatively, let me try with math:

1. Polarizer Acts First:​

Given the initial entangled state:

$\ket{\psi} = \frac{1}{\sqrt{2}} \left( \ket{HV} - \ket{VH} \right)$
When Photon A encounters the 45° polarizer, the state collapses to:

$\ket{D}_A = \frac{1}{\sqrt{2}} \left( \ket{H} + \ket{V} \right)$
This results in Photon B being in the ∣A⟩ state:

$\ket{A}_B = \frac{1}{\sqrt{2}} \left( \ket{H} - \ket{V} \right)$
When Photon B then goes through the HWP, it transforms to:

$\hat{U} \ket{A}_B = \ket{H}_B$
Upon encountering the PBS, Photon B will be reflected and directed towards detector B1.

2. HWP + PBS Act First:​

Given the initial state:
$\ket{\psi} = \frac{1}{\sqrt{2}} \left( \ket{HV} - \ket{VH} \right)$

When Photon B encounters the HWP:
$\hat{U} \ket{H} = \ket{A}$
$\hat{U} \ket{V} = \ket{D}$

The state then evolves to:
$\ket{\psi'} = \frac{1}{\sqrt{2}} \left( \ket{HA} - \ket{VD} \right)$

Upon reaching the PBS, both ∣A⟩ and ∣D⟩ components of Photon B have an equal probability to be transmitted or reflected::

The component of Photon B in state ∣A⟩ has a 50% chance of being transmitted (leading to a B2 click) and a 50% chance of being reflected (leading to a B1 click).Similarly, the component of Photon B in state ∣D⟩ also has a 50% chance of being transmitted (leading to a B2 click) and a 50% chance of being reflected (leading to a B1 click).

$\ket{\psi''} = \frac{1}{2} \left( \ket{HA} + \ket{HV} - \ket{VD} - \ket{VH} \right)$

After Photon B's interaction with the PBS, the entanglement with Photon A is broken:

• Photon B can be in either ∣H⟩ or ∣V⟩ state.
• Photon A will correspondingly be in either ∣H⟩ or ∣V⟩ state due to their initial entangled state.

When Photon A later encounters the 45-degree polarizer:

• If Photon A is in the state ∣H⟩ (because Photon B was |V> after going through the PBS), it has a 50% chance of passing through the polarizer (converting to ∣D⟩) and a 50% chance of being blocked.

$\ket{H} \rightarrow \frac{1}{\sqrt{2}} \left( \ket{H} + \ket{V} \right) = \ket{D}$

• If Photon A is in the state ∣V⟩ (because Photon B was |H> after going through the PBS), it has a 50% chance of passing through the polarizer (converting to ∣D⟩) and a 50% chance of being blocked.

$\ket{V} \rightarrow \frac{1}{\sqrt{2}} \left( \ket{H} - \ket{V} \right) = \ket{D}$

In summary, for this scenario:

• If there's a click at B1, Photon A has a 50% chance of passing through the 45-degree polarizer (converting to ∣D⟩) and a 50% chance of being blocked by it.
• If there's a click at B2, Photon A also has a 50% chance of passing through the 45-degree polarizer (converting to ∣D⟩) and a 50% chance of being blocked by it.

In total, it still seems to me that order of interaction matters, and math and logic seem to support this conclusion. If the polarizer acts first we only get clicks at B1. If the HWP+PBS act first then we get clicks at both B1 and B2. Please help me see where I've made errors.

 

[DrClaude]

Alternatively, let me try with math:

1. Polarizer Acts First:​

When Photon B then goes through the HWP, it transforms to:

$\hat{U} \ket{A}_B = \ket{H}_B$
Upon encountering the PBS, Photon B will be reflected and directed towards detector B1.

Photon B actually acquires V polarization, so B2 will click.

2. HWP + PBS Act First:​

The state then evolves to:
$\ket{\psi'} = \frac{1}{\sqrt{2}} \left( \ket{HA} - \ket{VD} \right)$

Upon reaching the PBS, both ∣A⟩ and ∣D⟩ components of Photon B have an equal probability to be transmitted or reflected::

The component of Photon B in state ∣A⟩ has a 50% chance of being transmitted (leading to a B2 click) and a 50% chance of being reflected (leading to a B1 click).Similarly, the component of Photon B in state ∣D⟩ also has a 50% chance of being transmitted (leading to a B2 click) and a 50% chance of being reflected (leading to a B1 click).

$\ket{\psi''} = \frac{1}{2} \left( \ket{HA} + \ket{HV} - \ket{VD} - \ket{VH} \right)$

This is incorrect. After the HWP, you are correct in saying that the state is
$$
\ket{\psi'} = \frac{1}{\sqrt{2}} \Big( \ket{HA} - \ket{VD} \Big)
$$
However, the PBS does not change the state. It only associates $\ket{H}$ and $\ket{V}$ for photon B with different paths in space (which will ultimately correspond to different detectors clicking). To make this explicit, we can first rewrite the state for photon B in the H/V basis (again, same state, only a different way of writing it)
$$
\begin{align*}
\ket{\psi'} &= \frac{1}{\sqrt{2}} \Big( \ket{HA} - \ket{VD} \Big) \\
&= \frac{1}{2} \Big( \ket{HH} - \ket{HV} - \ket{VH} - \ket{VV} \Big) \\
&= \frac{1}{2} \Big( \ket{HH} - \ket{VH} - \ket{HV} - \ket{VV} \Big)
\end{align*}
$$
Now lets rewrite photon A in the A/D basis (again, same state, only a different way of writing it)
$$
\begin{align*}
\ket{\psi'} &= \frac{1}{2} \Big( \ket{HH} - \ket{VH} - \ket{HV} - \ket{VV} \Big) \\
&= \frac{1}{\sqrt{2}} \Big( \ket{AH} - \ket{DV} \Big) \\
\end{align*}
$$
So with probability 1/2, photon B is found in state $\ket{H}$, detector B1 will click, and photon A will be left in state $\ket{A}$, will thus not pass the polarizer and not lead to a click of detector A. With probability 1/2, photon B is found in state $\ket{V}$, detector B2 will click, and photon A will be left in state $\ket{D}$, will pass the polarizer and detector A will click. Correlated click will only be found between detector A and detector B2, just as in the case 1, where the polarizer acts first.

After Photon B's interaction with the PBS, the entanglement with Photon A is broken:

• Photon B can be in either ∣H⟩ or ∣V⟩ state.
• Photon A will correspondingly be in either ∣H⟩ or ∣V⟩ state due to their initial entangled state.

This is incorrect and I think the crux of your misunderstanding. In the initial state, photon A in state H/V requires photon B to be in state V/H (100% anti-correlation). Likewise, if one were to measure using the D/A basis, in the initial state, photon A in state D/A requires photon B to be in state A/D (100% anti-correlation). However, the HWP changes this, as it replaces V/H for photon B with A/D. Hence measuring photon B in either states $\ket{H}$ or $\ket{V}$ doesn't mean we will find photon A in the opposite H/V state. The particles are still entangled, but do not show 100% anti-correlation on measurements using the same polarization axis.

 

[PeterDonis]

First, with AI (I know this forum does not approve of using AI but I believe the text below can help clarify).

Whether you think it can "help clarify" is irrelevant. AI-generated content is off limits here. If you have something to say, you need to say it, not ask an AI to say it for you.

Alternatively, let me try with math

Which, as @DrClaude has shown you, is wrong. Again, I strongly, strongly suggest that you stop posting until you have gone through the logic step by step, comparing yours with @DrClaude's, to see where you are going wrong.

 

[tistemfnp]

With sequences as I describe here, as far as I can tell, the math actually supports the idea that interaction order does matter,

In my world, math does not contradict physics .

Result: only detection at Detector B1 (when considering coincidences with Detector A).

correct (or the other one, but only one of them all the time - too lazy to check which one)

Alternatively, if we assume that the polarizer interaction occurs after both the HWP and PBS (Scenario 2, case 1) then Photon B was H/V superposition when approaching the HWP, gets converted to A/D superposition, and at the PBS is reflected or transmitted roughly 50% of the time while being converted to either H or V in the process. The PBS breaks entanglement. When Photon A subsequently encounters the polarizer it's in either H or V, either way gets through 50% of the time while being converted to D (which doesn't matter anymore for Photon B since entanglement has been broken). Result: Detection at Detectors both B1 and B2 (when considering coincidences with Detector A).

This really hurts... H/V =::= A/D when you talk about a super position state. Advice: basic QM class, beginner level. The only difference is the chosen basis. There is nothing "converted" by an HWP in an H/V superposition state. You can choose whatever basis. If you like you can rotate the basis by 5° and call the eigenstates fixy and foxy --> H/V =::== A/D =::= fixy/foxy. The HWP in this scenario is doing: n o t h i n g.

So same result no matter what is the path length of B.

 

[vanhees71]

@PeterDonis Please allow me to try using a couple different approaches to articulate my points better. First, with AI (I know this forum does not approve of using AI but I believe the text below can help clarify).

1. Forum Member's Claim: "No, they are not. @DrClaude has already shown you the logic and the math in several posts. Your arguments about the two different results contradict what he said. I strongly suggest that you go through his post #2 in detail, step by step, matching up your steps in this post with his, until you see where you are going wrong."
   Response: While @DrClaude provided mathematical representations, the interpretation of these representations in the context of the physical experiment is where there seems to be disagreement. Specifically, when considering the sequence where Photon B encounters the HWP and PBS before Photon A interacts with the polarizer, it is clear that Photon B will be in an ∣A⟩/∣D⟩ superposition when hitting the PBS. This leads to probabilistic outcomes at detectors B1 and B2.
2. Forum Member's Assertion: "Yes, that's because in those two states, photon B is in state ∣H⟩ and ∣V⟩ respectively, which correspond to those detections."
   Response: This is an accurate description of the states, but it doesn't necessarily determine the outcome at detectors B1 and B2. Specifically, when Photon B encounters the PBS before Photon A interacts with the polarizer, Photon B's state will be in a superposition of ∣A⟩ and ∣D⟩. Thus, it does not have a definitive polarization state of ∣H⟩ or ∣V⟩ when hitting the PBS.
3. Forum Member's Assertion: "Are irrelevant to the states you mentioned, because photon B is not in those states. Photon A is. Photon A is in state ∣H⟩ in the state ∣AH⟩, and photon A is in state ∣D⟩ in the state ∣DV⟩."
   Response: The forum member is correct in stating that the ∣AH⟩ and ∣DV⟩ states describe Photon A's polarization. However, the important point is what happens to Photon B based on the sequence of interactions. If Photon B encounters the PBS prior to Photon A hitting the polarizer, then Photon B's state will be in a superposition of ∣A⟩ and ∣D⟩ when it reaches the PBS. This leads to probabilistic outcomes at detectors B1 and B2.
4. Forum Member's Claim: "Yes, it is. You're just reading the math wrong. See above."
   Response: The math provided is a representation of the states, but the interpretation in terms of the sequence of interactions and subsequent outcomes at the detectors is where the disagreement lies. Given the sequence where Photon B encounters the HWP and PBS prior to Photon A interacting with the polarizer, it is clear that outcomes at detectors B1 and B2 will be different than if the polarizer acted first.

In essence, while the forum member has provided accurate descriptions of the states, the main point of contention is the interpretation of these states in terms of the sequence of interactions and the outcomes at the detectors. The sequence indeed matters, and different sequences lead to distinct outcomes at detectors B1 and B2.
Alternatively, let me try with math:

1. Polarizer Acts First:​

Given the initial entangled state:

$\ket{\psi} = \frac{1}{\sqrt{2}} \left( \ket{HV} - \ket{VH} \right)$
When Photon A encounters the 45° polarizer, the state collapses to:

$\ket{D}_A = \frac{1}{\sqrt{2}} \left( \ket{H} + \ket{V} \right)$
This results in Photon B being in the ∣A⟩ state:

$\ket{A}_B = \frac{1}{\sqrt{2}} \left( \ket{H} - \ket{V} \right)$
When Photon B then goes through the HWP, it transforms to:

$\hat{U} \ket{A}_B = \ket{H}_B$
Upon encountering the PBS, Photon B will be reflected and directed towards detector B1.

2. HWP + PBS Act First:​

Given the initial state:
$\ket{\psi} = \frac{1}{\sqrt{2}} \left( \ket{HV} - \ket{VH} \right)$

When Photon B encounters the HWP:
$\hat{U} \ket{H} = \ket{A}$
$\hat{U} \ket{V} = \ket{D}$

The state then evolves to:
$\ket{\psi'} = \frac{1}{\sqrt{2}} \left( \ket{HA} - \ket{VD} \right)$

Upon reaching the PBS, both ∣A⟩ and ∣D⟩ components of Photon B have an equal probability to be transmitted or reflected::

The component of Photon B in state ∣A⟩ has a 50% chance of being transmitted (leading to a B2 click) and a 50% chance of being reflected (leading to a B1 click).Similarly, the component of Photon B in state ∣D⟩ also has a 50% chance of being transmitted (leading to a B2 click) and a 50% chance of being reflected (leading to a B1 click).

$\ket{\psi''} = \frac{1}{2} \left( \ket{HA} + \ket{HV} - \ket{VD} - \ket{VH} \right)$

After Photon B's interaction with the PBS, the entanglement with Photon A is broken:

• Photon B can be in either ∣H⟩ or ∣V⟩ state.
• Photon A will correspondingly be in either ∣H⟩ or ∣V⟩ state due to their initial entangled state.

When Photon A later encounters the 45-degree polarizer:

• If Photon A is in the state ∣H⟩ (because Photon B was |V> after going through the PBS), it has a 50% chance of passing through the polarizer (converting to ∣D⟩) and a 50% chance of being blocked.

$\ket{H} \rightarrow \frac{1}{\sqrt{2}} \left( \ket{H} + \ket{V} \right) = \ket{D}$

• If Photon A is in the state ∣V⟩ (because Photon B was |H> after going through the PBS), it has a 50% chance of passing through the polarizer (converting to ∣D⟩) and a 50% chance of being blocked.

$\ket{V} \rightarrow \frac{1}{\sqrt{2}} \left( \ket{H} - \ket{V} \right) = \ket{D}$

In summary, for this scenario:

• If there's a click at B1, Photon A has a 50% chance of passing through the 45-degree polarizer (converting to ∣D⟩) and a 50% chance of being blocked by it.
• If there's a click at B2, Photon A also has a 50% chance of passing through the 45-degree polarizer (converting to ∣D⟩) and a 50% chance of being blocked by it.

In total, it still seems to me that order of interaction matters, and math and logic seem to support this conclusion. If the polarizer acts first we only get clicks at B1. If the HWP+PBS act first then we get clicks at both B1 and B2. Please help me see where I've made errors.

I've shown the math with the two photons. That should make it clear. The order doesn't play a role, because the operators used to discribe how the two-photon states change due to the optical elements used commute!

 

[vanhees71]

In my world, math does not contradict physics .correct (or the other one, but only one of them all the time - too lazy to check which one)

This really hurts... H/V =::= A/D when you talk about a super position state. Advice: basic QM class, beginner level. The only difference is the chosen basis. There is nothing "converted" by an HWP in an H/V superposition state. You can choose whatever basis. If you like you can rotate the basis by 5° and call the eigenstates fixy and foxy --> H/V =::== A/D =::= fixy/foxy. The HWP in this scenario is doing: n o t h i n g.

So same result no matter what is the path length of B.

Of course the HWP changes the states. It's discribed by a unitary operator as given by @DrClaude already in #2, and I tried to explain, how this operator is derived from the physics (birefringent crystal) in #15, where I then applied this formalism to the two-photon states.

 

[PeterDonis]

Thread closed for moderation.

 

[PeterDonis]

After some cleanup, the thread is reopened.

 

[PeterDonis]

H/V =::= A/D when you talk about a super position state.

If the entangled two-photon state is the singlet state, then yes, it looks the same in any basis. The entangled two-photon state produced by the source in this experiment is the singlet state, yes. But after the HWP acts, the entangled two-photon state is no longer the singlet state, because the HWP only acts on one photon, not both. So after the HWP acts, the state no longer looks the same in any basis.

 

[JamesPaylow]

However, the PBS does not change the state. It only associates $\ket{H}$ and $\ket{V}$ for photon B with different paths in space (which will ultimately correspond to different detectors clicking). To make this explicit, we can first rewrite the state for photon B in the H/V basis (again, same state, only a different way of writing it)
$$
\begin{align*}
\ket{\psi'} &= \frac{1}{\sqrt{2}} \Big( \ket{HA} - \ket{VD} \Big) \\
&= \frac{1}{2} \Big( \ket{HH} - \ket{HV} - \ket{VH} - \ket{VV} \Big) \\
&= \frac{1}{2} \Big( \ket{HH} - \ket{VH} - \ket{HV} - \ket{VV} \Big)
\end{align*}
$$
Now lets rewrite photon A in the A/D basis (again, same state, only a different way of writing it)
$$
\begin{align*}
\ket{\psi'} &= \frac{1}{2} \Big( \ket{HH} - \ket{VH} - \ket{HV} - \ket{VV} \Big) \\
&= \frac{1}{\sqrt{2}} \Big( \ket{AH} - \ket{DV} \Big) \\
\end{align*}
$$
So with probability 1/2, photon B is found in state $\ket{H}$, detector B1 will click, and photon A will be left in state $\ket{A}$, will thus not pass the polarizer and not lead to a click of detector A. With probability 1/2, photon B is found in state $\ket{V}$, detector B2 will click, and photon A will be left in state $\ket{D}$, will pass the polarizer and detector A will click. Correlated click will only be found between detector A and detector B2, just as in the case 1, where the polarizer acts first.This is incorrect and I think the crux of your misunderstanding. In the initial state, photon A in state H/V requires photon B to be in state V/H (100% anti-correlation). Likewise, if one were to measure using the D/A basis, in the initial state, photon A in state D/A requires photon B to be in state A/D (100% anti-correlation). However, the HWP changes this, as it replaces V/H for photon B with A/D. Hence measuring photon B in either states $\ket{H}$ or $\ket{V}$ doesn't mean we will find photon A in the opposite H/V state. The particles are still entangled, but do not show 100% anti-correlation on measurements using the same polarization axis.

@DrClaude my sincere gratitude for continuing to respond, and in a friendly manner. I hear your point that the PBS does not change the state. I'm still struggling to understand the *why* of the outcome you're describing though. Specifically in the case where the polarizer acts after both the HWP and PBS, and let's say also add after interacting with detectors B1/B2. The way I understand you point, you're saying that since interaction with the polarizer is the thing that finally defines the polarization state, when that happens the entire system will behave as if the entangled photons started in that final state. In other words, it doesn't matter when sequentially Photon A encounters the polarizer, in order to get past the polarizer it will become (D) and as a result Photon B will behave as if it started at as (A), subsequently being converted to V at the PBS and being routed to Detector B2. Correct?

I'm stuck on the idea that sequence can matter, though I know basically everyone here tells me it can't, I'm really trying to better understand why it cannot. If I've accurately captured the description above, my core question is why the polarizer is the only thing in this setup that can determine the final state. You've made it clear that the PBS does not do so as I initially thought. How about if I insert distinctly oriented polarizers, suppose D and A, immediately in front of Detectors B1 and B2? Then photons coming out of the PBS will have a determined state. And assume still that interaction with Detectors B1/B2 happens sequentially before that with the polarizer in Path A.

So in this modified example it would look like the attached. Photon B starts as H/V, encounters the HWP and becomes A/D, is routed 50% towards Detectors B1/B2 (so B1 has 25% of overall Photon B photons and those are D, and another 25% of total that are A --- B2 has the same), and then encounters a linear filter associated oriented in association with their respective routing, which makes Photon B either (D) or (A) before hitting the detector. Would you similarly assert in this example that regardless of whether Detectors B1 and B2 register prior to any interaction with polarizer A we will always only see coincidences at one of the B detectors? If so, can you help me understand why? Because it seems by Photon B ultimately becoming A or D (as forced by Pol B1 and Pol B2), if we account for the action of the HWP, we arrive at it having to have been H or V prior to encountering the HWP. If Photon B was H or V, then Photon A similarly was either H or V (the opposite of Photon B). Now, when Photon A encounters Polarizer A, regardless of whether it's H or V, 50% gets through and it becomes D. In terms of Photon B, all Polarizer A is ultimately doing is reducing the coincidence counts at Detectors B1 and B2 by roughly 50% each.

Written descriptions are easier for me to understand than math btw. Thank you

 

[DrChinese]

So in this modified example it would look like the attached.

1. Photon B starts as H/V, encounters the HWP and becomes A/D...2. ... is routed 50% towards Detectors B1/B2 (so B1 has 25% of overall Photon B photons and those are D, and another 25% of total that are A --- B2 has the same)...

3. Would you similarly assert in this example that regardless of whether Detectors B1 and B2 register prior to any interaction with polarizer A we will always only see coincidences at one of the B detectors?

1. Photon B (from stream B) starts in a superposition of H/V, true, but H and V are arbitrary labels. There is no detectable difference in B stream photons before or after the wave plate. H/V and A/D are equally valid labels at this point.

Yes, there is a change of state as has pointed out by @vanhees71 , @DrClaude and @PeterDonis. And this change of state can be objectively revealed in coincidence with the A stream. Specifically, the A and B streams are now offset 45 degrees relative to each other.

Note: I am assuming that the intended effect of your adding the wave plate is to create such an effect (rotation). If not, please correct me. I would usually expect that wave plates might be used instead to switch from one Bell State to another of the 4 states.

2. I agree with this - sorta. An important point though: After the PBS, the B stream is no longer entangled with the A stream. The outputs of the PBS are streams of V and H polarized photons, no longer in a superposition. So the outputs of the B1 and B2 polarizers are simply random (i.e. unentangled) photon streams polarized A (if from B1) or D (if from B2). Note that the intensity is a function of the theta between the PBS and the respective B1 or B2 polarizers. Because that is 45 degrees, the intensity is 50% per the cos^2 rule.

3. Yes, just at one (although I admit I get confused as to which)! The math works the same regardless of whether you examine from A's perspective or from B's. Let's examine starting from A's.

a. Any A photon hitting detector A is polarized D (diagonal), right? So the B partner will act as if it is A (antidiagonal). Since they are entangled in an anti-correlated manner.
b. Then it is rotated by 45 degrees at the wave plate (as before, I am assuming you are intending a rotation of this type). So now it is H (unless I have reversed them, but let's assume H is correct). All of the B stream partners for those A stream antidiagonals will go to the H fork out of the PBS.
c. The other half would go to the V fork, and they match with the A stream antidiagonals. None of those will pass to the A detector (because they are filtered out).

You can analyze it as I have from B's perspective and you will get the same result. Either perspective occurring first will get the same answer. So obviously order does not matter.

 

[vanhees71]

1. Photon B (from stream B) starts in a superposition of H/V, true, but H and V are arbitrary labels. There is no detectable difference in B stream photons before or after the wave plate. H/V and A/D are equally valid labels at this point.

Yes, there is a change of state as has pointed out by @vanhees71 , @DrClaude and @PeterDonis. And this change of state can be objectively revealed in coincidence with the A stream. Specifically, the A and B streams are now offset 45 degrees relative to each other.

Note: I am assuming that the intended effect of your adding the wave plate is to create such an effect (rotation). If not, please correct me. I would usually expect that wave plates might be used instead to switch from one Bell State to another of the 4 states.

Of course that's correct.

2. I agree with this - sorta. An important point though: After the PBS, the B stream is no longer entangled with the A stream. The outputs of the PBS are streams of V and H polarized photons, no longer in a superposition. So the outputs of the B1 and B2 polarizers are simply random (i.e. unentangled) photon streams polarized A (if from B1) or D (if from B2). Note that the intensity is a function of the theta between the PBS and the respective B1 or B2 polarizers. Because that is 45 degrees, the intensity is 50% per the cos^2 rule.

After the PBS, which is a unitary operation too (in the idealized sense of course), the two photons are still entangled. Only after detection they are no longer entangled. Then, of course, the detected photon is absorped by the detector, and you have a single-photon state left.

3. Yes, just at one (although I admit I get confused as to which)! The math works the same regardless of whether you examine from A's perspective or from B's. Let's examine starting from A's.

a. Any A photon hitting detector A is polarized D (diagonal), right? So the B partner will act as if it is A (antidiagonal). Since they are entangled in an anti-correlated manner.
b. Then it is rotated by 45 degrees at the wave plate (as before, I am assuming you are intending a rotation of this type). So now it is H (unless I have reversed them, but let's assume H is correct). All of the B stream partners for those A stream antidiagonals will go to the H fork out of the PBS.
c. The other half would go to the V fork, and they match with the A stream antidiagonals. None of those will pass to the A detector (because they are filtered out).

You can analyze it as I have from B's perspective and you will get the same result. Either perspective occurring first will get the same answer. So obviously order does not matter.

That's correct too.

 

[JamesPaylow]

1. Photon B (from stream B) starts in a superposition of H/V, true, but H and V are arbitrary labels. There is no detectable difference in B stream photons before or after the wave plate. H/V and A/D are equally valid labels at this point.

This may not be a material point, but my expectation is that we start with an H/V superposition explicitly, not A/D. I believe I have a method of creating entangled pairs in this way, and have come up with a way of validating that prior to the experiment. It's all beyond the scope of this question though, so if we can let's just assume that the entangled photons are generated such that we know one is H and the other is V (though don't know which is which).

2. After the PBS, the B stream is no longer entangled with the A stream. The outputs of the PBS are streams of V and H polarized photons, no longer in a superposition.

Seems to be some disagreement on whether the PBS breaks entanglement or not, but let's just assume the detectors also interact before the polarizer in Path A. So if the PBS doesn't break entanglement the detectors at least will.

3. Yes, just at one (although I admit I get confused as to which)! The math works the same regardless of whether you examine from A's perspective or from B's. Let's examine starting from A's.

a. Any A photon hitting detector A is polarized D (diagonal), right? So the B partner will act as if it is A (antidiagonal). Since they are entangled in an anti-correlated manner.
b. Then it is rotated by 45 degrees at the wave plate (as before, I am assuming you are intending a rotation of this type). So now it is H (unless I have reversed them, but let's assume H is correct). All of the B stream partners for those A stream antidiagonals will go to the H fork out of the PBS.
c. The other half would go to the V fork, and they match with the A stream antidiagonals. None of those will pass to the A detector (because they are filtered out).

You can analyze it as I have from B's perspective and you will get the same result. Either perspective occurring first will get the same answer. So obviously order does not matter.

It doesn't seem to give the same result if we analyze from B's perspective though; I suppose that's what I'm struggling with. Photon B starts as H/V, encounters the HWP and becomes A/D, is routed 50% towards Detectors B1/B2. So B1 registers half A and half D, B2 also registers half A and half D - that is, of course, unless we add a 45/-45 degree filter directly in front of each detector, as included in my previous diagram, but let's ignore that filter for now. So basically by evaluating a given Photon B that has reached either Detector B1 or B2 we can't say whether it started as H or V, because either state could have led to detection at either B detector. But, now that it's been detected at Detectors B1 or B2 the polarization state is defined; it's no longer in superposition. But, if Photon B started as H or V, as we expected, then Photon A was orthogonality polarized as either H or V. Regardless of H or V it will pass through Polarizer A 50% of the time. Since 50% of Photon A volume gets blocked at Polarizer A we are effectively ignoring 50% of Photon B volume as well, but since these are evenly distributed across both Detectors B1 and B2 they both see an overall reduction of 50%, but still both deteectors register roughly the same number of coincidences with Detector A.

 

[vanhees71]

Seems to be some disagreement on whether the PBS breaks entanglement or not, but let's just assume the detectors also interact before the polarizer in Path A. So if the PBS doesn't break entanglement the detectors at least will.

You can check this for yourself. You just need to think about what the PBS does. It's (thinking about an idealized lossless device again) another unitary transformation, when acting on the polarization states. What it does is simply to entangle the polarization states H/V to the photon momentum: One state goes through the other is reflected to 90 degrees, i.e., you have
$$\hat{U}_{\text{PBS}} |H, \vec{p}_{\text{in}} \rangle = |H,\vec{p}_{\text{refl}} \rangle,$$ $$\hat{U}_{\text{PBS}} |V, \vec{p}_{\text{in}} \rangle = |V,\vec{p}_{\text{in}} \rangle.$$
So the two-photon state when one photon went through the polarizer and the other through the HWP and the PBS is $-|D \rangle \otimes |V,\vec{p}_{\text{in}}$ (which is not an entangled state, because it's a product state). Since in the direction $\vec{p}_{\text{in}}$ if Detector A detects the its photon then for sure Detector B2 clicks.

 

[DrChinese]

1. This may not be a material point, but my expectation is that we start with an H/V superposition explicitly, not A/D. I believe I have a method of creating entangled pairs in this way, and have come up with a way of validating that prior to the experiment. It's all beyond the scope of this question though, so if we can let's just assume that the entangled photons are generated such that we know one is H and the other is V (though don't know which is which).

2. Seems to be some disagreement on whether the PBS breaks entanglement or not, but let's just assume the detectors also interact before the polarizer in Path A. So if the PBS doesn't break entanglement the detectors at least will.

3. It doesn't seem to give the same result if we analyze from B's perspective though; I suppose that's what I'm struggling with. Photon B starts as H/V, encounters the HWP and becomes A/D, is routed 50% towards Detectors B1/B2. So B1 registers half A and half D, B2 also registers half A and half D...

4. So basically by evaluating a given Photon B that has reached either Detector B1 or B2 we can't say whether it started as H or V, because either state could have led to detection at either B detector. But, now that it's been detected at Detectors B1 or B2 the polarization state is defined; it's no longer in superposition. But, if Photon B started as H or V, as we expected, ...

1. There is no such thing as creating 2 photon polarization entanglement on the H/V basis, but not polarization entanglement on the A/D basis. The H/V designation in these cases is arbitrary. An entangled photon pair is in a superposition of all polarizations, and will yield perfect anti-correlations (or correlations depending on PDC type) at *all* angles. For your analysis, this poses a problem.

2. There is no disagreement between @vanhees71 and I on this point. He is technically correct (as he usually is) that entanglement does not stop at the PBS. We know that because it is possible to reverse that operation and restore the entanglement as long as it is done before the detectors. But for analysis of your setup, that point does not matter. After the PBS, for all practical purposes there is no polarization entanglement remaining between photon A and photon B. Note again that the timing and ordering of which occurs first - A's polarizer or B's PBS - does not matter.

3. This analysis is acceptable so far. Keep in mind, however, that the B stream A/D pairing now maps its entanglement to the A stream H/V pairing. Which also means: B stream H/V pairing now maps its entanglement to the A stream A/D pairing. This is critical to understand for the next step. And this is why your assumption is a problem.

4. When the rotated B stream H/V basis arrives at the H/V PBS, the matching A stream entanglement is on the A/D basis. So when those A photons pass the D oriented filter, they are being matched only to the B stream that goes one way at the PBS. Again, here we are examining from the perspective that the B stream is the controlling perspective (as if ordering mattered). So the PBS is serving to "cause" collapse of the A stream into its A/D entanglement.

Again, in this analysis (as in the analysis from A's perspective from my prior post): The B stream started life "as if" it was A/D polarized (of course it was really in a superposition of all polarization states). Naturally that means the A stream started life "as if" it was similarly polarized.

----------

So we conclude: your invalid assumption that the entangled photon pair is entangled at a preferred angle (H/V) - but not otherwise - cannot be used to yield a correct analysis of a quantum mechanical setup. If you use it anyway, you will come to a conclusion that is at odds with what actually happens, i.e. that somehow ordering matters (it doesn't per theory and experiment).

 

[Nugatory]

This may not be a material point, but my expectation is that we start with an H/V superposition explicitly, not A/D. I believe I have a method of creating entangled pairs in this way, and have come up with a way of validating that prior to the experiment.

You have made some mistake here, as the A/D superposition and the H/V superposition are the same state, just written as the sum of two vectors in different ways. I can write 12=7+5 and 12=8+4, but it does not follow that there are two kinds of cartons of a dozen eggs, the 7+5 kind and the 8+4 kind. Likewise $|A\rangle+|D\rangle$ and $|H\rangle+|V\rangle$ aren't different states, just the same state written as the sum of two vectors in different ways.

 

[PeterDonis]

the A/D superposition and the H/V superposition are the same state

To be clear: the states (ignoring normalization factors) $\ket{AD} - \ket{DA}$ and $\ket{HV} - \ket{VH}$ are the same entangled state (the "singlet" state), expressed in two different bases. This is the initial state in the experiment. ("Entangled" is a better term than "superposition" because, as I think I've already pointed out in this thread, the latter is basis dependent but the former is not.)

But after the HWP acts, the state is a different entangled state, $\ket{AH}+ \ket{DV}$ (again ignoring the normalization factor).

 

[PeterDonis]

$|A\rangle+|D\rangle$ and $|H\rangle+|V\rangle$ aren't different states

Yes, they are. $\ket{A} + \ket{D}$ is just $\ket{H}$ (up to normalization). Which is obviously a different state from $\ket{H}$ + $\ket{V}$, which is $\ket{D}$ (again up to normalization). See the formulas @DrClaude gave in post #2.