Inertia tensor around principal Axes part 2

[Lambda96]

Homework Statement

I need to calculate the inertia tensor again around a principal axes showing the following $I_{23}=0$ and $I_{22}=I_{33}$.

Relevant Equations

none

Hi,

it's about the task e)

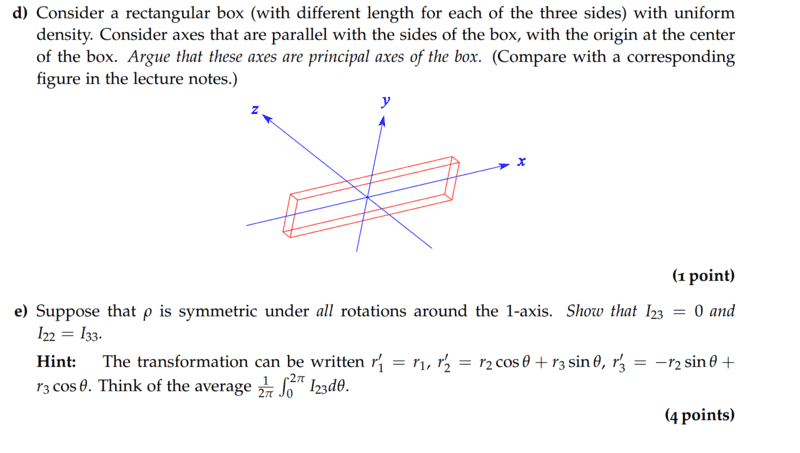
Since the density is homogeneous, I have assumed the following for $\rho=\frac{M}{V}$.

I then started the proof of $I_{23}$, the integral looks like this:

$$ I_{23}=\int_{}^{} -\frac{M}{V}r'_2r'_3 d^3r$$

Now I apply the transformation

$$ I_{23}=\int_{}^{} -\frac{M}{V}\Bigl(r_2cos\theta+r_3sin\theta)\Bigr)\cdot \Bigl(-r_2sin\theta + r_3cos\theta \Bigr) \ d^3r$$
$$ I_{23}=\int_{}^{} -\frac{M}{V}\Bigl(cos\theta sin\theta(r_3^2-r_2^2)+r_2r_3cos{2\theta})\Bigr) \ d^3r$$

Now I just used the clue, so $\frac{1}{2\pi} \int_{0}^{2\pi} I_{23} d\theta$

$$I_{23}=\int_{}^{} \frac{1}{2\pi} \int_{0}^{2\pi} -\frac{M}{V}\Bigl(cos\theta sin\theta(r_3^2-r_2^2)+r_2r_3cos{2\theta})\Bigr) \ d\theta d^3r $$
$$ I_{23}=\int_{}^{} 0 d^3r=0$$With $I_{22}=I_{33}$ I proceeded as follows

$$ \int_{}^{} \frac{M}{V}{r'}_1^2+{r'}_3^2 d^3r $$
$$ \int_{}^{} \frac{M}{V}{r'}_1^2+{r'}_2^2 d^3r $$

Then I did the transformation,

$$ \int_{}^{} \frac{M}{V}\Bigl(r_1^2-r_2^2sin^2\theta-2r_2r_3sin\theta cos\theta + r_3^2cos^2\theta\Bigr) d^3r $$
$$ \int_{}^{} \frac{M}{V}\Bigl(r_1^2+r_2^2cos^2\theta+2r_2r_3sin\theta cos\theta + r_3^2sin^2\theta\Bigr) d^3r $$

Unfortunately, I am now stuck on how to show that the two terms in the integral are equal.

 

[pasmith]

Use $$\begin{split}<br /> \cos^2 \theta &= \tfrac12(1 + \cos 2\theta) \\<br /> \sin^2 \theta &= \tfrac12(1 - \cos 2 \theta) \\<br /> \cos \theta \sin \theta &= \tfrac12 \sin 2\theta \\<br /> \end{split}$$ and recall that the average of sin or cos over a period is zero.

 

[Lambda96]

Thanks pasmith for your help , I was now able to show that $I_{22}=I_{33}$.