Mech_LS24
- 148
- 16
- TL;DR Summary
- In a structure with 5 pivot points and provided with deep groove ball bearings. I want to calculate the losses due to friction.
Hello,
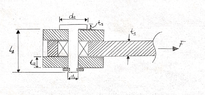
I have a structure with 5 pivot point and those are provided with deep groove ball bearings (see sketch).
Calculation for friction losses (deep grove ball bearing):
Parameters:
- Number of pivot points: 5
- Friction coefficient: 0.0010 - 0.0015 (Reference)
Total friction in the structure: 5*0.0015*100% = 0.75%
If compared to plain bearings:
Calculation for friction losses (plain bearing):
- Number of pivot points: 5
- Friction coefficient: 0.2 (worst case, Reference)
Total friction in the structure: 5*0.2*100% = 100%
I already imagined that a plain bearing has a higher impact on friction, but this amount does surprises me. Are those assumptions and calculations done in a correct manner? Or do I miss something here?
Besides, that 100% for plain bearings doesn't seem to be realistic. Does it mean the structure can't operate/move because the it can't 'overcome' the friction?
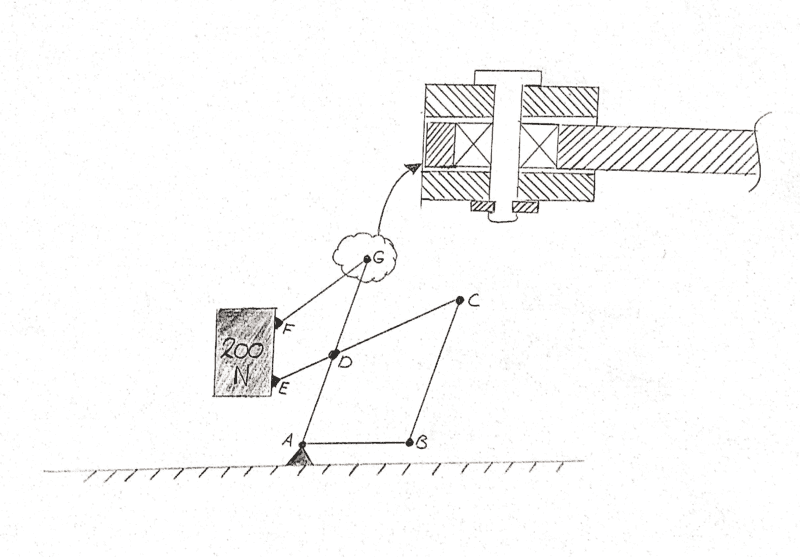
I have a structure with 5 pivot point and those are provided with deep groove ball bearings (see sketch).
Calculation for friction losses (deep grove ball bearing):
Parameters:
- Number of pivot points: 5
- Friction coefficient: 0.0010 - 0.0015 (Reference)
Total friction in the structure: 5*0.0015*100% = 0.75%
If compared to plain bearings:
Calculation for friction losses (plain bearing):
- Number of pivot points: 5
- Friction coefficient: 0.2 (worst case, Reference)
Total friction in the structure: 5*0.2*100% = 100%
I already imagined that a plain bearing has a higher impact on friction, but this amount does surprises me. Are those assumptions and calculations done in a correct manner? Or do I miss something here?
Besides, that 100% for plain bearings doesn't seem to be realistic. Does it mean the structure can't operate/move because the it can't 'overcome' the friction?