Andrei1
- 36
- 0
I would like to discuss the following problem.
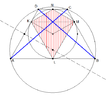
The quadrilateral $$ABCD$$ is inscribed into a circle of given radius $$R.$$ And it is circumscribed to a circle. The tangent points from the second circle produce another quadrilateral $$KLMN$$ such that $$S_{ABCD}=3S_{KLMN}.$$ Also $$\gamma$$ is the angle between diagonals $$AC$$ and $$BD.$$ Find the area of $$ABCD.$$
I have no ideas. I wonder if I have to search any regularities of $$ABCD.$$ All given elements seem to me "distanced" from each other.
The quadrilateral $$ABCD$$ is inscribed into a circle of given radius $$R.$$ And it is circumscribed to a circle. The tangent points from the second circle produce another quadrilateral $$KLMN$$ such that $$S_{ABCD}=3S_{KLMN}.$$ Also $$\gamma$$ is the angle between diagonals $$AC$$ and $$BD.$$ Find the area of $$ABCD.$$
I have no ideas. I wonder if I have to search any regularities of $$ABCD.$$ All given elements seem to me "distanced" from each other.