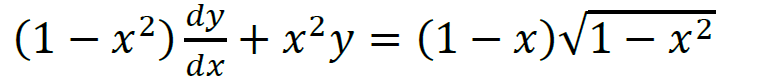Integrating Factors for Solving Linear Differential Equations

[mahmoud shaaban]

Can i have help with this linear differential equation ?
First, i divided by (1-x^2) to be like dy/dx + p(x)y= q(x). But i could not obtain Q(x).
Any help will be welcomed.
View attachment 6473

 

[MarkFL]

Let's look at what happens when we divide the RHS by $1-x^2$:

$$\frac{(1-x)\sqrt{1-x^2}}{1-x^2}=\frac{1-x}{\sqrt{1-x^2}}=\frac{1-x}{\sqrt{(1+x)(1-x)}}=\sqrt{\frac{1-x}{1+x}}$$

So, the ODE is now:

$$\d{y}{x}+\frac{x^2}{1-x^2}y=\sqrt{\frac{1-x}{1+x}}$$

Next, we want to compute the integrating factor:

$$\mu(x)=\exp\left(\int\frac{x^2}{1-x^2}\,dx\right)$$

Can you proceed?