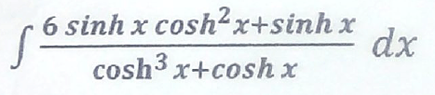Integration Problem - Can Someone Help?

[mahmoud shaaban]

Can someone help with this problem, i tried to solve it
using f'(x)/f(x) but couldn't figure it out.View attachment 5909

 

[Greg]

Hi mahmoud shaaban and welcome to MHB! :D

$$\begin{align*}\int\dfrac{6\sinh(x)\cosh^2(x)+\sinh(x)}{\cosh^3(x)+\cosh(x)}\,dx&=\int\tanh(x)\left(\dfrac{5\cosh^2(x)}{\cosh^2(x)+1}+1\right)\,dx \\
&=5\int\dfrac{\cosh(x)\sinh(x)}{\cosh^2(x)+1}\,dx+\int\tanh(x)\,dx\end{align*}$$

Can you continue?

 

[soroban]

$$\displaystyle \int \frac{6\sinh x\cosh^2x + \sinh x}{\cosh^3x + \cosh x}\,dx$$

$$\text{We have: }\;\int \left(\frac{3\sinh x\cosh^2\!x + \sinh x}{\cosh^3\!x + \cosh x} + \frac{3\sinh x\cosh^2\!x}{\cosh^3\!x + \cosh x}\right)\,dx$$

. . . $$=\;\int\left(\frac{3\sinh x\cosh^2\!x + \sinh x}{\cosh^3\!x + \cosh x} +\frac{3\sinh x \cosh x}{\cosh^2\!x + 1} \right)\,dx$$

. . . $$=\;\int \frac{3\sinh x\cosh^2\!x + \sinh x}{\cosh^3\!x + \cosh x}\,dx \;+\; \frac{3}{2}\int\frac{2\sinh x\cosh x}{\cosh^2\!x+1}\,dx$$

Can you finish it now?