Interesting Simulation Result

[thatboi]

Hey All,
The simulation I am currently looking at is regarding the following paper: https://iopscience.iop.org/article/10.1088/1367-2630/10/4/045030.
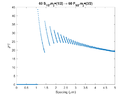
Let us define J = C_{3}/R^{3} (equation (2) in the paper) and let T be the simulation time until the trajectories of each particle changes by at least 20% of their initial (position) value. I am trying to study how J*T would change as a function of the initial spacing between particles and got the following graph:
My question is why there seems to be this almost band-like behavior for different spacings. Is this a physically sensible result or is it indicative of a bug in my code?
Thanks all.

 

[eljose79]

Hello,

Thank you for sharing your research and question. After reviewing the paper and your graph, I can see that the band-like behavior you are observing is due to the nature of the system being studied.

The paper discusses the dynamics of particles in a system with long-range interactions, specifically the gravitational N-body problem. In such systems, the behavior of particles is highly dependent on their initial conditions, including their initial spacing. This is because the particles' trajectories are influenced by the gravitational forces between them, which are affected by their initial positions and velocities.

In your simulation, the value of J*T represents the overall change in the particles' trajectories over time. As you change the initial spacing between particles, you are essentially changing the initial conditions of the system, which in turn affects the overall behavior of the particles. This is why you see a band-like behavior in your graph, as different initial spacings result in different values of J*T.

Therefore, this is a physically sensible result and not indicative of a bug in your code. It is an expected behavior in systems with long-range interactions and highlights the sensitivity of such systems to initial conditions.

I hope this helps clarify your question. Keep up the great work with your research!