- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading James Munkres' book, Elements of Algebraic Topology.
Theorem 6.2 on page 35 concerns the homology groups of the 2-dimensional torus.
I would appreciate some help with interpreting the term 'homologous to' as it relates to a part of the proof of Munkres' Theorem 6-2 concerning the homology groups of the torus.
The relevant part of the proof is as follows: (see end of my post for a complete copy of Theorem 6.2)
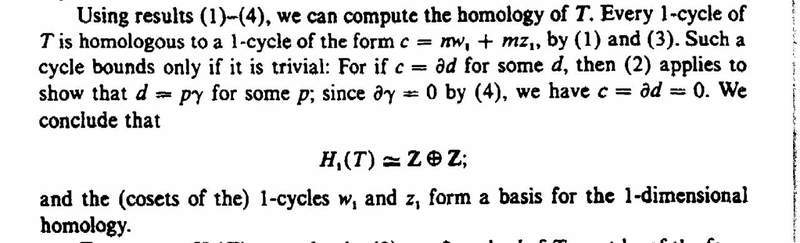
It seems that in proving that [itex] H_1 (T) = \mathbb{Z} \oplus \mathbb{Z} [/itex] Munkres has shown that every 1-cycle of T is homologous to a 1-cycle c of the form [itex] c = n w_1 + m z_1 [/itex], and, further, that the only boundaries of such cycles are trivial i.e. there are no boundaries of these cycles.
I basically follow the details of Munkres analysis ...
BUT ... to show that [itex] H_1 (T) = \mathbb{Z} \oplus \mathbb{Z} [/itex] we should show that every on cycle is of the form [itex] c = n w_1 + m z_1 [/itex], and, ... ... etc ...
... and not just that every 1-cycle is homologous to such as cycle ie that for every cycle [itex] c_1 [/itex] we have:
[itex] c - c_1 = \partial d [/itex] for some d ... ...
or is this just the same ...
Can someone please clarify this issue for me.
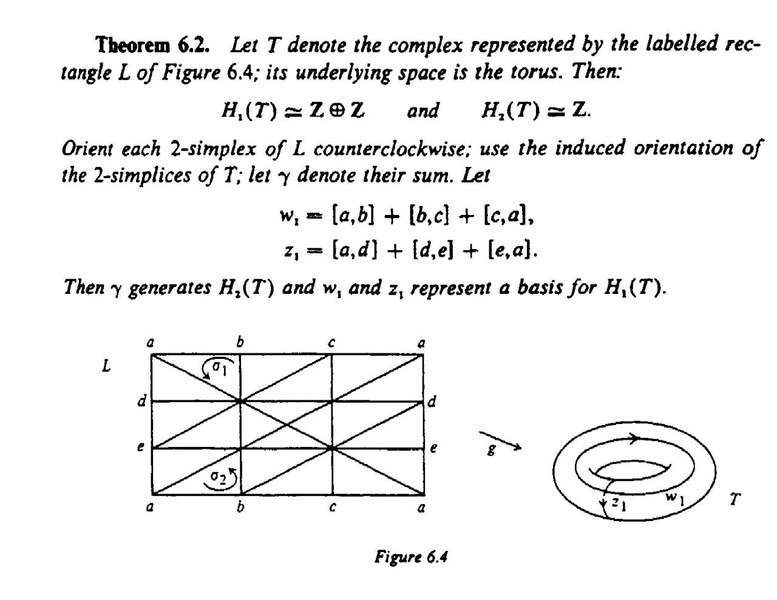
The details of Theorem 6.2 and its proof are as follows:

The definition of homologous is given in the following text from Munkres:
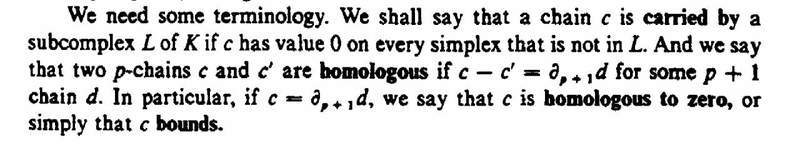
Hope someone can help,
Peter
Theorem 6.2 on page 35 concerns the homology groups of the 2-dimensional torus.
I would appreciate some help with interpreting the term 'homologous to' as it relates to a part of the proof of Munkres' Theorem 6-2 concerning the homology groups of the torus.
The relevant part of the proof is as follows: (see end of my post for a complete copy of Theorem 6.2)
It seems that in proving that [itex] H_1 (T) = \mathbb{Z} \oplus \mathbb{Z} [/itex] Munkres has shown that every 1-cycle of T is homologous to a 1-cycle c of the form [itex] c = n w_1 + m z_1 [/itex], and, further, that the only boundaries of such cycles are trivial i.e. there are no boundaries of these cycles.
I basically follow the details of Munkres analysis ...
BUT ... to show that [itex] H_1 (T) = \mathbb{Z} \oplus \mathbb{Z} [/itex] we should show that every on cycle is of the form [itex] c = n w_1 + m z_1 [/itex], and, ... ... etc ...
... and not just that every 1-cycle is homologous to such as cycle ie that for every cycle [itex] c_1 [/itex] we have:
[itex] c - c_1 = \partial d [/itex] for some d ... ...
or is this just the same ...
Can someone please clarify this issue for me.
The details of Theorem 6.2 and its proof are as follows:
The definition of homologous is given in the following text from Munkres:
Hope someone can help,
Peter
Attachments
Last edited:

