- #1
Ry122
- 565
- 2
Member warned about posting without the homework template and with no effort shown.
Hi
Could someone please help me to understand what questions a) and b) here are asking for?
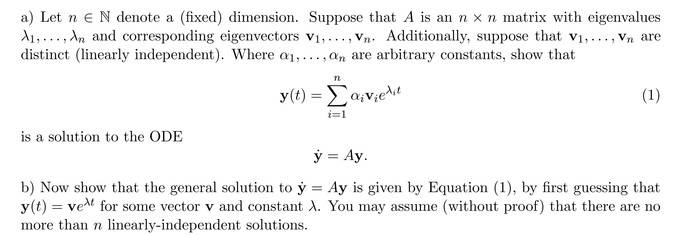
Could someone please help me to understand what questions a) and b) here are asking for?