Investigating Limit of Piecewise Function

In summary, the conversation discusses investigating the limit of f(x) as x approaches 2, both from the left and right side, as well as the overall limit. The limit is found to be 4 from the left and 2 from the right, but the limit does not exist due to the left-hand limit not equaling the right-hand limit. The speaker expresses a desire to understand limits better, particularly in calculus 3.
  • #1
nycmathguy
Homework Statement
Graphs & Functions
Relevant Equations
Piecewise Functions
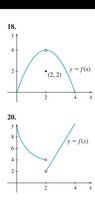
Use the graph to investigate

(a) lim of f(x) as x→2 from the left side.

(b) lim of f(x) as x→2 from the right side.

(c) lim of f(x) as x→2.

Question 20

For part (a), as I travel along on the x-axis coming from the left, the graph reaches a height of 4. The limit is 4. It does not matter if there is a hole at (2, 4), right?

For part (b), as I travel along on the x-axis coming from the right, the graph reaches a height of 2. The limit is 2. It does not matter if there is a hole at (2, 2), right?

For part (c), LHL DOES NOT EQUAL RHL.

I conclude the limit does not exist.

You say?
 

Attachments

  • Screenshot_20210613-160046_Drive.jpg
    Screenshot_20210613-160046_Drive.jpg
    9.6 KB · Views: 126
Physics news on Phys.org
  • #3
Mark44 said:
Yes to all.

I got another question right. It's a miracle. I need to understand this limit idea better. I know that limits in calculus 3 are more involved.
 

FAQ: Investigating Limit of Piecewise Function

What is a piecewise function?

A piecewise function is a mathematical function that is defined by different equations over different intervals of its domain. This means that the function has different rules for different parts of its input values.

How do you determine the limit of a piecewise function?

To determine the limit of a piecewise function, you need to evaluate the limit of each piece of the function separately. This involves finding the limit of each equation that makes up the piecewise function and then taking the limit of the overall function by considering the limits of each piece at the point where the function changes from one equation to another.

What are the common types of piecewise functions?

The most common types of piecewise functions are step functions, absolute value functions, and greatest integer functions. Step functions have a constant value over each interval, absolute value functions have a V-shaped graph, and greatest integer functions have a staircase-like graph.

How do you graph a piecewise function?

To graph a piecewise function, you need to plot the points for each equation that makes up the function. Then, you can connect the points with a solid or dashed line depending on whether the function is continuous or not. It is important to also label the different pieces of the function and indicate where the function changes from one equation to another.

What are the applications of piecewise functions in real life?

Piecewise functions have many applications in real life, such as in economics, physics, and engineering. For example, they can be used to model cost functions with different rates for different intervals, describe the motion of an object with different equations for different parts of its trajectory, or design a circuit with different components that operate under different conditions.

Similar threads

Use Graph To Investigate Limit
Replies
7
Views
1K
Investigating A Limit Via Graph
Replies
2
Views
995
Investigate Limit of Piecewise Function
Replies
11
Views
1K
Use the graph of f(x) to investigate the limit
Replies
6
Views
1K
Use Graph to Determine Limit: Calculating Limits with Piecewise Functions
Replies
3
Views
1K
Use Graph To Investigate Limit
Replies
6
Views
1K
How Do Limits Behave for Piecewise Functions at Specific Points?
Replies
30
Views
2K
What Happens to f(x) as x Approaches 2?
Replies
15
Views
1K
Proving piecewise function is k-differentiable
Replies
5
Views
1K
Finding the limits of a piecewise function
Replies
7
Views
2K

Hot Threads

Recent Insights

Back
Top