Applying the 1D Gauss law to charge densities

[Noki Lee]

Can we apply the 1d equation (dE/dx = labmda/epsilon0)dEdx=λϵ0 to the first and the second figures?

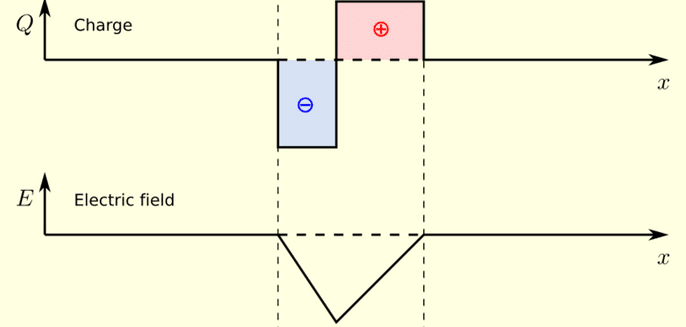
But, in the 2nd case,

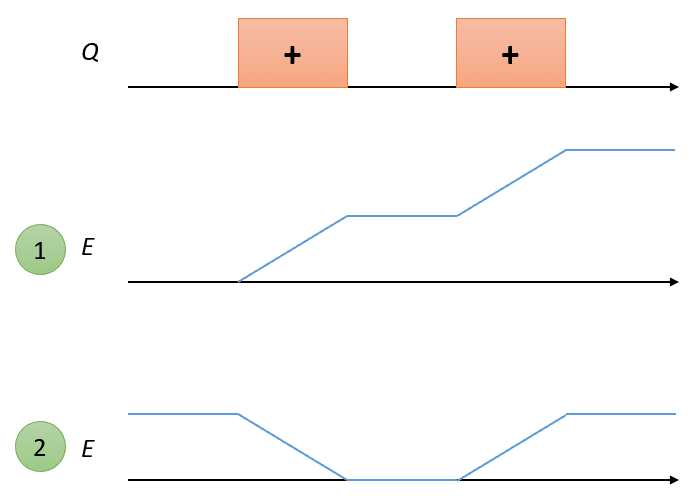
if we integrate the charge density, some field exists between the two charge densities. Intuitively, it should be like the last figure.
What's wrong with this?

 

[phyzguy]

Your intuition is wrong. The E-field on the left and right point in opposite directions. This is what you get in your case (1) if you add a (negative) constant of integration so E is zero between the two charges.

 

[Noki Lee]

Your intuition is wrong. The E-field on the left and right point in opposite directions. This is what you get in your case (1) if you add a (negative) constant of integration so E is zero between the two charges.

I mistook the intuition, did you mean this figure?

But why we can't apply the above 1D equation?

 

[phyzguy]

Yes, I mean that figure. You can use the above 1D equation, but when you do the integration, you always have a constant of integration that you have to determine from the boundary conditions. So your graph (1) needs to have a negative constant added to it so it looks like the graph (2) in post #3. Do you understand?

 

[Noki Lee]

Yes, I mean that figure. You can use the above 1D equation, but when you do the integration, you always have a constant of integration that you have to determine from the boundary conditions. So your graph (1) needs to have a negative constant added to it so it looks like the graph (2) in post #3. Do you understand?

I got it, thank you.