Investigating Wake Profiles of Different Objects

[yonese]

Hi,

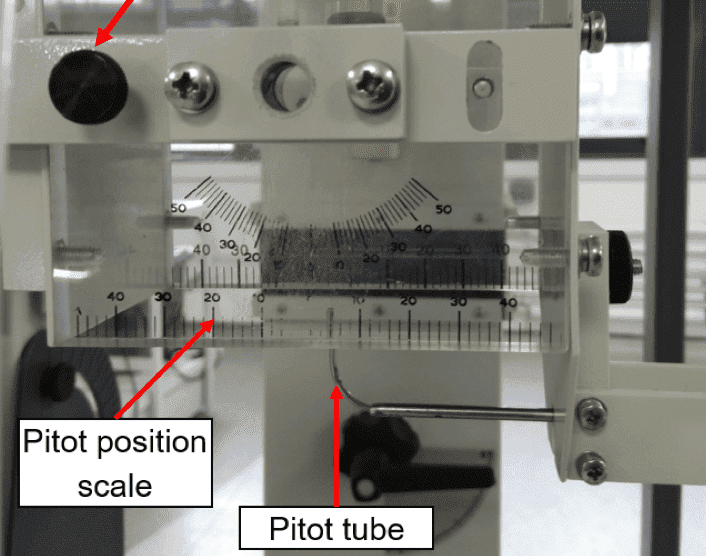
Recently I did a drag lab which included doing an experiment to find the wake profile of 3 different objects. In order to measure the air speed downstream the object, a Pitot tube is used as shown in the image below. The end of the Pitot tube is vertical, and aligned with the flow direction. The position of the Pitot tube is fixed at the channel centre, and it can move horizontally from the left wall (x=+50 mm) to the right wall (x=-50 mm), with x=0 matching the location of the axis of the object. Taking different readings at different x locations, I found a velocity profiles of each object.

Below are the velocity profiles of 3 different objects (a cylinder, prism, and an airfoil)

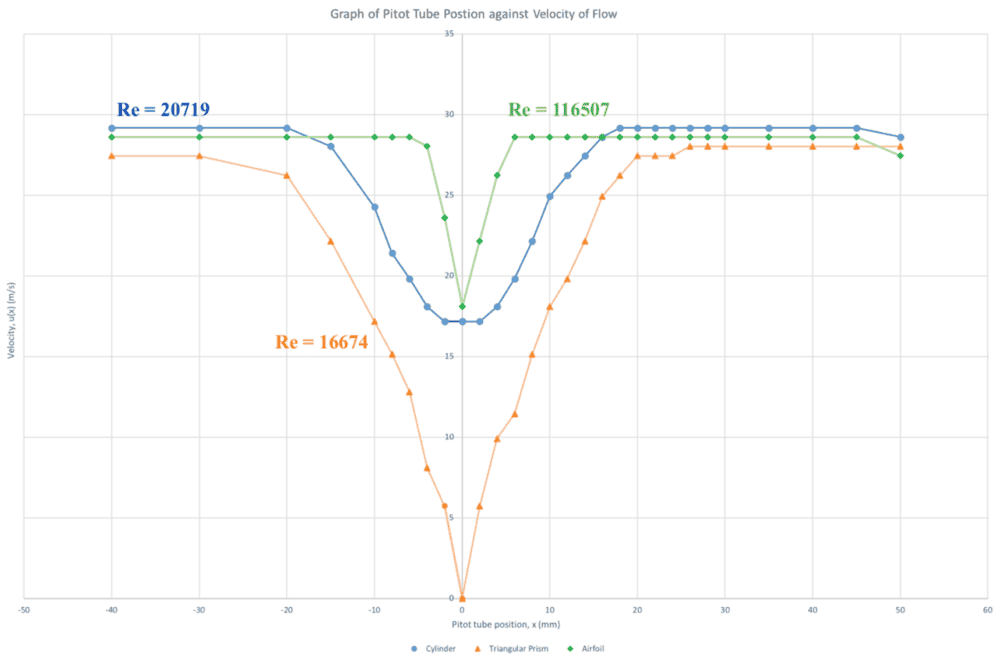
From my lab results, I was able to conclude that the larger the drag coefficient of an object, the larger it’s profile width. The cylinder and airfoil profiles came out as expected, but I can't seem to explain the triangular prism's velocity drop to null at the centreline. I know it's because the pitot tube reading and the open tube reading had the same value, meaning there was no height difference, and therefore 0 velocity as I used the equations pd= ρ(water)gΔh for the dynamic pressure and u=√2pd/ρ(air)... Why does the flow separate like an airfoil instead a cylinder, as i expected it to be? What has its flow separation and connection to wake development affected by the shape or it's Re number?

I assume it would be due to experimental error.

Why do the wakes of cylinder and triangular prism differ? What about the airfoil? Why/how are the drag coefficients different?Thanks.

 

[jrmichler]

I assume it would be due to experimental error.

No. NEVER assume that unexpected results are experimental error. You did a good job of describing how you got this reading, so assume that the reading is correct.

Now you need to understand why your data is what it is. What is the orientation of the prism to the flow - flat face leading or point leading? Is the published drag coefficient different for those two cases? Why? Did you add some smoke so that you could see the flow?

There is some good discussion of two dimensional drag in Fluid-Dynamic Drag, by Hoerner: https://hoernerfluiddynamics.com/. That book is a standard reference on the subject of drag, and is well worth the price.

 

[Arjan82]

If the triangle was pretty wide (say an equilateral triangle), the point is in the flow direction, the Reynolds number is high enouch and you are measuring fairly close to the triangle (say, within the length of one of the sides) then it doesn't really surprise me that you have zero velocity there. There is quite a recirculation area in that case. See for example:

(this is actually still quite a low Reynolds number, around 20, see here)

 

[yonese]

No. NEVER assume that unexpected results are experimental error. You did a good job of describing how you got this reading, so assume that the reading is correct.

Now you need to understand why your data is what it is. What is the orientation of the prism to the flow - flat face leading or point leading? Is the published drag coefficient different for those two cases? Why? Did you add some smoke so that you could see the flow?

There is some good discussion of two dimensional drag in Fluid-Dynamic Drag, by Hoerner: https://hoernerfluiddynamics.com/. That book is a standard reference on the subject of drag, and is well worth the price.

Hi, thanks for commenting! :)

The prism was supposedly orientated face leading, and I wondered whether it could have been facing the wrong way with point leading, causing the flow to look the way it does? (As this was an online laboratory with my teacher doing the lab live, but it was hard to see clearly what was happening in detail). There was no smoke.

The experimental drag coefficient was 2.43 compared to a published coefficient of 2.

 

[yonese]

If the triangle was pretty wide (say an equilateral triangle), the point is in the flow direction, the Reynolds number is high enouch and you are measuring fairly close to the triangle (say, within the length of one of the sides) then it doesn't really surprise me that you have zero velocity there. There is quite a recirculation area in that case.

Thanks for commenting! :)

Although I wasn't given the exact dimensions for the triangle, it seems to look the lengths are equal. With that being said, your explanation would make a lot of sense. I hadn't thought that recirculation could occur, as I only expected to see them in wakes past a shape. I can't say how close the triangle is measured but looking at the picture in my question, it seems to be pretty close. What relationship does the distance between the triangle and the flow have that it would be normal to have 0 velocity?

 

[Arjan82]

Well, that relation is given by the Navier-Stokes equations . So it is not easy to say, and also Reynolds number dependent.

By the way, if the prism is oriented face leading, you can also get 0 velocity in the wake as I do not expect the flow to stay attached to the downstream sides for a 30 degree inward slope.