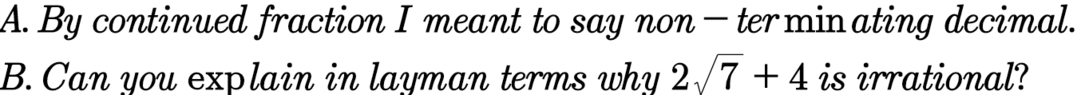- #1
You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Is 2√(7)+4 an Irrational Number?
- MHB
- Thread starter nycfunction
- Start date
-
- Tags
- Rational
Mathematics news on Phys.org
- #2
HallsofIvy
Science Advisor
Homework Helper
- 42,895
- 981
What, exactly, do you mean by "continued decimal number"? If you mean simply that it is not a terminating decimal, a rational number such as 1/3 has that property. That's not the reason $2\sqrt{7}+ 4$ is irrational.
First, the rational numbers are "closed under subtraction and division" so if $x= 2\sqrt{7}+ 4$ were rational so would be $\sqrt{7}= (x- 4)/2$. And if $\sqrt{7}$ were rational then there would exist integers, a and b, with no common factors, such that $\sqrt{7}= \frac{a}{b}$. From that $7= \frac{a^2}{b^2}$ and $a^2= 7b^2$. That is, $a^2$ has a factor of 7 and, since 7 is a prime number, a has a factor of 7. a= 7n for some integer n so $a^2= 49n^2= 7b^2$. Then $b^2= 7n^2$ so, as before, b has a factor of 7, contradicting the fact that a and b has no factors in common.
First, the rational numbers are "closed under subtraction and division" so if $x= 2\sqrt{7}+ 4$ were rational so would be $\sqrt{7}= (x- 4)/2$. And if $\sqrt{7}$ were rational then there would exist integers, a and b, with no common factors, such that $\sqrt{7}= \frac{a}{b}$. From that $7= \frac{a^2}{b^2}$ and $a^2= 7b^2$. That is, $a^2$ has a factor of 7 and, since 7 is a prime number, a has a factor of 7. a= 7n for some integer n so $a^2= 49n^2= 7b^2$. Then $b^2= 7n^2$ so, as before, b has a factor of 7, contradicting the fact that a and b has no factors in common.
Last edited by a moderator:
- #3
nycfunction
- 12
- 0
HallsofIvy said:What, exactly, do you mean by "continued decimal number"? If you mean simply that it is not a terminating decimal, a rational number such as 1/3 has that property. That's not the reason [tex]2\sqrt{7}+ 4[/tex] is irrational.
First, the rational numbers are "closed under subtraction and division" so if [tex]x= 2\sqrt{7}+ 4[/tex] were rational so would be [tex]\sqrt{7}= (x- 4)/2[/tex]. And if [tex]\sqrt{7}[/tex] were rational then there would exist integers, a and b, with no common factors, such that [tex]\sqrt{7}= \frac{a}{b}[/tex]. From that [tex]7= \frac{a^2}{b^2}[/tex] and [tex]a^2= 7b^2[/tex]. That is, [tex]a^2[/tex] has a factor of 7 and, since 7 is a prime number, a has a factor of 7. a= 7n for some integer n so [tex]a^2= 49n^2= 7b^2[/tex]. Then [tex]b^2= 7n^2[/tex] so, as before, b has a factor of 7, contradicting the fact that a and b has no factors in common.
See attachment.
Attachments
Last edited:
Similar threads
- Replies
- 19
- Views
- 2K
- Replies
- 5
- Views
- 2K
- Replies
- 4
- Views
- 3K
- Replies
- 27
- Views
- 3K
- Replies
- 16
- Views
- 3K
- Replies
- 7
- Views
- 2K
- Replies
- 2
- Views
- 1K
- Replies
- 6
- Views
- 2K
- Replies
- 7
- Views
- 2K
- Replies
- 10
- Views
- 1K
Share: