Is dx Negative in Non-Standard Analysis?

[fresh_42]

@etotheipi

If in doubt, always look for the tangents. They are hidden somewhere when it comes to differentiation.

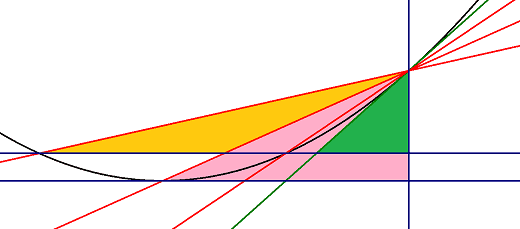
It is the quotient $\dfrac{\Delta f(x)}{\Delta x}$, i.e. the slope of the hypotenuse of the triangle which must be considered, not just one kathode, whether as $\Delta x$ or as $dx$. The limiting process of simultaneously both kathodes, the lengths of the difference of function values and the lengths of the $x$ intervals. The quotient does the trick!

If it stands alone, it abbreviates something else and things are more complex, namely a differential form. This is the function that attaches another function to each point: $x \longmapsto L_x$ (see post #6). My picture used $y=\frac{1}{5}x^2$ and $x_0=3$. So $dx$ attaches the function $\tilde{x} \longmapsto \frac{2}{5} \tilde{x}$, which has at $x_0=3$ the value $\frac{6}{5}$. Here we changed the origin of the curve space $(0,0)$ into the origin at the tangent space (the green line) $(3,\frac{9}{5})$ which becomes our new origin if we talk about the tangent space as a vector space. Hence the tangent at $x_0=3$ is $f'(\tilde{x})=(\frac{2}{5}\cdot 3) \tilde{x}$ which is a linear function in the coordinate system of the tangent. In old coordinates it is $f'(x)=\frac{6}{5}x - \frac{9}{5}$. One of the things which adds more confusion and requires to distinguish the curve from the tangents. Every single tangent is a line, i.e. a one dimensional vector space: different points $x_0$, different tangent spaces. At school it is all in one coordinate system, whereas physicists have to distinguish the $(x,y)$ space above from all the possible green lines, e.g. the one I drew in the picture with $(\tilde{x},f'(\tilde{x}))$ coordinates. That's why a tangent should always be considered as the pair $(x_0, L_{x_0})$, the point of evaluation and the direction (slope) which it points to. This distinction is basically the secret behind all other perspectives under which a differentiation can be seen.

 

[etotheipi]

And, if you sit down and prove this, then it does not rely on cancelling $dx$ as in:
$$\int_a^b f(u)\frac{du}{dx}dx = \int_{u(a)}^{u(b)} f(u)du$$
Simply cancelling the $dx$ here is not a proof! In real analysis (pure mathematics) it must be proved otherwise.

That's helpful, thank you. My "rule" is that we're "allowed" to effectively cancel infinitesimals but not operators, as in $$\frac{dy}{dx} dx = dy$$ whilst I'd need to change the following $$\frac{d}{dx} (\frac{dy}{dx}) dx = \frac{d(\frac{dy}{dx})}{dx} dx = d(\frac{dy}{dx})$$ The difference isn't too noticeable in the below example, but it seems to be important in things like operator equations.

But I think like you say it's more a case of taking advantage of the notation.

 

[sysprog]

That's helpful, thank you. My "rule" is that we're "allowed" to effectively cancel infinitesimals but not operators, as in $$\frac{dy}{dx} dx = dy$$ whilst I'd need to change the following $$\frac{d}{dx} (\frac{dy}{dx}) dx = \frac{d(\frac{dy}{dx})}{dx} dx = d(\frac{dy}{dx})$$ The difference isn't too noticeable in the below example, but it seems to be important in things like operator equations.

But I think like you say it's more a case of taking advantage of the notation.

It's not precisely a "below example"; it's above the text that refers to it; but it's nevertheless a good example; and, at least in my view, a little bit of notational abuse can sometimes be rather good.

 

[WWGD]

$dx$ is not a number and doesn't assume any values. $x$ is assumed here to be a real variable, so it and $f(x)$ assume real values. But $dx$ is a notational device to indicate, along with the $\int$ symbol, integration with respect to the variable $x$.

You can say, for example, let $x = 1$, then $f(1)$ is well-defined, but $d1$ or $dx \big | _{x = 1}$ has no meaning.

I meant when you integrate it does take numerical values. The simplest case, integrate 1dx from 0 to 1. The answer will be 1(1-0)=1. Dx is a measure of the width of an interval. When we do a Riemann integral, we're doing an infinite sum f(x_j)dx_j. dx_j := x_{j+1}-x_j. So dx_j is the measure of the length of an interval. Sure, with infinite Riemann sums we do not consider each, but you may use a partition into finitely-many rectangles and assign a length to each. You may then say dx_i:=x_{j+1}-x_j=0.5, etc. So it is not just a place-holder, though maybe you said it in a different sense. So you can say dx_i or dx at the ith interval assumes the value x_{j+1}-x_j = Real number.

 

[PeroK]

I meant when you integrate it does take numerical values. The simplest case, integrate 1dx from 0 to 1. The answer will be 1(1-0)=1. Dx is a measure of the width of an interval. When we do a Riemann integral, we're doing an infinite sum f(x_j)dx_j. dx_j := x_{j+1}-x_j. So dx_j is the measure of the length of an interval. Sure, with infinite Riemann sums we do not consider each, but you may use a partition into finitely-many rectangles and assign a length to each. You may then say dx_i:=x_{j+1}-x_j=0.5, etc. So it is not just a place-holder, though maybe you said it in a different sense.

There are no sub-intervals in an integral and it is not an infinite sum. It's the limit of a sequence of finite sums. An infinite sum is something of the form:
$$\sum_{n= 1}^{\infty} a_n$$
If the integral was an infinite sum, it would defined as such, with the approriate width $dx_j$ specified! There are no $dx_j$ in an integral. There is only the symbol $dx$, which is neither a number nor an interval.

 

[WWGD]

There are no sub-intervals in an integral and it is not an infinite sum. It's the limit of a sequence of finite sums. An infinite sum is something of the form:
$$\sum_{n= 1}^{\infty} a_n$$
If the integral was an infinite sum, it would defined as such, with the approriate width $dx_j$ specified! There are no $dx_j$ in an integral. There is only the symbol $dx$, which is neither a number nor an interval.

You do have an infinite sum where the terms are of the form f(x_j)dx_j and, you specify additional conditions by quantifying over all sums where dx_j goes to 0.
Well, yes, the limit of a sum, not necessarily an infinite sum. The with dx_j is a variable, and you do not specify it for infinitely-many values, but it does assume values. You may partition [0,1] into [0,1/2], [1/2,1]. Then dx_(interval [0,1/2])=1/2-0 and dx_(interval[1/2,1])=1-1/2=1/2. So you do assign actual numerical values. Of course, in the limit you do a quantification over all intervals , over all sums as width goes to zero but these are actual widths. But maybe it is a semantic thing and we are saying the same thing in different ways.

 

[PeroK]

But maybe it is a semantic thing and we are saying the same thing in different ways.

Perhaps, but just as example. In the integral
$$\int_0^1 x^2 dx$$
What is the value of the width(s) $dx_j$ that you would use?

 

[WWGD]

The Riemann integral when it converges to R is the limit of a net of intervals/partitions ordered by inclusion into the Reals. You want, if Partition 1 subset Partition 2, both in an eps- neighborhood of R. You assign a Riemann sum to each partition so that , by net convergence, every subpartition is eventually in any eps-neighborhood of R. I don't know if I explained it well, but I think the net convergence issue is a bit unwieldy.

 

[WWGD]

Guess my post was confusing. My point is that the convergence of the Riemann integral is not your standard convergence but instead convergence as a net.

 

[sysprog]

Guess my post was confusing. My point is that the convergence of the Riemann integral is not your standard convergence but instead convergence as a net.

How about looking at it as a state space?

 

[PeroK]

I've actually got some notes on doing the following integral from first principles, by calculating the limits of the upper and lower sums for a set of regular partitions:
$$\int_a^b x^2 dx$$
Note that the definite integral (unlike $dx$) is a real number!

We take $P_n$ as the partition of $[a, b]$ into $n$ equal sub-intervals of width $\frac{b -a}{n}$. Note that each partition has sub-intervals, but the integral itself does not.

The minimum value of $x^2$ on each interval is at the lower end and the maximum at the higher end. This gives us the upper and lower sums as:
$$L_n = \sum_{k = 0}^{n-1} (a + \frac{k}{b-a})^2(\frac{b-a}{n}) \\
= (b-a)[a^2 + (b-a)^2\frac{(n-1)(2n-1)}{6n^2} + \frac{a(b-a)(n-1)}{n}] \\
U_n = \sum_{k = 1}^{n} (a + \frac{k}{b-a})^2(\frac{b-a}{n}) \\
= (b-a)[a^2 + (b-a)^2\frac{(n+1)(2n+1)}{6n^2} + \frac{a(b-a)(n+1)}{n}]$$
Each of the $L_n$ must be an under-estimate of the integral and each of the $U_n$ must be an over-estimate. If they both converge to the same number, then that number is the integral.

Now, we have:
$$\lim_{n \rightarrow \infty} L_n = \frac 1 3 (b^3 - a^3) \\
\lim_{n \rightarrow \infty} U_n = \frac 1 3 (b^3 - a^3)$$
The definite integral, therefore, is well-defined and we have:
$$\int_a^b x^2 dx = \frac 1 3 (b^3 - a^3)$$

 

[archaic]

Yes! This reminded me of something that happened with me. I was trying to determine the work of gravity (or $F_{spring}$, I forgot) after having fixed a positive direction.
When I considered the position to be increasing, I found the correct answer, but then, when considering the case where the position was decreasing, I got a wrong one. I then caught that in $\vec F\cdot d\vec s=|F|\,|ds|\cos\theta$ the $ds$ must be negative, so $|ds|=-ds$.

 

[archaic]

Perhaps you didn't phrase this precisely, but a real number is either zero or it's not. It can't "go to zero". In any case, $dx$ in the integral is not a real number.

Hm, hasn't Abraham Robinson formalised infinitesimals?

 

[PeroK]

Hm, hasn't Abraham Robinson formalised infinitesimals?

In post #46 I showed how the integral of $x^2$ could be done from first principles using "standard" real analysis. I invite you to do the same using non-standard analysis, where $dx$ is an infinitesimal.

The following proposition is a cornerstone of real analysis. Let $x \in \mathbb R$ with $x \ne 0$.

1) Either $x > 0$ or $x < 0$, but not both.

2) If $x > 0$, then $\exists \ y \in \mathbb R, \ s.t. \ 0 < y < x$.

If this proposition fails for $dx$, then $dx \notin \mathbb R$.

 

[archaic]

If this proposition fails for $dx$, then $dx \notin \mathbb R$.

Right, REAL analysis.

I invite you to do the same using non-standard analysis, where $dx$ is an infinitesimal.

No experience with non-standard analysis .