Is Fermi-Dirac Probability Part B Solvable?

[falcon555]

Hi dear friends
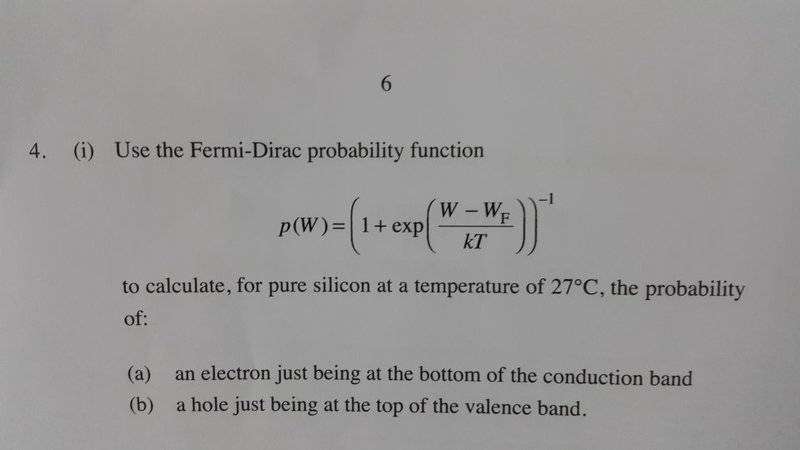
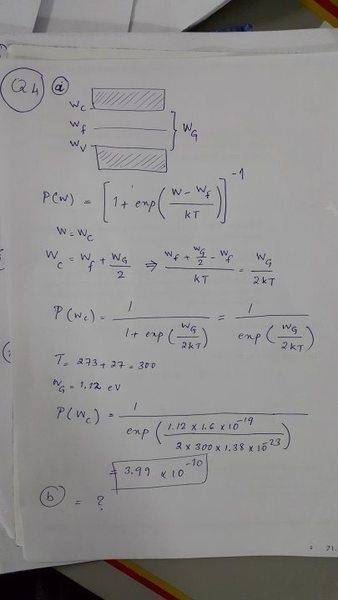
Please reffer to my work , I did part ( a )
Can someone help me to solve part( b )
Please

 

[rude man]

(a) is correctly done.
(b):
My textbook gives the probability distribution for holes as not W(p) as it is for electrons, but as 1 - W(p). Without a believable rationale, but I'm sure it's correct, because later on they use that expression for deriving the totality of free carriers for electrons and for holes and get roughly the same number for each, which has to be correct.

So, bottom line, if you use 1 - W(p) for the holes, and use W_v in lieu of W_c, and proceedig exactly as you did in part (a), you will get your answer. Do that and let us know what you come up with.

 

[falcon555]

If we assume the number of electrons and the holes are the same ,then we suppose to get the same value

Or you mean to say

1 - 3.99 × 10^(-10) = 2.99 × 10^(-10)

 

[rude man]

If we assume the number of electrons and the holes are the same ,then we suppose to get the same value
View attachment 105749

Right. The answer for (b) is the same as the answer for (a).

But you derived p(W_h) = {1 - exp(W_G/kT)}^-1 when it should be {1 + exp(W_G/kT)}^-1 which may be just a typo.

Or you mean to say
1 - 3.99 × 10^(-10) = 2.99 × 10^(-10)

I don't think anyone would say that! (Look at that statement more carefully! )

 

[falcon555]

Ok
It means without any extra calculation we can asume that both the values are the same as a rule of thumb, right?
Because the holes and the electrons are generated in pairs, right?

 

[rude man]

Ok
It means without any extra calculation we can asume that both the values are the same as a rule of thumb, right?
Because the holes and the electrons are generated in pairs, right?

That's what I always suspected, but I decided to make sure by going with the formulas. I wasn't sure if an electron at the very bottom of the conduction band implied a hole at the very top of the valence band until I worked the formulas.

It's certainly true that, in an intrinsic (undoped) semiconductor like Si, a hole is created when and only when an electron is kicked up to the conduction band, and vice-versa.

 

[falcon555]

Thank you very much bro for helping me.
You are great.

Cheers

 

[rude man]

Thank you very much bro for helping me.
You are great.

Cheers

You're very welcome. Educational for me too!
P.S. is that your cat? Lovely boy!