- #1
bolzano95
- 89
- 7
- Homework Statement
- Does friction exist?
- Relevant Equations
- More in the solution
What I know is that the force of friction and the normal force are the components of a contact force. So force of friction is related to the contact force. Friction is also related to the normal force by equation ##F_t= μ\cdot N##.
In this case (because the block is not moving) N=0 and therefore ##F_t=0## and there is no friction.
What bothers me is the fact that the wall and the block are in contact so there must be a force acting on the block? Am I even thinking right? So what would be this force?
Also: Does anybody knows how did we get this equation ##F_t= μ\cdot N##?
I know that the experiments showed that on the horizontal surface the force of friction is proportional to the mass of the object we observe and the ##F_t= μ\cdot N## is true.
But how do we get the same conclusion in a vertical case like this?
In other words: were there experiments also in this case or we just took a concept and put it in a vertical direction?
If so - what happens if we side a block vertically between two walls and we have been given a static coefficient? Does the friction acts on the body or not?
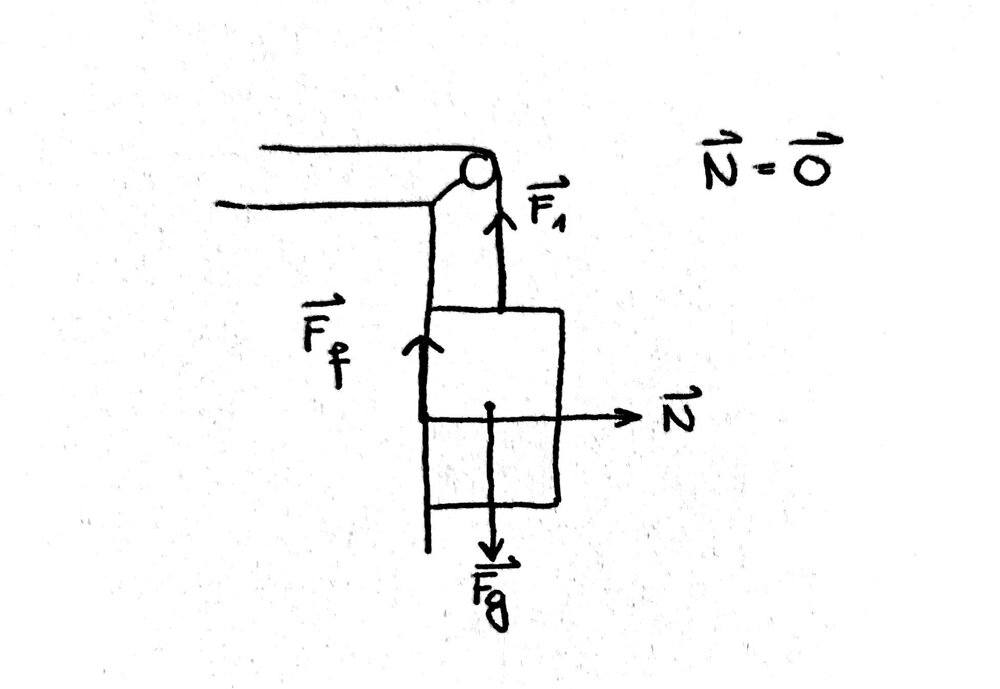
In this case (because the block is not moving) N=0 and therefore ##F_t=0## and there is no friction.
What bothers me is the fact that the wall and the block are in contact so there must be a force acting on the block? Am I even thinking right? So what would be this force?
Also: Does anybody knows how did we get this equation ##F_t= μ\cdot N##?
I know that the experiments showed that on the horizontal surface the force of friction is proportional to the mass of the object we observe and the ##F_t= μ\cdot N## is true.
But how do we get the same conclusion in a vertical case like this?
In other words: were there experiments also in this case or we just took a concept and put it in a vertical direction?
If so - what happens if we side a block vertically between two walls and we have been given a static coefficient? Does the friction acts on the body or not?