Is my equation for projectile trajectory accurate?

[person123]

I derived an equation for the trajectory of a projectile. Given the height and distance of the projectile, and the initial velocity, it determines the initial angle. When plugging it into desmos, it seemed to make sense (https://www.desmos.com/calculator/pfylvs4tau), but I still can't be sure.

I first determined the vertex of the parabola. I knew two points of the parabola and the derivative at one of these points. This gave me the following:

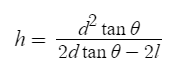
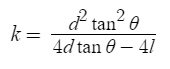
where d is the horizantal distance from the target, l is the vertical distance, and θ is the initial angle.

Since I knew the initial kinetic energy is the same is the product of the mass, acceleration due to gravity, and the height k, I was able to solve for the velocity v. By moving around the variables in this equation, it gave me

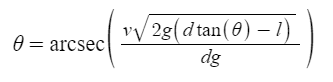
Does my method seem correct? Do the equations seem correct? (There's also the problem that I can't get theta completely to one side, although that's not really a physics problem).

 

[Ibix]

If k is the height, what's h?

Since I knew the initial kinetic energy is the same is the product of the mass, acceleration due to gravity, and the height k,

This is incorrect unless it's a vertical shot. The change in kinetic energy is equal to the change in gravitational potential, but the kinetic energy at the top of the trajectory is not zero in general.

 

[person123]

If k is the height, what's h?

h is the horizontal distance from the object's initial location to the vertex of the parabola.

This is incorrect unless it's a vertical shot. The change in kinetic energy is equal to the change in gravitational potential, but the kinetic energy at the top of the trajectory is not zero in general.

Sorry about that—that is a problem with the explanation of my work. The potential energy at the vertex would be equal to half of the product of mass and the initial vertical velocity squared. My work uses this instead of what I wrote previously.

 

[Ibix]

So - you are launching from x=h, y=l to hit a target at x=0, y=0. The shot is initially launched at speed v at angle $\theta$ to the horizontal. The peak of the arc is x=d, y=k.

Is that right?

 

[person123]

No. You initially launch at position (0,0). For the peak of the arc, I just used the standard notation for a vertex of a parabola, (h,k). The target is the coordinate (d,l). (I used l because I was running out of letters). As you said, the target is launched with velocity v at an angle θ above the horizontal.

 

[Ibix]

Sorry - this slipped off my todo list.

I agree all your expressions. To solve your last one, I suggest backing up a couple of steps. You'll have had something like $$\frac{d^2}{\cos^2\theta}=\frac{2v^2}{g}(d\tan\theta-l)$$If you multiply both sides by $\cos^2\theta$ and apply double-angle formulae you should be able to get an expression in terms of the sine and cosine of $2\theta$. That ought to be solvable.

 

[person123]

I have decided on putting this equation to the test. I'm going to shoot a projectile (a marble) at different angles and velocities and see if it lands at the predicted location. I decided on using a makeshift slingshot to propel the marble, but I have a question on what to make it out of.

The easiest solution would be to use rubber bands. However, it seems that rubber bands don't follow Hooke's law with nearly as much accuracy as would an actual spring (http://c21.phas.ubc.ca/sites/default/files/rubber_band_write_up.pdf https://www.wired.com/2012/08/do-rubber-bands-act-like-springs/). I'm wondering if that would be a significant enough inaccuracy to make my results unreliable—it's quite difficult to extrapolate the data provided for my situation.

I would use springs instead, but the only ones I found were really too stiff for this experiment.

 

[person123]

Edit:

I tested the spring constant for rubber bands, and it gave me something pretty linear. I did it for both one rubber band and 4 rubber bands attached in a chain. Here are the results:
https://www.desmos.com/calculator/w0dd8dpuf87.