- #1
pkc111
- 225
- 26
This is my latest attempt at trying to summarise but I feel there maybe a mistake in the wording. Any help would be appreciated.
Thanks.

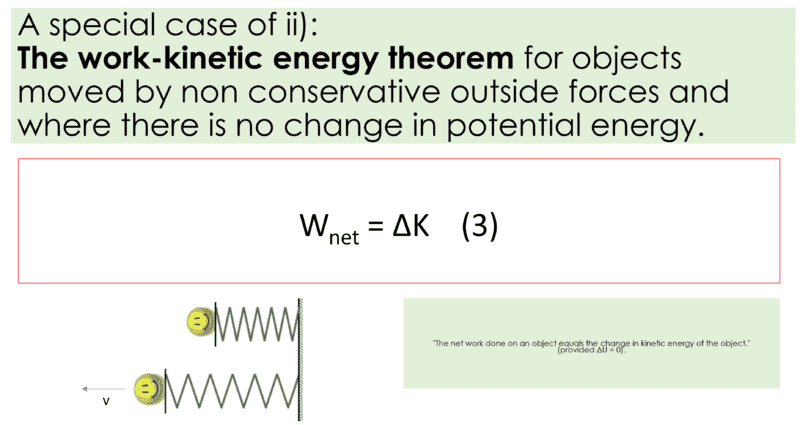
Thanks.