Eclair_de_XII
- 1,082
- 91
- Homework Statement
- Consider the curve ##r=\tan t## for ##t\in[0,2\pi]##. Substituting ##t=0## into this equation implies that ##r=0##. We convert this into rectangular coordinates.
We get the curve ##\sqrt{x^2+y^2}=\frac{y}{x}##, which is oddly undefined for some values of ##(x,y)## satisfying the original equation, even though the polar parametrization was defined for all values of ##t##. Squaring both sides of the equation corrects this problem for the most part.
The line ##y=0## intersects the curve at ##x=0##. On the other hand, the line ##y=mx##, where ##m\neq0##, intersects the curve at exactly two non-zero points. This implies that the curve is not defined at the origin.
So the questions:
(1) Why is the rectangular representation of the curve not 1-1 w.r.t. the polar representation?
(2) Why does the algebra suggest that any line with non-zero slope does not intersect the curve at the origin?
- Relevant Equations
- ##x=r\cos t##
##y=r\sin t##
The curve ##\sqrt{x^2+y^2}=(\frac{y}{x})## is not defined for points ##(x,y)## in the second and fourth quadrants.
Consider the transformed curve ##x^2+y^2=(\frac{y}{x})^2##.
If ##y = 0##, then ##x^2+0^2=\frac{0^2}{x^2}=0##, which means that ##x=0##.
Along the line ##y=mx##, where ##m\neq0##, ##x^2(m^2+1)=m^2## is not defined if ##x=0##. This equation also suggests that the line intersects the curve at exactly two points.
I thought of something that suggests that the curve might not be defined at the origin. In the parametrization ##r=\tan t=\frac{r\sin t}{r\cos t}##, I think that it is implicitly assumed that ##r\neq0##. But plugging in ##t=0## contradicts this.
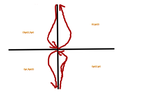
You can find a rough sketch of the curve attached to this post.
Consider the transformed curve ##x^2+y^2=(\frac{y}{x})^2##.
If ##y = 0##, then ##x^2+0^2=\frac{0^2}{x^2}=0##, which means that ##x=0##.
Along the line ##y=mx##, where ##m\neq0##, ##x^2(m^2+1)=m^2## is not defined if ##x=0##. This equation also suggests that the line intersects the curve at exactly two points.
I thought of something that suggests that the curve might not be defined at the origin. In the parametrization ##r=\tan t=\frac{r\sin t}{r\cos t}##, I think that it is implicitly assumed that ##r\neq0##. But plugging in ##t=0## contradicts this.
You can find a rough sketch of the curve attached to this post.
Attachments
Last edited: