- #1
highschoolstudent454
- 4
- 0
Is this wrong?
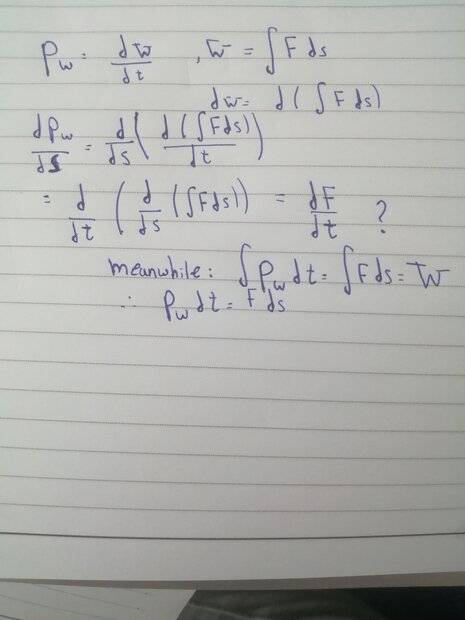
For a varying force applied at constant speed, yes.highschoolstudent454 said:Is the time derivate of force equal to the position derivative power?
The time derivative of force refers to how the force acting on an object changes with respect to time. Mathematically, it can be expressed as dF/dt, where F is the force. This derivative is related to the rate of change of momentum, as force is defined as the rate of change of momentum with respect to time (F = dp/dt).
The position derivative of power refers to how power changes with respect to position. Power is defined as the rate at which work is done, and it can be expressed as P = F · v, where F is force and v is velocity. The position derivative of power would involve taking the derivative of power with respect to position, which can provide insights into how power output varies as an object moves through space.
No, the time derivative of force is not equal to the position derivative of power. The time derivative of force relates to the change of force over time, while the position derivative of power relates to the change of power with respect to position. These are different concepts and involve different physical quantities and relationships.
The relationship between force, power, and their derivatives highlights the interconnectedness of these physical concepts. Understanding how force changes over time can provide insights into the dynamics of a system, while analyzing power in relation to position can help in understanding energy transfer and efficiency in mechanical systems.
To calculate the time derivative of force, you would need to measure how force varies with time and apply the derivative dF/dt. For the position derivative of power, you would first calculate power using P = F · v and then take the derivative of that expression with respect to position, using the chain rule if necessary. Both calculations require knowledge of the forces and motions involved in the specific system being analyzed.