bbq_build
- 62
- 2
I see. So, what is the purpose of measuring the resistance by stalling the motor shaft while measuring the voltage across the motor and the current in series with the motor? I am a bit confused now.
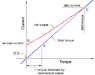
In my previous post, I mentioned that based on the torque vs current curve from the company, 0 kgcm at 1.2A and 135 kgcm at 17.5A. After unit conversion, 0 Nm at 1.2A and 13.24Nm at 17.5A. Since Torque = Kt * I, Kt = 13.24Nm/17.5 = 0.76. Since Ke = Kt and they are not affected by the input voltage, then I can use Kt = Ke = 0.76 in my Simulink model. Is this the right thing to do? From the data given by the company, their Kt =Ke= 3.55 which is quite different from my calculated Kt=Ke=0.76. Another confusion.
In my previous post, I mentioned that based on the torque vs current curve from the company, 0 kgcm at 1.2A and 135 kgcm at 17.5A. After unit conversion, 0 Nm at 1.2A and 13.24Nm at 17.5A. Since Torque = Kt * I, Kt = 13.24Nm/17.5 = 0.76. Since Ke = Kt and they are not affected by the input voltage, then I can use Kt = Ke = 0.76 in my Simulink model. Is this the right thing to do? From the data given by the company, their Kt =Ke= 3.55 which is quite different from my calculated Kt=Ke=0.76. Another confusion.