I Is the uncertainty principle applicable to single slit diffraction?

[greypilgrim]

Hi.

I've seen single slit diffraction being brought up as an example of the uncertainty principle: Narrowing the slit restricts the particles more in one dimension, which means the momentum in this dimension is more uncertain, which results in a more spread-out diffraction pattern.

I've even seen "derivations" of the uncertainty relation like the following:

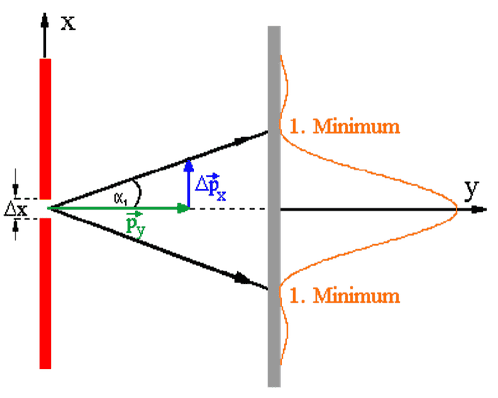
They use the 1st minimum of the pattern to define $\Delta\vec{p}_x$, and then with $\sin(\alpha_1)\approx\tan(\alpha_1)$ and the de Broglie wavelength successfully arrive at $\Delta x\cdot\Delta p_x\approx h$.

Well just taking the 1st minimum seems arbitrary. But if I'm not mistaken, a correct derivation of $\Delta p_x$ diverges since $x^2\sinc^2 (x)$ isn't integrable. This of course doesn't contradict the uncertainty principle, but is there a more rigorous way to make sense of it in the case of the single slit?

It's kind of weird that this "spreading out" of the pattern while narrowing the slit isn't reflected in $\Delta p_x$ at all which is always infinite.

 

Advertisement

[BvU]

Hi,

Not a real answer, but:

The colleagues have a thread on your subject.

There is also Sheet 24 here

$\$

 

[PeroK]

This of course doesn't contradict the uncertainty principle, but is there a more rigorous way to make sense of it in the case of the single slit?

It's kind of weird that this "spreading out" of the pattern while narrowing the slit isn't reflected in $\Delta p_x$ at all which is always infinite.

The uncertainty principle is a quick way to justify diffraction, but it doesn't explain the detail. A more detailed explanation is:

When the particle reaches the slit it has effectively the uniform wavefunction of a plain wave. The slit acts like an infinite square well and the wavefunction transforms to a linear combination of momentum eigenstates appropriate to the width of the well. When it emerges from the well, that superposition evolves as a superposition of free particle states. The width of the central band corresponds to the ground state of the well. The narrower the slit, the wider the band. The other bands correspond to the excited states. For a wider slit only the ground state is significant. For a narrow slit, more of the excited energy states become significant.

That's still some way short of a full mathematical treatment. But, it explains more than simply invoking the UP.

 

Advertisement