- #36
Is the Work Done by a Variable Force Non-Conservative?
- Thread starter MatinSAR
- Start date
In summary: Yeah, you replace it with whatever variable you want.the equation of path is x=(2a) sinθ and y=(a) cosθWhat is ##\theta## here? It can't be the argument parameter of the polar representation of (x,y) since we would deduce ##\tan^2(\theta)=\frac 12##. So I assume it is an arbitrary parameter unrelated to polar coordinates. But then, where do the integration bounds come from?I see no diagram.
- #37
MatinSAR
- 606
- 183
What about Theta ?! Did I specify theta correctly?haruspex said:
I get the factor 6 again ! Let me send a picture ...
- #38
- #39
erobz
Gold Member
- 3,893
- 1,662
I'm getting what you are getting.
$$ \int 3 x y^2 dy = \int 3 \cdot y^2 \cdot 2 \sqrt{a^2 - y^2} dy $$
Let:
##\frac{y}{a} = \sin \beta##
## \frac{ \sqrt{a^2 - y^2} }{a} = \cos \beta##
It follows that
$$ y^2 = a^2 \sin^2 \beta$$
$$\sqrt{a^2 - y^2} = a \cos \beta$$
$$ dy = a \cos \beta d \beta$$
$$ \implies \int 3 x y^2 dy = 6 a^4 \int \sin^2 \beta \cos^2 \beta d \beta $$
$$ \int 3 x y^2 dy = \int 3 \cdot y^2 \cdot 2 \sqrt{a^2 - y^2} dy $$
Let:
##\frac{y}{a} = \sin \beta##
## \frac{ \sqrt{a^2 - y^2} }{a} = \cos \beta##
It follows that
$$ y^2 = a^2 \sin^2 \beta$$
$$\sqrt{a^2 - y^2} = a \cos \beta$$
$$ dy = a \cos \beta d \beta$$
$$ \implies \int 3 x y^2 dy = 6 a^4 \int \sin^2 \beta \cos^2 \beta d \beta $$
Last edited:
- #40
- 23,583
- 5,819
I also get a factor of 6, although I would never have done the math the way that he did it>haruspex said:
- #41
MatinSAR
- 606
- 183
Was it true ?!Chestermiller said:
What was wrong with the way I have done the math
erobz said:
Can you please tell me what is your final answer ?!
- #42
erobz
Gold Member
- 3,893
- 1,662
I didn't do the integral!MatinSAR said:Was it true ?!
What was wrong with the way I have done the math
Can you please tell me what is your final answer ?!
- #43
erobz
Gold Member
- 3,893
- 1,662
Post your work, it will get checked by someone...
- #44
- 42,084
- 10,187
Yes, my mistake… I should have waited until I had the time to check properly.MatinSAR said:
- #45
MatinSAR
- 606
- 183
erobz said:
- #46
- 42,084
- 10,187
It is a line integral, not a double integral, so you must arrange that there is only one variable in the integrand. If you want to do it as an integral wrt y then you must first replace all the occurrences of x with what x is as a function of y.MatinSAR said:
But really, the parametric approach using theta is much easier.
- #47
erobz
Gold Member
- 3,893
- 1,662
Show the work. Explain how you get the last result?MatinSAR said:
- #48
MatinSAR
- 606
- 183
So my answer is wrong , isn't it ?!haruspex said:
Thank you ... I will try to do it using parametric approach and I will send a picture of the work ...
Thank you ... I will try to do it using parametrich approach and I will send a picture of the work ...erobz said:
- #49
- 42,084
- 10,187
I'm confused. Isn’t that what you already did in post #32? Except that you did not perform the integral.MatinSAR said:
- #50
- 23,583
- 5,819
The integration is very simple. It just involves the use of trigonometric identities.$$(\sin{\theta}\cos{\theta})^2=\frac{\sin^2{2\theta}}{4}=\frac{1-\cos{4\theta}}{8}$$
Last edited:
- #51
Steve4Physics
Homework Helper
Gold Member
- 2,421
- 2,252
Hi @MatinSAR. Can I point out a fundamental mistake you are making? I don’t think anyone has mentioned it yet (apologies if they already have).MatinSAR said:
The final expression for W can not contain x or y (or θ). These are variables which change as you move along the path. They must disappear when the definite integral is evaluated.
You made the same mistake in your Post #1 solution, where your final expression for W contained x and y.
The expression for W can contains only given constants (here only the 'a') and pure numbers like 5, π and √2 (just picking random values as examples).
For example, if you determine the formula for the circumference of a circle, x²+y²=a², it wouldn’t make sense to have x and/or y in the final formula.
- #52
MatinSAR
- 606
- 183
Yes. It was unfinished.haruspex said:
Thank you.Chestermiller said:
Thank you ... I didn't know about this.Steve4Physics said:
- #53
MatinSAR
- 606
- 183
I hope it's finally true ...
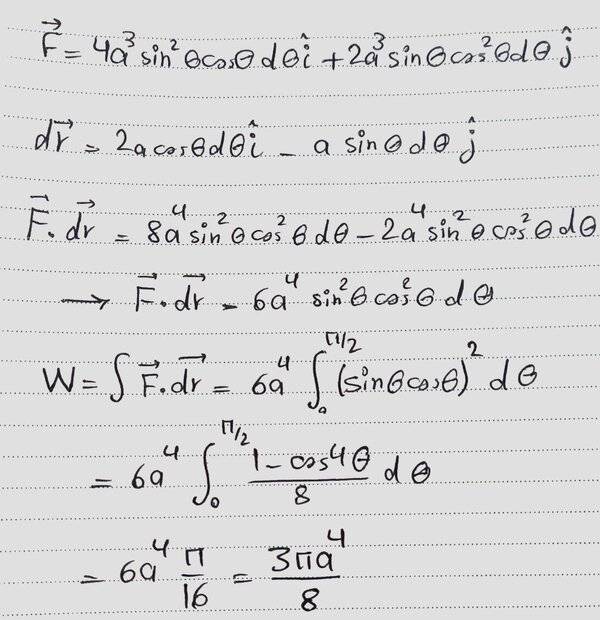The force is conserative so If I choose another path the final answer shouldn't change, Is it true ?!
- #54
- 42,084
- 10,187
Is it? What do you get if you integrate from 0 to 2π?MatinSAR said:
I agree with your answer.
- #55
MatinSAR
- 606
- 183
It was mentioned in question.haruspex said:
Thank you.haruspex said:
- #56
MatinSAR
- 606
- 183
haruspex said:
Steve4Physics said:
Chestermiller said:
erobz said:
kuruman said:
I hope I haven't forgotten anyone.Mister T said:
Thank you for your help and time.
- #57
- 42,084
- 10,187
Try the path along the axes. Isn't F always zero there?MatinSAR said:
- #58
- #59
Steve4Physics
Homework Helper
Gold Member
- 2,421
- 2,252
Oh yes it is! (If you familiar with British pantomime.)MatinSAR said:
Can I expand on what @haruspex said in Post #57?
Referring to the Post #21 diagram, suppose you take the following route:
- from A(0,a), move along the y-axis to the origin (0,0);
- from the origin (0,0) move along the x-axis to C(2a,0).
##\vec F = x^2y~\hat i + xy^2 \hat~j##
While moving along the y-axis (x=0); both components of ##\vec F## are zero.
Similarly while moving along the x-axis.
The total work done along this path is therefore zero. This is different to your calculated value for the original route from A to C.
So you can see (without doing any maths) that the force is not conservative.
- #60
MatinSAR
- 606
- 183
Great !Steve4Physics said:
So it's not conserative ...
Thank you.