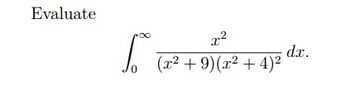- #1
nacho-man
- 171
- 0
This problem came up in worksheet for a real analysis class, so I can only assume they want us to use a method from real analysis.
It would be pretty rigorous to solve otherwise, could anyone point me in the correct direction?
Or do they simply want us to test our differentiating skills?
It would be pretty rigorous to solve otherwise, could anyone point me in the correct direction?
Or do they simply want us to test our differentiating skills?