- #1
hiyok
- 109
- 0
hi,
I'm studying a system using self-consistent mean field theory. And I have obtained its free energy, which however looks a bit unusual in that it bears a steep (almost abrupt) rise across the phase transition point (see the figure). This feature brought about fierce questioning from my boss. He said a free energy should not have such steep rise. But I think it is possible: around the transition point, the order parameter decreases very fast, and hence the potential energy, which is proportional to the square of the order parameter, should increase fast accordingly.
How do you think, my friends ?
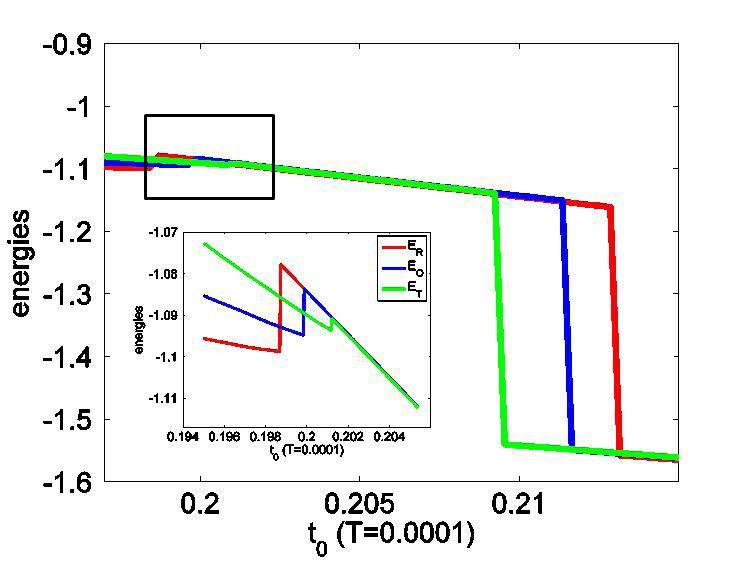
I'm studying a system using self-consistent mean field theory. And I have obtained its free energy, which however looks a bit unusual in that it bears a steep (almost abrupt) rise across the phase transition point (see the figure). This feature brought about fierce questioning from my boss. He said a free energy should not have such steep rise. But I think it is possible: around the transition point, the order parameter decreases very fast, and hence the potential energy, which is proportional to the square of the order parameter, should increase fast accordingly.
How do you think, my friends ?