- #1
Ameen1985
- 12
- 0
Hi all,
For an Isothermal compression process of air in a vessel with constant volume, I found the following expressions
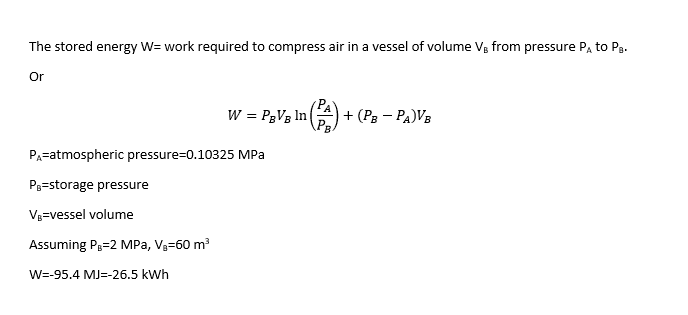
and
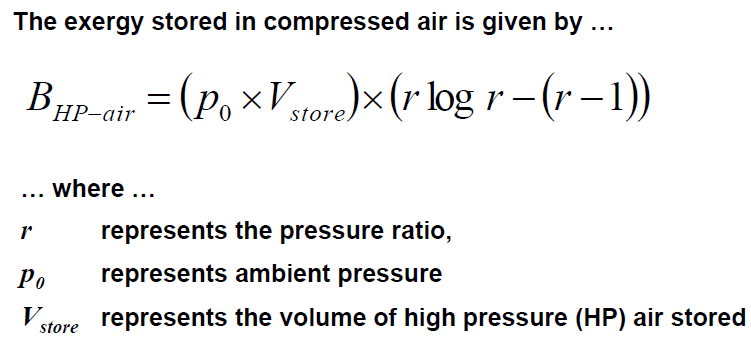
and
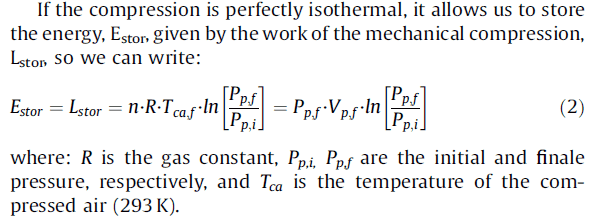
The first two give the same result, meanwhile the third gives another solution and I don't know why.
For adiabatic compression I found these two expression which also give different answers. Can someone please help me with this.
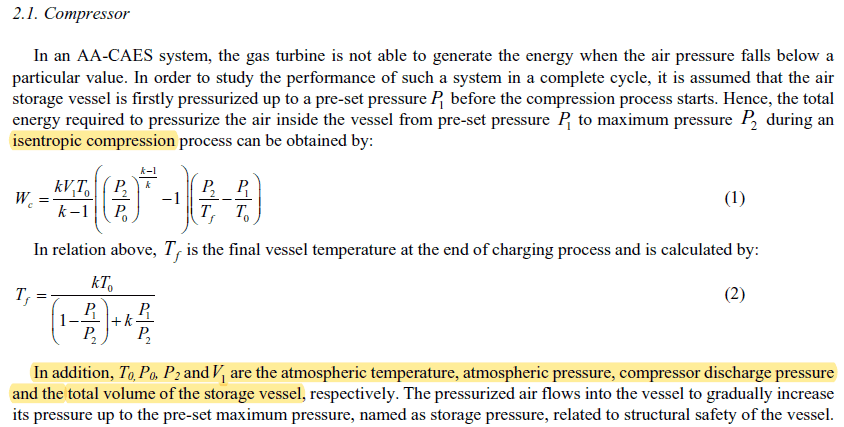
and
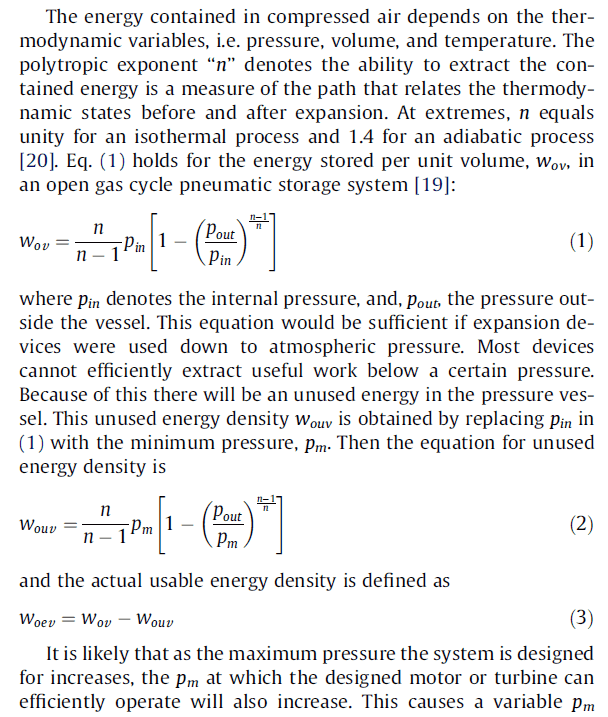 Let's say we have a 11 kWh to be stored in compressed air, P0 = 101325 Pa, P1 = 6.3 bar, P2 = 10 bar, and T0= 298 K. What would be the required air vessel volume?
Let's say we have a 11 kWh to be stored in compressed air, P0 = 101325 Pa, P1 = 6.3 bar, P2 = 10 bar, and T0= 298 K. What would be the required air vessel volume?
For an Isothermal compression process of air in a vessel with constant volume, I found the following expressions
and
and
The first two give the same result, meanwhile the third gives another solution and I don't know why.
For adiabatic compression I found these two expression which also give different answers. Can someone please help me with this.
and