MHB Kaska's question at Yahoo Answers involving related rates

[MarkFL]

Here is the question:

Calculus related rates question, Help please!?

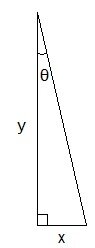
A lighthouse is fixed 170 feet from a straight shoreline. A spotlight revolves at a rate of 12 revolutions per minute, (24 rad/min ), shining a spot along the shoreline as it spins. At what rate is the spot moving when it is along the shoreline 11 feet from the shoreline point closest to the lighthouse?

I got the answer: 4080π(sec(0.0646)^2) but it seems to be wrong, I don't understand what I am doing wrong, any help?

Here is a link to the question:

Calculus related rates question, Help please!? - Yahoo! Answers

I have posted a link there to this topic so the OP can find my response.

 

[MarkFL]

Hello Kaska,

Let's generalize a bit and derive a formula we can then plug our data into.

The first thing I would do is draw a diagram:

View attachment 618

As we can see, we may state:

$\displaystyle \tan(\theta)=\frac{x}{y}$

Now, let's differentiate with respect to time $t$, bearing in mind that while $x$ is a function of $t$, $y$ is a constant.

$\displaystyle \sec^2(\theta)\cdot\frac{d\theta}{dt}=\frac{1}{y} \cdot\frac{dx}{dt}$

Since we are being asked to find the speed of the spot, whose position is $x$, we want to solve for $\dfrac{dx}{dt}$:

$\displaystyle \frac{dx}{dt}=y\sec^2(\theta) \cdot\frac{d\theta}{dt}$

Let's let the angular velocity be given by:

$\omega=\dfrac{d\theta}{dt}$

and from the diagram and the Pythagorean theorem, we find:

$\displaystyle \sec^2(\theta)=\frac{x^2+y^2}{y^2}$

Hence, we have:

$\displaystyle \frac{dx}{dt}=\frac{\omega}{y}(y^2+x^2)$

Now we may plug in the given data:

$\displaystyle \omega=24\pi\frac{1}{\text{min}},\,y=170\text{ ft},\,x=11\text{ ft}$

$\displaystyle \frac{dx}{dt}=\frac{24\pi}{170}(170^2+11^2)\,\frac{\text{ft}}{\text{min}}=\frac{348252\pi}{85}\, \frac{\text{ft}}{\text{min}}$

This is equivalent to the answer you obtained (accounting for rounding), however we have done away with the need to use a trig. function.