MHB Katlynsbirds' question at Yahoo Answers regarding inverse trigonometric identity

[MarkFL]

Here is the question:

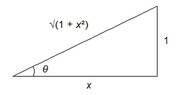
Prove the identity, pre calc!?

cot inverse= sin inverse of 1/sqr of 1+x^2

Here is a link to the question:

Prove the identity, pre calc!? - Yahoo! Answers

I have posted a link there to this topic so the OP can find my response.

 

[MarkFL]

Re: katlynsbirds' question at Yahoo! Answers regarding inverse trignometric identity

Hello katlynsbirds,

We are given to prove:

$$\cot^{-1}(x)=\sin^{-1}\left(\frac{1}{\sqrt{1+x^2}} \right)$$

Let's let $$\theta=\cot^{-1}(x)\,\therefore\,x=\cot(\theta)$$, and now please refer to this diagram:

https://www.physicsforums.com/attachments/765._xfImport

We see that $$\cot(\theta)=\frac{x}{1}=x$$ and we can also see that:

$$\sin(\theta)=\frac{1}{\sqrt{1+x^2}}\,\therefore\, \theta=\sin^{-1}\left(\frac{1}{\sqrt{1+x^2}} \right)$$

and so we may conclude:

$$\theta=\cot^{-1}(x)=\sin^{-1}\left(\frac{1}{\sqrt{1+x^2}} \right)$$

Shown as desired.

To katlynsbirds and any other guests viewing this topic I invite and encourage you to post other trigonometry problems here in our http://www.mathhelpboards.com/f12/ forum.

Best Regards,

Mark.