jeremyfiennes
- 323
- 17
A very basic and simple query, but I can't see my way through it.
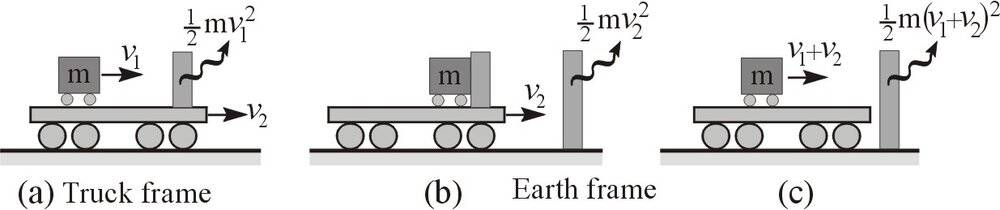
A mass m moves at speed v1 relative to a truck traveling at speed v2 , fig.a. All components except this mass are massless.
In a truck-stationary frame, the mass collides with a barrier on the truck liberating kinetic energy 1/2.mv1^2.
Switching to an Earth-stationary frame, the mass+truck continue at v2 and collide with an Earth-fixed barrier liberating a further kinetic energy 1/2.mv2^2, fig.b.
Had, however, the mass from the start gone straight on to collide with the Earth-fixed barrier, fig.c, it would have liberated kinetic energy 1/2.m(v1+v2)^2.
How can energy apparently vanish on switching frames?
A mass m moves at speed v1 relative to a truck traveling at speed v2 , fig.a. All components except this mass are massless.
In a truck-stationary frame, the mass collides with a barrier on the truck liberating kinetic energy 1/2.mv1^2.
Switching to an Earth-stationary frame, the mass+truck continue at v2 and collide with an Earth-fixed barrier liberating a further kinetic energy 1/2.mv2^2, fig.b.
Had, however, the mass from the start gone straight on to collide with the Earth-fixed barrier, fig.c, it would have liberated kinetic energy 1/2.m(v1+v2)^2.
How can energy apparently vanish on switching frames?