haha0p1
- 46
- 9
- Homework Statement
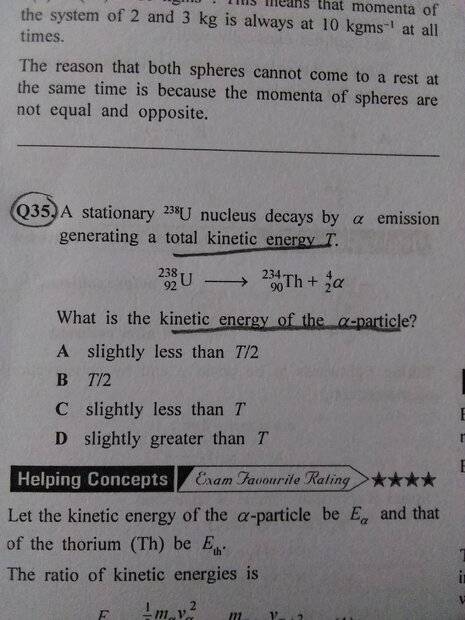
- A stationary U-238 nucleus decays be alpha emission generating a total kinetic energy T. What is the kinetic energy of alpha particle?
- Relevant Equations
- Ek=1/2mv²
Ek=Momentum²/2m
Kindly help me solve this question. The only thing so far that I know in this question is that energy is conserved and the momentum of Alpha particle will equal momentum of Thorium.