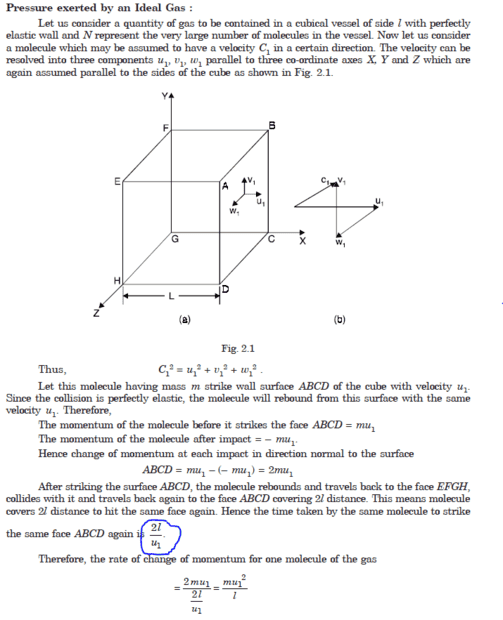
The discussion centers on the use of time between collisions rather than the time of collision when analyzing momentum change in molecules. It emphasizes that the average rate of momentum change for a molecule is calculated based on the time interval between its collisions with a wall, similar to how one calculates average income over a month. While introductory physics textbooks present this concept effectively, the erratic paths of molecules in a gas raise questions about the simplicity of this derivation. More advanced derivations can be found in statistical mechanics literature, suggesting a need for deeper analysis. Overall, the focus is on understanding momentum change in molecular dynamics through appropriate time intervals.