Paul159
- 15
- 4
I have a question about the Klein paradox in the massless case, for a potential step of height ##V_0## (this is exactly the situation described by Wikipedia). I don't have a problem to understand the "paradox", and I think the Wikipedia's illustration is quite telling.
My question is : what append at the limit case ##E_0 = V_0## ? The "wave function" after the step is constant (##k=0##), and the Dirac equation for the spinor's components ##\Psi_1##, ##\Psi_2## become ##0 = 0##... Thus there are no conditions for the value of those components. If I choose ##\Psi_1 = \Psi_2 = 1## for example, I still get ##T=1##. If I choose ##\Psi_1 = -\Psi_2 = 1##, I get ##R=1##. I "understand" this with the fact that at the node the group velocity is not defined.
So what would really happen in real life ?
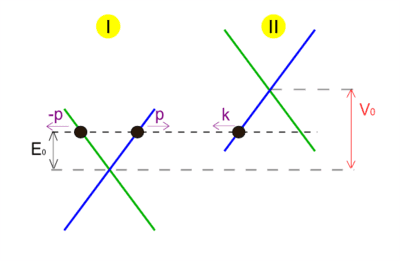
My question is : what append at the limit case ##E_0 = V_0## ? The "wave function" after the step is constant (##k=0##), and the Dirac equation for the spinor's components ##\Psi_1##, ##\Psi_2## become ##0 = 0##... Thus there are no conditions for the value of those components. If I choose ##\Psi_1 = \Psi_2 = 1## for example, I still get ##T=1##. If I choose ##\Psi_1 = -\Psi_2 = 1##, I get ##R=1##. I "understand" this with the fact that at the node the group velocity is not defined.
So what would really happen in real life ?