Kronecker Delta and Gradient Operator

[maxhersch]

I am looking at an explanation of the gradient operator acting on a scalar function $\phi$. This is what is written:

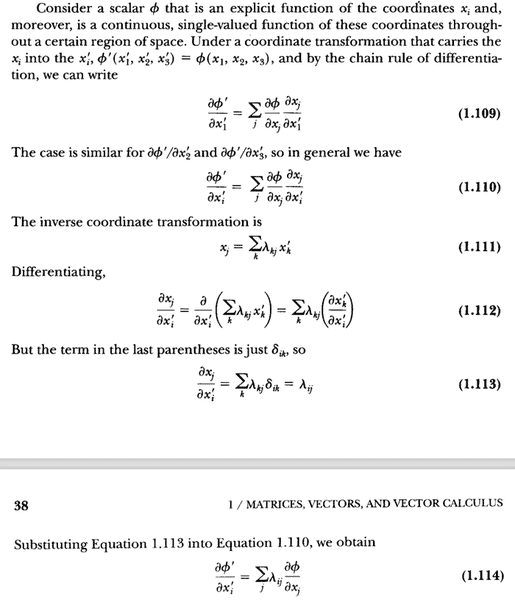
In the steps 1.112 and 1.113 it is written that $\frac {\partial x'_k} {\partial x'_i}$ is equivalent to the Kronecker delta. It makes sense to me that if i=k, then the expression is equal to 1 but why would it be 0 if they are not equal? Perhaps I'm not looking at it the right way but any explanation would be appreciated.

Thanks.

 

[ShayanJ]

Just think about Cartesian coordinates. What is $\frac{\partial x}{\partial y}$ equal to?