Lagrangian for a rod pivoting at the end of a clock hand

[member 731016]

Homework Statement

Please see belwo

Relevant Equations

$ml^2\ddot \phi + mRl\varphi \dot \phi\sin(\phi - \varphi t) = 0$

For (d),

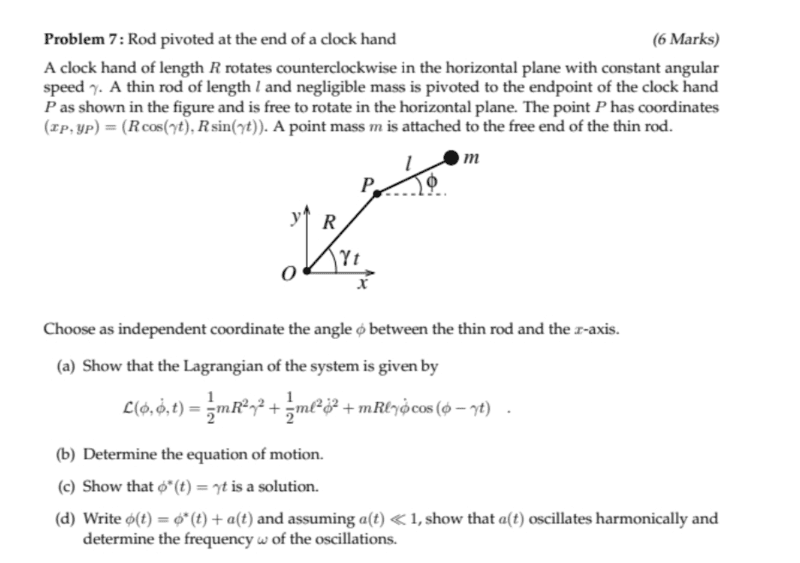
I am confused how $a(t)$ oscillates harmonically. The EOM is $ml^2\ddot \phi + mRl\varphi \dot \phi\sin(\phi - \varphi t) = 0$

Then using $\phi - \varphi t = a(t)$
$l\ddot \phi + R\phi \varphi a(t) = 0$
Where I used the small angle approximation after substituting in $a(t)$
Does anybody please know where to go from here to find $\omega$?

Thanks!

 

[Orodruin]

Simply linearise the equation of motion around $\phi^*$. You have attempted this but need to double check your math.

Also note: It is $\gamma$, not $\varphi$.

 

[member 731016]

Simply linearise the equation of motion around $\phi^*$. You have attempted this but need to double check your math.

Also note: It is $\gamma$, not $\varphi$.

Thank you for your reply @Orodruin!

$l^2\ddot \phi + Rl\phi \gamma a(t) = 0$ is that please correct now?

Sorry I don't understand how you linearize the equation of motion around $\phi^*$. I have not been taught that. Could you please explain a bit more?

Thanks!

 

[Orodruin]

Thank you for your reply @Orodruin!

$l^2\ddot \phi + Rl\phi \gamma a(t) = 0$ is that please correct now?

No. Carefully consider the second term. Where did the $\phi$ come from?

Sorry I don't understand how you linearize the equation of motion around $\phi^*$. I have not been taught that. Could you please explain a bit more?

It is essentially what you are (attempting) to do. You write $\phi = \phi^* + a(t)$ and then assume that $a(t)$ is small. Perform a Taylor expansion in $a(t)$ and keep only linear terms.

 

[Orodruin]

Also, for the first term, you have not inserted the assumption $\phi = \phi^* + a$ ...

 

[member 731016]

I have solved the problem now.

EOM: $l^2 \ddot a + Rl \gamma \dot \phi a = 0$

Angular frequency $\omega = \sqrt{\frac{R \gamma \dot \phi}{l}}$

Thanks!