- #1
mille2eo
- 2
- 0
1.
Problem Statement:
For the regular solution model, develop the equations for the compositions of the coexisting phases in a binary system and plot the phase boundary as a function of χ/RT.2. This question stems from Sandler's Introduction to Applied Statistical Thermodynamics.
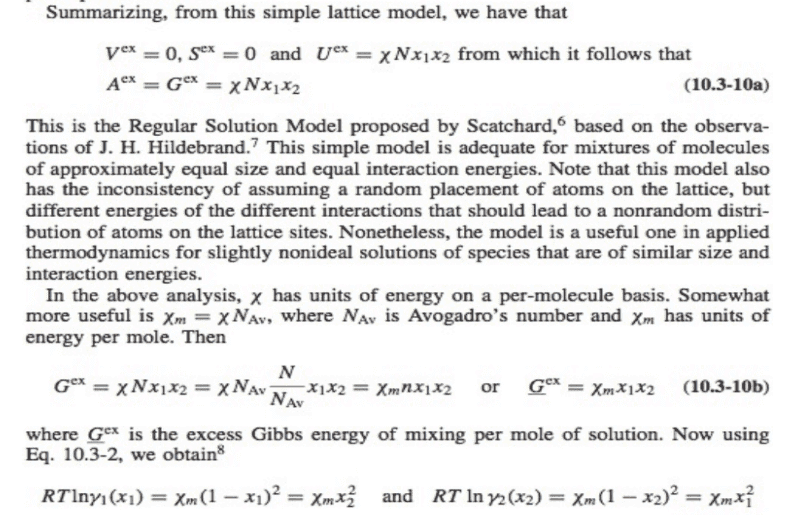
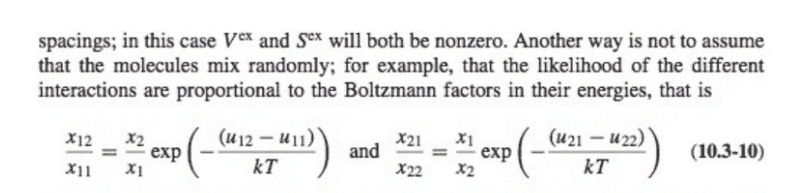
I am having trouble understanding how to approach this problem. I know that the equations I need to develop are a function of x1, x2 -> (1-x1). I do not know where to start when considering an equation for a phase boundary.
Any help would be appreciated. [/B]
Problem Statement:
For the regular solution model, develop the equations for the compositions of the coexisting phases in a binary system and plot the phase boundary as a function of χ/RT.2. This question stems from Sandler's Introduction to Applied Statistical Thermodynamics.
The Attempt at a Solution
I am having trouble understanding how to approach this problem. I know that the equations I need to develop are a function of x1, x2 -> (1-x1). I do not know where to start when considering an equation for a phase boundary.
Any help would be appreciated. [/B]