Law of Cosines: Solve for angles given the sides of triangle

[Elissa89]

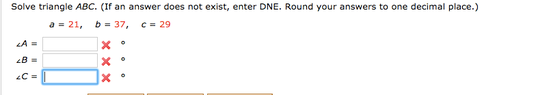
I've attached the problem and my work. When I enter cos^1(6.890625) I get an error, but 6.9 is also not the answer and Does Not Exist is also not an acceptable answer. So where I am going wrong with this?

View attachment 8436

View attachment 8437

 

[MarkFL]

Your image is sideways and hard to read.

Okay, if I am trying to find $$\angle A$$, I would begin with the fact that this angle is opposite side \(c\) and write:

$$c^2=a^2+b^2-2ab\cos(A)$$

$$\cos(A)=\frac{a^2+b^2-c^2}{2ab}$$

$$A=\arccos\left(\frac{a^2+b^2-c^2}{2ab}\right)\approx51.4^{\circ}$$

Can you proceed in like manner to find the other two angles?

 

[Elissa89]

Your image is sideways and hard to read.

Okay, if I am trying to find $$\angle A$$, I would begin with the fact that this angle is opposite side \(c\) and write:

$$c^2=a^2+b^2-2ab\cos(A)$$

$$\cos(A)=\frac{a^2+b^2-c^2}{2ab}$$

$$A=\arccos\left(\frac{a^2+b^2-c^2}{2ab}\right)\approx51.4^{\circ}$$

Can you proceed in like manner to find the other two angles?

Answer wasn't correct for angle A but it was correct for angle c. Sorry it was sideways, that was just how it uploaded. Still not sure why I can't find angle A though when a=21 is opposite of angle A.

 

[MarkFL]

Answer wasn't correct for angle A but it was correct for angle c. Sorry it was sideways, that was just how it uploaded. Still not sure why I can't find angle A though when a=21 is opposite of angle A.

Okay, that's not standard practice, the way the angles and sides are labeled. But, given that it is labeled that way, then what I posted would indeed work for $$\angle C$$.

To find $$\angle A$$, we would then use:

$$A=\arccos\left(\frac{b^2+c^2-a^2}{2bc}\right)\approx34.5^{\circ}$$

 

[I like Serena]

Answer wasn't correct for angle A but it was correct for angle c. Sorry it was sideways, that was just how it uploaded. Still not sure why I can't find angle A though when a=21 is opposite of angle A.

Hi Elissa89,

Your initial formula was correct.
How is that not the standard practice MarkFL?

You have:
$$a^2=b^2+c^2-2bc\cos A\\
21^2=29^2+37^2-2(29)(37)\cos A \\
441=841+1369-2146\cos A $$
How did you get from there to:
$$\frac{441}{64}=\frac{(\cancel{64})\cos A}{\cancel{64}}$$
Because that is not correct.

 

[MarkFL]

...How is that not the standard practice MarkFL?...

From what I've always seen, triangles are labeled thusly:

 

[I like Serena]

From what I've always seen, triangles are labeled thusly:

That's indeed the correct triangle, although I'm used to having A at bottom left.
Either way, $a$ is opposite the angle $A$ as it should be.
And then the cosine rule is: $a^2=b^2+c^2 -2bc\cos A$.

 

[Olinguito]

The image itself isn’t sideways – I downloaded it and it was the right way up – for some reason it was rotated when attached in the post. I’ve uploaded it to Imgur and retrieved it from there instead:

View attachment 8440​

 

[MarkFL]

That's indeed the correct triangle, although I'm used to having A at bottom left.
Either way, $a$ is opposite the angle $A$ as it should be.
And then the cosine rule is: $a^2=b^2+c^2 -2bc\cos A$.

Okay, now that I cock my head to the side and squint my eyes, I see the triangle is labeled that way. Sorry for all the confusion. :(

 

[I like Serena]

The image itself isn’t sideways – I downloaded it and it was the right way up – for some reason it was rotated when attached in the post. I’ve uploaded it to Imgur and retrieved it from there instead:

​

That's odd.
After downloading I see a thumbnail that is sideways.
But when I open it in an image viewer it is indeed the right way up.
Never seen that before!

Anyway, I can see in my image viewer that the option Auto rotate according to EXIF info is checked.
And indeed, the EXIF info shows swapped dimensions.

Elissa89, if I may ask, how did you scale down the image?
I can see that the original size was much bigger.

 

[MarkFL]

Since I caused so much confusion, I wanted to redeem myself by posting a complete solution. Here is a diagram drawn to scale:

View attachment 8441

$$A=\arccos\left(\frac{b^2+c^2-a^2}{2bc}\right)\approx34.5^{\circ}$$

$$B=\arccos\left(\frac{a^2+c^2-b^2}{2ac}\right)\approx94.1^{\circ}$$

$$C=\arccos\left(\frac{a^2+b^2-c^2}{2ab}\right)\approx51.4^{\circ}$$