Leaf Spring Deflection: Calculating Force & Displacement

[FEAnalyst]

TL;DR Summary

How to calculate the deflection of a leaf spring?

Hi,

I'm trying to calculate the deflection of a leaf spring. I've found several formulas but the one I'm currently using looks like this: $$f=k \frac{F L^{3}}{6EI}$$ where: $k$ - coefficient depending on the way leaves change their shape in the width direction (in my case it's $1$ because leaves are not tapered if you look at them from below), $F$ - force, $L$ - length of the spring (between the centers of both eyes), $E$ - Young's modulus, $I$ - section moment of inertia. I calculated $I$ using a standard formula for rectangular section: $$I=\frac{bh^{3}}{12}$$ where: $b$ - width of the spring, $h$ - height of the whole section (sum of all layers' heights). My leaf spring is curved (initially bent) but I think that this formula should account for that. I also assume that a small mounting hole in the middle where a force is applied doesn't influence the calculations too much.

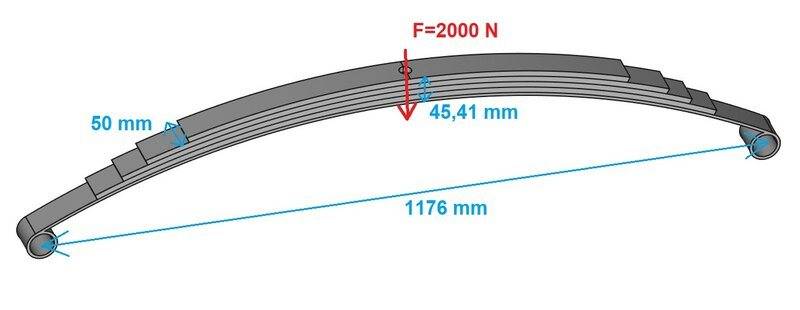
Anyway, here's what I get for the data included in the picture above: $$f=\frac{2000 \cdot 1176^{3}}{6 \cdot 210000 \cdot \frac{50 \cdot 45,41^{3}}{12}}=6,62 \ mm$$ According to finite element analysis, the deflection in the middle of the spring should be around $0,079 \ mm$... I checked all the inputs in both analytical and numerical calculation and I couldn't find any error. Do you know what can be wrong here ? I must have missed something or the formula is incorrect (I've found it in a Polish book about the design of cars).

 

[jrmichler]

How did you model the contact between leaves? Did you inadvertently model it such that the software is modeling it as one solid part with stepped thickness? Are the eyes free to spread out? If I was modeling a leaf spring, here's how I would do it:

Constrain the center holes to stay on a vertical line.
Constrain one side of the leaves to stay on a vertical surface.
Spring support on the bottom of the eyes with initial value 1,000,000 lbs/inch/inch^2 normal and zero tangential spring constant. You will need to delineate an area on the bottom of the eyes the width of the spring and 10 to 20 mm long. Adjust the area and spring constants if needed.
Delineate an area on the top leaf extending 1" or 2" on each side of the center hole and the width of the leaf. Apply the vertical force to that area.
Define a spring between each pair of leaves. The spring will be stiff in the normal direction and zero stiffness in the tangential direction. I would start with spring constant 1,000 lbs/inch/inch^2, then adjust as indicated.

I think that should do it. The leaves are constrained to stay aligned, the eyes can deflect outward, and the entire spring is constrained against spinning around or tipping over. This model is unrealistic in assuming zero friction between leaves, but adding friction would require modeling contact with friction. Such a model would require a high end FEA package and hours or days of run time vs minutes for the model I laid out.

 

[FEAnalyst]

Bonded (tied) contact is applied between the leaves so the surfaces can’t separate or slide on each other. Eyes are fixed in all directions but I also tried a case where they were free to rotate about their axes. The thing is that this model is supposed to be simple as it will only serve for educational purposes. I just want its results to agree with analytical solution which involves even more simplifications (for example, it doesn’t take into account the differences in leaf lenghts). It should be possible to obtain quite good agreement of this basic numerical model with analytical calculations. Hence, I think that there is a mistake somewhere that causes the large discrepancy in deflection results.

 

[jrmichler]

Bonded (tied) contact is applied between the leaves so the surfaces can’t separate or slide on each other. Eyes are fixed in all directions

That would explain the overly stiff FEA results. A quick ball park calculation:

Beam stiffness = (a constant that includes length) divided by EI.
Simplifying further: Stiffness is proportional to 1/I.
Rectangular cross section: $I = bh^3/12$
I is proportional to $h^3$.
Assume two leaf springs, each with five leaves, and all leaves identical dimensions.
One spring has the leaves sliding against each other. The stiffness is proportional to $5*h^3$.
The other spring has the leaves bonded to each other. The stiffness is proportional to $(5h)^3$.
The ratio of the two leaf spring stiffness is then: ${(5h)^3}/{(5*h^3)}~=~25$

The spring with bonded leaves is 25 times stiffer than an otherwise identical spring with leaves that slide against each other. Fixing the eyes instead of allowing them to slide outward further stiffens the spring.

These two differences can easily account for a stiffness error in the FEA model of about two orders of magnitude. I did not check your analytical equation, but it does not look right because there is nothing in it about the number of leaves or the length of each leaf.

For a sanity check on your results, typical automotive leaf springs have spring rates on the order of 100 to 200 lbs/inch.

 

[FEAnalyst]

Thank you. I can replace these bonded connections with contact (even frictional), no problem. I thought that the differences won’t be that big and that I shouldn’t add features that aren’t included in the analytical solution. But maybe it wasn’t the right approach. Another thing is that the force is applied via special constraint that makes the selected surfaces (walls of the mounting hole) rigid. But the hole is narrow and I don’t expect it to spoil the results. I may try to apply the load in a different way though.

When it comes to analytical formula, it’s the best one that I’ve found (it’s surprisingly hard to find reliable sources about leaf spring calculations) but I also think that it may be inaccurate. The number of leaves is taken into account in the second moment of area (here I used the global one but the original formula involves summation of second moments of area of all springs). However, as we both note, the lengths of individual springs are not considered in this analytical solution.

When it comes to boundary conditions in the eyes (also applied via this rigid constraint), I can think of two options:
- all degrees of freedom fixed
- rotations about their axes free
- the same but one eye has translation in the spring’s length direction free (roller support like in a simply-supported beam)

Which option would you choose ? Maybe some other variant that I didn’t mention ?

By the way, there are many research papers that present leaf spring analyses with bonded contact and other approximations and there’s no analytical verification. Now I see how incorrect their results probably are.