Leakage Flux Inside a Slot of an AC Machine (dual stator)

[BlackMelon]

Hello All,

Please see the attachments. The pdf file is the IEEE paper I'm reading. Figure 1 show a slot of the AC machine similar to the front view of the picture below:

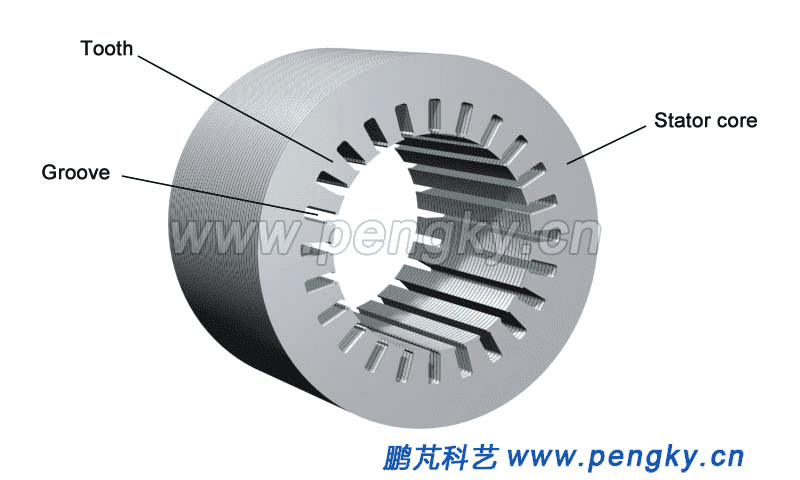
But in one slot, they fit two sets of conductors. The first set carries current i1 in total. The second set carries current i2. They study the leakage flux path just like in the figure 1. So, they apply the Ampere's law in (1) to find the magnetic field strength (hs(Y)) first. However, I am curious about the (3) and (4) equation. Why do we have to multiply dφs by nSi to get dφsi and then integrate it to get φsi in (4) ? Can we just integrate the (2) equation to get φsi?

Ps. To my understanding, please see the image file (drawn by MS paint). I align the x-axis and y-axis as those in the IEEE paper. But I add another dimension (z-axis) to clarify the constant "L". The number conductors was taken into account in the (1) equation already. L*dY is the area perpendicular to u0*hs(Y). So, multiply these two terms and integrate the product along the Y-axis should get the total flux for a certain area.

 

[Future Bruno]

The answer to your question is that when you integrate the dφs equation (2) to get φsi, you are actually integrating over the entire area of the slot. This is because when you integrate, you are summing up all the individual contributions from each current carrying conductor in the slot. The equation (3) and (4) are used to calculate the contribution from each individual conductor, which is why it is multiplied by the number of turns (nSi). By integrating this product, we can then get the total flux (φsi) in the slot.