How Does Lenz's Law Explain the Jumping Ring Phenomenon?

[sss1]

Homework Statement

Not a homework question, but not understanding how jumping ring works with AC current

Relevant Equations

NA

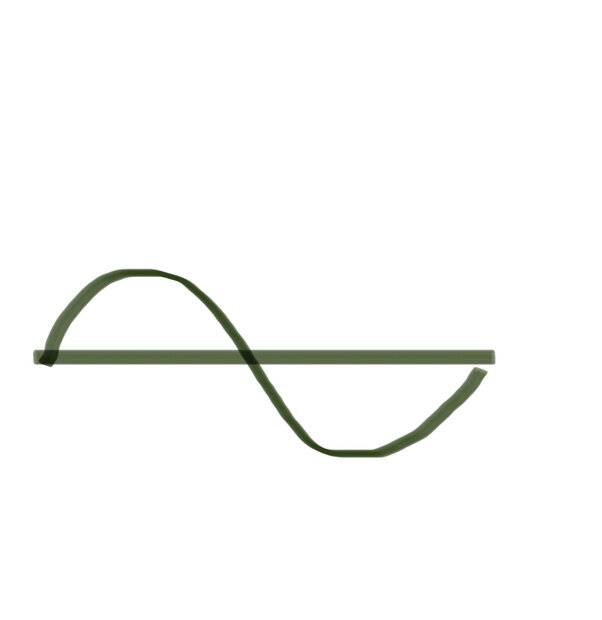
From what I understand, when the current is increasing(in the first quarter of the graph I drew), there is an increase in magnetic flux assuming that the current goes in the direction I drew in. That means the current induced in the ring is going to create a magnetic field trying to oppose that change, ie decrease the magnetic flux. So it’s going to point down, which means the two north poles of the two magnetic fields are facing each other, so the ring experiences a repelling force, so it goes up. But when the current is decreasing(in the second quarter of the graph I drew), there is now a decrease in magnetic flux, so the induced current in the ring is going to create a magnetic field such that it tries to increase the flux? But then that would mean the south and the North Pole of the magnetic fields are facing each other, so the ring will get attracted and not fly up anymore? Or is it because since now the ring is flying up, the decrease in flux is so small so that the attractive force becomes negligible?

 

[kuruman]

The following site has an informative video that (unfortunately) can be watched only at the original website as desired by the video owner (MIT). To go there, highlight the link past the first extraneous "h" from "hhttps" and then copy the link to your browser window.

Watch the video. Why do you think that there is such a maximum height difference between AC and DC voltage connected to the solenoid?

hhttps://www.youtube.com/watch?v=Pl7KyVIJ1iE

 

[TSny]

The time-averaged force on the ring for a complete oscillation of the B field is upward. This is due to a phase shift between the induced emf in the ring and the induced current in the ring.

The force on the ring at an instant of time is proportional to the product of the induced current $I(t)$ in the ring and the radial component of the magnetic field $B_r(t)$ of the solenoid’s magnetic field at the location of the ring material. Here, “radial” means perpendicular to the axis of the solenoid.

If the B-field varies as $\sin(\omega t)$, then the induced emf will vary as $\cos(\omega t)$. The induced current will vary as $\cos(\omega t - \varphi)$, where $\varphi$ is the phase “lag” of the induced current. The phase shift $\varphi$ of the current is the result of the ring having both resistance $R$ and inductive reactance $\omega L$. From basic AC theory, $\varphi = \tan^{-1}\left(\frac{\omega L}{R}\right)$.

So, the upward force on the ring is proportional to $\sin(\omega t)\cos(\omega t - \varphi)$. For part of a cycle of the B field, the force will be upward and for part of the cycle, the force will be downward. However, there is more upward force than downward force during a cycle. That is, the time average of this expression for a complete cycle of the B field is not zero.

If you hold the ring in place, you will feel the ring vibrate due to the oscillating upward and downward forces. But, you can feel that the average force is definitely upward. (You won't be able to hold the ring in place for long because the ring will heat up rapidly due to the large induced current in the ring.)

 

[kuruman]

This article has a lot of useful information plus experimental results about what's going on. It's a bit more involved than what meets the eye.

https://arxiv.org/pdf/1404.6024.pdf

 

[sss1]

The time-averaged force on the ring for a complete oscillation of the B field is upward. This is due to a phase shift between the induced emf in the ring and the induced current in the ring.

The force on the ring at an instant of time is proportional to the product of the induced current $I(t)$ in the ring and the radial component of the magnetic field $B_r(t)$ of the solenoid’s magnetic field at the location of the ring material. Here, “radial” means perpendicular to the axis of the solenoid.

If the B-field varies as $\sin(\omega t)$, then the induced emf will vary as $\cos(\omega t)$. The induced current will vary as $\cos(\omega t - \varphi)$, where $\varphi$ is the phase “lag” of the induced current. The phase shift $\varphi$ of the current is the result of the ring having both resistance $R$ and inductive reactance $\omega L$. From basic AC theory, $\varphi = \tan^{-1}\left(\frac{\omega L}{R}\right)$.

So, the upward force on the ring is proportional to $\sin(\omega t)\cos(\omega t - \varphi)$. For part of a cycle of the B field, the force will be upward and for part of the cycle, the force will be downward. However, there is more upward force than downward force during a cycle. That is, the time average of this expression for a complete cycle of the B field is not zero.

If you hold the ring in place, you will feel the ring vibrate due to the oscillating upward and downward forces. But, you can feel that the average force is definitely upward. (You won't be able to hold the ring in place for long because the ring will heat up rapidly due to the large induced current in the ring.)

OO! That makes more sense, so the ring does experience a downward force, but its magnitude is negligible compared to the amount of upward force?

 

[sss1]

This article has a lot of useful information plus experimental results about what's going on. It's a bit more involved than what meets the eye.

https://arxiv.org/pdf/1404.6024.pdf

Thanks! I'll have a look at it :)

 

[TSny]

OO! That makes more sense, so the ring does experience a downward force, but its magnitude is negligible compared to the amount of upward force?

I wouldn't say that the downward force is necessarily negligible. It depends on the amount of phase lag $\varphi$ of the induced current. Here are some plots for the force vs. time for one cycle of the solenoid's B field. Positive values of the force indicate upward force on the ring.

The first plot is for no phase shift ($\varphi = 0$). In this case, force averages to zero over one cycle. As $\varphi$ increases, the positive (upward) force dominates more and more. The vertical scales of these graphs are arbitrary. But the graphs do show the fraction of time that the force is upward in each case.

These graphs are for the case where the ring is held at a fixed height. When the ring is in vertical motion, there are other complicating factors as shown in the paper that @kuruman references. But, I think the main reason why the average force is upward is the phase shift $\varphi$.

 

[sss1]

Can you explain what is meant by current lag of the induced current? I didn't quite understand that bit.

 

[TSny]

Can you explain what is meant by current lag of the induced current? I didn't quite understand that bit.

The "current lag" is the phase shift of the induced current in the ring due to the self-inductance of the ring. It is given by the phase angle $\varphi$ in post #3. If the magnetic field of the solenoid varies with time as $B(t) \sim \sin(\omega t)$ then the induced current in the ring varies as $I(t) \sim \cos(\omega t - \varphi)$.

If there were no self-inductance, then $\varphi = 0$. The graphs of $B(t)$ and $I(t)$ for this case are shown below.

I've chosen positive directions for $B$ and $I$ such that the force on the ring is upward when $B$ and $I$ have the same sign and the force is downward when they have opposite signs. The vertical lines separate the regions of upward force and downward force. For the first quarter of a cycle, the force is upward, for the second quarter of a cycle it is downward, etc. This is the case you were describing in your first post. The average force for a complete cycle is zero.

However, $\varphi$ will not be zero due to the self-inductance of the ring. This causes the current in the ring to "lag" behind the case where $\varphi = 0$. For example, the graphs for $\varphi = 20^o$ are shown below

Note how the graph of the induced current is now shifted to the right a bit. This means the current is lagging behind what it was when $\varphi = 0$. The vertical lines still separate regions of upward and downward force on the ring. You can see that now the force is upward more than downward during a cycle.

The force on the ring is proportional to the product of $B(t)$ and $I(t)$: $F(t) \sim \sin(\omega t) \cos(\omega t -\varphi)$.
So, a plot of the force on the ring for $\varphi = 20^o$ for one cycle of the solenoid's magnetic field looks like

Positive force corresponds to upward force.