B Understanding Lewin's Circuit Paradox: Explained Simply and Clearly

[alan123hk]

I just saw the following clip on youtube



It seems that many people are still interested in this topic.
Here is the simple clear explanation I came up with, is there any error please ?

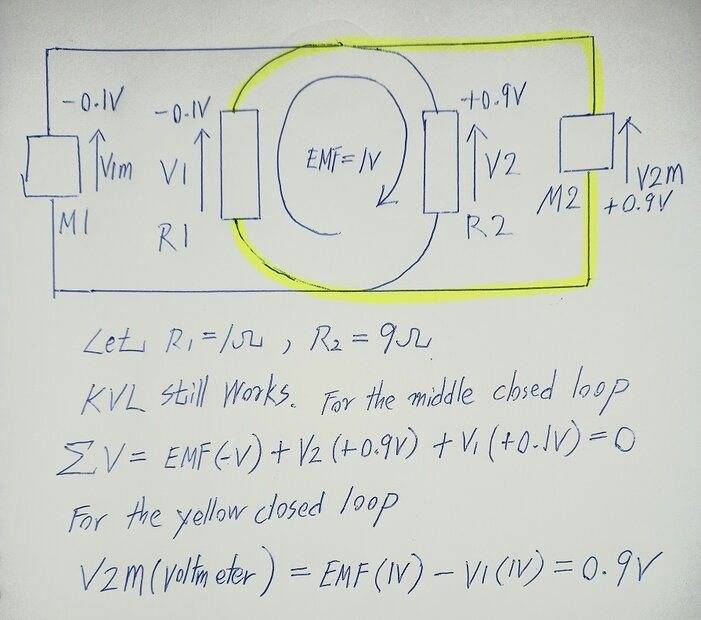
This means that voltmeter 2 is not measuring the potential difference produced by the charge accumulated on resistor R2, it is actually the voltage produced by the EMF minus the voltage drop across R1, so of course we cannot apply KVL to the outermost closed loop (including R1 and R2) and expect the sum of potential differences to be zero.
The crux of the matter is that KVL should not be applied in this way.

 

[alan123hk]

Sorry, it seems I haven't explained it thoroughly. I think kVL applies to all closed loops of the structure, be it the middle loop, the yellow loop and the outermost loop.

Middle loop and Outermost Loop, $\sum {V} = EMF(1V) + V1(-0.9)+V2(-0.1)=0$

Yellow loop, $\sum {V} = EMF(1V) + VM2(-0.9)+V1(-0.1)=0$

So my conclusion is, it's not that kVL can't be applied to this particular structural case, the question is how to apply it. For example to add EMF where appropriate.

 

[hutchphd]

So my conclusion is, it's not that kVL can't be applied to this particular structural case, the question is how to apply it.

With respect, this seems to me a distinction without a difference.

It strikes me that the guy from "RSD academy" is a perfect example of the old adage that "if the only tool you have is a hammer then everything looks like a nail". It is difficult to watch his contortions. It is not a nail.

 

[alan123hk]

a distinction without a difference

Dr. Lewin is an outstanding physicist, and I have great respect for him. In front of this giant, I'm insignificant, not as good as a kid, but he did say that kVL doesn't apply to this example involving induced electric fields, so how to explain it? So I can only think that my application method may be different from his application method. Or maybe my thoughts are different from his.

 

[vanhees71]

Kichhoff's Laws are just Faraday's Law (the EMF around a loop is the time derivative of the magnetic flux going through the enclosed surface) and charge conservation (the sum of all currents going into a node vanishes), i.e., very fundamental laws, and thus always apply.

Why there should be any paradox I never understood. The calculations in this thread look correct and demonstrate that there's no paradox. You must, of course, not call everything a voltage. That term should only be used for electrostatics, where the electric field has a potential. Here you have an electromotive force. I didn't watch the video nor Lewin's one, but Lewin is at least correct in this manuscript:

https://web.mit.edu/8.02/www/Spring02/lectures/lecsup4-1.pdf

Maybe he doesn't call the Eq. (4) "Kirchhoff's Law", but that's just semantics. I'd call it Kirchhoff's Law, and of course one has to take into account the EMF.

 

[hutchphd]

One explains it using Maxwell's equations (Faraday) because Kirchhoff (KVL) usually assumes ideal isolated wires and components and so does not apply when that is not true. Or one can make a horrible kluge to try to maintain the sanctity of KVL. Maxwell is "always" true, sometimes KVL is not because there are assumptions required. Prof Lewin is exactly correct.
I see @vanhees71 has preempted me!

 

[vanhees71]

Well, it depends on what you call "Kirchhoff's Laws". For me it's the integrated version of Faraday's Law
$$\dot{\Phi}_B[A]=\mathrm{d}_t \int_A \mathrm{d}^2 \vec{f} \cdot \vec{B} = - \mathcal{E}[\partial A]=-\int_{\partial A} \mathrm{d} \vec{r} \cdot (\vec{E}+\vec{v} \times \vec{B}),$$
where $\vec{v}$ is the velocity field along the boundary of the surface, $\partial A$. As I said, in the above quoted manuscript Lewin is fully correct. The important point indeed is that the magnetic flux is a functional of the surface $A$ and the EMF is a functional of the corresponding boundary $\partial A$.

 

[alan123hk]

After reading this https://web.mit.edu/8.02/www/Spring02/lectures/lecsup4-1.pdf, I very much agree.
Of course, if there is a time-varying magnetic field in the circuit, the potential difference between the two points will inevitably change with the difference of the electric field integration path, so the concept of potential difference no longer exists in theory.

Many times, when we discuss some individual examples, we assume that the magnetic field is completely confined to some fixed region, and that the magnetic field outside this range is zero. Therefore, it is considered that outside this range, the potential difference between two points does not vary with the integration path. However, this is practically impossible. Once the changing magnetic field is generated, it must spread everywhere in a closed loop. The question is only of strength and weakness.

But in real life, people are still used to refer to the output of the electromagnetic induction generator and the electromagnetic induction transformer as the potential difference.
While this may seem problematic in theory, in practice they do come close to a potential difference as it is rare to hear people complain about using very long wires to connect the output of these devices to the load and then finding that placing the wires affects the voltage output differently.

 

[vanhees71]

Indeed, and of course the standard laws of circuit theory work for transformers too! Whether you call them Kirchhoff's Laws or not, is a question of semantics only. If you correctly apply Maxwell's equations and the implied conservation of electric charge, you can't go wrong!

 

[hutchphd]

But in real life, people are still used to refer to the output of the electromagnetic induction generator and the electromagnetic induction transformer as the potential difference.

As @vanhees71 point out, the real issue here is understanding the model. The lumped-element model of electronic circuits makes the simplifying assumption that the attributes of the circuit ( resistance, capacitance, inductance, and gain) are concentrated into idealized electrical components which are resistors, capacitors, and inductors, etc. joined by a network of perfectly conducting wires. It is this model that fails and failure to understand that causes much confusion.

 

[Sam Gallagher]

I agree with the others, and would only add that I asked a question about this in PhysicsSE which lays out the ideas involved very carefully, so everyone can be on the same page about terminology and assumptions. The answer there is the same as here, it's not hard to "fix" KVL by including the induced term, but you can't assign it to a specific lumped element, so most people would say it's not strictly within the realm of circuit theory. I think the confusion that came out of Lewin's lecture is a great example of confusing tons of people with a question about semantics instead of teaching them something about physics. But at least it demonstrates how to analyze a model for where it breaks down, even if modifying the model is trivial

 

[hutchphd]

I think the confusion that came out of Lewin's lecture is a great example of confusing tons of people with a question about semantics instead of teaching them something about physics.

A farmer sells a mule. He says the mule is very obedient, and all you have to do is whisper in his ear. The buyer can't get the mule to do anything. He whispers, he yells, he cusses and smacks the mule but the mule won't move. He calls the farmer to complain.The farmer comes, picks up a 2X4, and hits the mule in the head. He gives the mule a simple command, and the mule obeys. "All you have to do is whisper in his ear," says the farmer."But first you have to get his attention."

For me most true learning is preceeded by confusion. So the fact that confusion was engendered by a lecture is not necessarilly a negative quality measure. I am a great admirer of Prof. Lewin's lecture style. The fact that many folks were clearly stuck on the dogma underscores this.

 

[Sam Gallagher]

There are two types of confusion in learning: (1) confusion arising from difficult concepts, clever proofs and derivations, unique applications of ideas, intuitive leaps required in problem solving, breaking down of assumptions, etc, and (2) confusion arising from imprecise definitions, lack of motivation, or incomplete explanations. The former is helpful, the latter should be considered a mortal sin for a teacher. This whole discussion should be a footnote about exactly what Lewin considers "KVL" to mean, with a mention of lumped elements as used in circuit theory (which I might add is arbitrary and needlessly limiting in cases just like this), and nothing more.

 

[hutchphd]

If you care his MIT lectures are availible everywhere..
They are availible because he has been reknowned as a lecturer for more than half a century. His bona fides do not need examination.
I would point out that confusion very often arises from misconceptions (for whatever reason) of simple ideas by the student, novice or advanced.

 

[DaveE]

The first line of Wikipedia says it all:
"Kirchhoff's circuit laws are two equalities that deal with the current and potential difference (commonly known as voltage) in the lumped element model of electrical circuits."

"lumped element model" - get it? it's that simple! There is less here than many people think*, it's no paradox. KVL is little more than a book keeping rule for EEs analysing schematics.

For this to make sense, all induced voltages must be represented somehow as a circuit element (inductors). Of course there are many examples where a lumped element model isn't a good representation of the real world. It which case you ignore KVL and use Maxwell's equations.

PS: * including Prof Lewin, I think. He makes a big deal about a simple misunderstanding. His video could be about 10 seconds: "You can't use KVL here, it's not applicable. Now, let's talk about induced voltages the right way..."

 

[vanhees71]

If you care his MIT lectures are availible everywhere..
They are availible because he has been reknowned as a lecturer for more than half a century. His bona fides do not need examination.
I would point out that confusion very often arises from misconceptions (for whatever reason) of simple ideas by the student, novice or advanced.

Yes, and by copying from earlier textbooks and writing new ones as bad as the old ones. All the confusion with Faraday's Law can simply be avoided by strictly sticking to the fact that electrodynamics is a field theory and the fundamental laws are the local differential Maxwell equations. Bringing them in integral form has to be done carefully for each special case, and it starts by carefully choosing the integration regions of the line-, surface-, and volume integrals needed. Then you'll never get in such useless discussions about misconceptions, particularly if also moving pieces are involved (e.g., for generators and motors).

BTW: That's what makes the Feynman lectures so valuable. He really carefully wrote it from scratch and often found better explanations for subjects often confused by the more traditional books. Of course there are also exceptions (e.g., the relativistic treatment of the straight DC-conducting wire ;-)).

 

[hutchphd]

That's what makes the Feynman lectures so valuable.

And yet as Feynman himself has admitted they were not particularly successful (popular? testable? advantageous?) as freshman texts even at Caltech. I have a well-worn set on my shelf, but there was a price to pay for that relentless devotion to never settling for the glib answer. I am fond of his (lengthy!) address to the National Science Teachers Association

 

[Sam Gallagher]

Classical electrodynamics books seem to suffer a bit from whisper-down-the-lane syndrome. Certain ideas are very carefully put in context in older texts (e.g. Maxwell, Jeans, Smythe) but later the context becomes more lax, and derivations which have clear need for more precise meaning are left unsaid. I think part of it comes from a desire for generality, and putting too many conditions on a result gives it the appearance of being too specific. Other times, it's due to the level of physical sophistication of the author, that they don't consider it necessary to make clear certain points, because it should be familiar or evident.

Some examples, Maxwell defines the electric field in Treatise vol 1 article 68 as:

In order to simplify the mathematical process, it is convenient to consider the action of an electrified body, not on another body of any form, but on an indefinitely small body, charged with an indefinitely small amount of electricity, and placed at any point of the space to which the electrical action extends. By making the charge of this body indefinitely small we render insensible its disturbing action on the charge of the first body.
...
Definition. The Resultant electric Intensity at any point is the force which would be exerted on a small body charged with the unit of positive electricity, if it were placed there without disturbing the actual distribution of electricity.

Compare this with Halliday and Resnick (8th ed) describing the same:

In principle, we can define the electric field at some point near the charged object, such as point P in Fig. 22-1a, as follows: We first place a positive charge q0, called a test charge, at the point. We then measure the electrostatic force F that acts on the test charge. Finally, we define the electric field E at point P due to the charged object as E=F/q0.
...
(We assume that in our defining procedure, the presence of the test charge does not affect the charge distribution on the charged object, and thus does not alter the electric field we are defining.)

The changes are subtle but (in my opinion) very important to a student. Things Maxwell emphasizes are different from Halliday and Resnick, for example he makes clear:
1. The electric field is a mathematical convenience
2. We define the force not on any body, but on a specific extremely small body (no unnecessary terminology as test charge)
3. A formal definition is given after the context is set.
Halliday and Resnick, by trying to make the introduction more intuitive, end up leaving out small details, and introduce new terminology which doesn't contribute to better understanding. From H&R it's not clear what the electric field is even though they try to explain it intuitively, while in Maxwell, despite being older and less refined, it's much more clear why we're defining it this way. Not because that's how physicists define it, but because it's convenient to define it that way.
OK I've ranted long enough, and it's all subjective in the end so I better not get too carried away, but that's my 2c!

PS sorry for hijacking your thread @alan123hk , I'm done now I promise!

 

[hutchphd]

Not because that's how physicists define it, but because it's convenient to define it that way.

I like Maxwell's exposition. Of course because of his seminal position he had the luxury of using whatever words he desired. Halliday and Resnick et al are in a slightly different positon. To teach classical EM fields without ever introducing the concept (and appellation) "test charge" would be negligent. There are many such constraints on the textbook author and physics professor and so the comparison is not really fair. Sometimes it is even important to teach bad physics as a cautionary tale (note I did not say teach physics badly!).

 

[vanhees71]

And yet as Feynman himself has admitted they were not particularly successful (popular? testable? advantageous?) as freshman texts even at Caltech. I have a well-worn set on my shelf, but there was a price to pay for that relentless devotion to never settling for the glib answer. I am fond of his (lengthy!) address to the National Science Teachers Association

Well, yes. They are no freshmen texts. I think they are a very good second textbook in the beginning theory curriculum, i.e., mechanics, electrodynamics, and non-relativistic QM.

 

[SDL]

In this video, he makes the trick at 11:37: "of course the secondary winding of the transformer is actually two wires between the two resistors". Indeed this is false: an inductor as a lumped element incapsulates Faraday's law and gives voltage and current relation as u = Ldi/dt. Having substituded the inductor with just a wire, he lost 1V of voltage drop around the loop which he was trying to find throughout the rest of the video. Of course, Ohm's law across all perfectly conducting wires would always give zero voltage drop.

 

[alan123hk]

Simply put, there is no contradiction, there is no dispute.

Kirchhoff's laws deal with voltages caused by accumulated charge in a circuit, and currents caused by changes in accumulated charge in a circuit.

We first replace all the induced electromotive forces that affect the circuit with models such as AC sources, inductors, and capacitors, and then assume that the induced electromotive force caused by current changes and charge accumulation changes will no longer affect the circuit from now on.

Therefore, the electric field in a circuit dealt with by Kirchhoff's laws has no curl, so the sum of the loop voltages must be zero.

 

[SDL]

Absolutely. Lumped element circuit model assumes that all time-varyinng magnetic fields (and non-potential EMF) are "hidden" inside rigid-body elements, leaving only potential electric fields outside and making KVL valid.

 

[alan123hk]

Yes, this is the method applied in engineering. For a more complex electromagnetic induction system, in order to simplify the analysis, it can also be transformed into a more complex circuit for processing. For example, it may contain multiple inductors, and there may also be complex mutual inductance relationships between them. This makes the simulation results of the circuit more and more close to the real situation.

 

[hutchphd]

Ptolemy did OK with epicycles, but (with hindsight) it was not good Physics.