- #1
gloo
- 261
- 2
My question is, for the diagram attached. The set up is:
1. The object (gray), including the handle , weighs 2000 Newtons
2. The object is not open to the water below it but there is an air pipe that allows for air above to fill in the pipe
3. The object is snug against the wall (in black), so as to not allow water to seep between and into the pipe area
If a constant upward force of 2000 Newtons is used to pull the entire object up so the bottom of the object is at Level A, is the force large enough? I assume that the water above it is not lifted but gets moved to the side as it "falls" over the top of the object. (Assume there is zero friction).
I know the speed will be reduced, but will the constantly applied force large enough to eventually lift the bottom of the object to Level A?
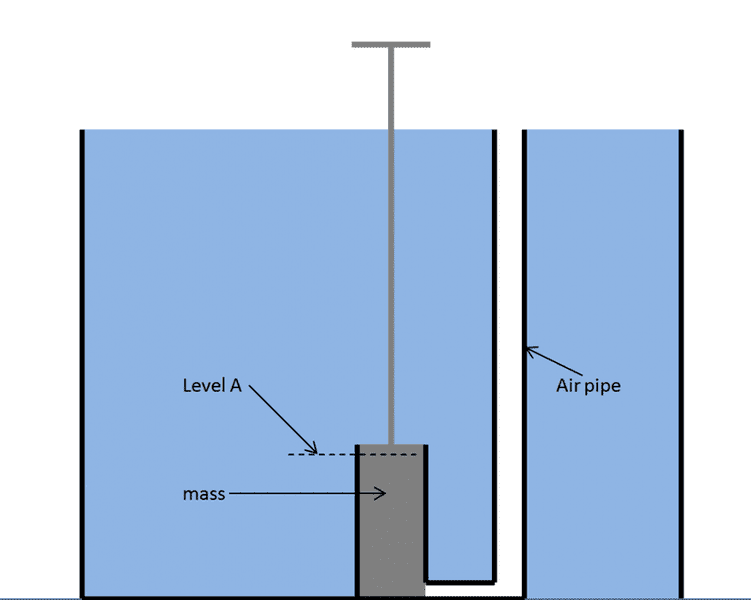
1. The object (gray), including the handle , weighs 2000 Newtons
2. The object is not open to the water below it but there is an air pipe that allows for air above to fill in the pipe
3. The object is snug against the wall (in black), so as to not allow water to seep between and into the pipe area
If a constant upward force of 2000 Newtons is used to pull the entire object up so the bottom of the object is at Level A, is the force large enough? I assume that the water above it is not lifted but gets moved to the side as it "falls" over the top of the object. (Assume there is zero friction).
I know the speed will be reduced, but will the constantly applied force large enough to eventually lift the bottom of the object to Level A?