- #1
gonegahgah
- 376
- 0
Hi Pervect
I have done up a couple of diagrams which I was hoping to get your input on.
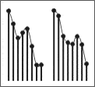
I have looked into the example that is given where light is sent through an elevator that is accelerating. It describes a curved path and this is used to depict the path of light under gravity due to the principle of equivalence.
I have done up some diagrams of this however mine show light as I believe it should be shown; as an oscillation through a point in space; and not as a particle as is shown in the texts.
The results are interesting. Please have a look and let me know if I can say what I think the results show.
One of the interesting results is that mine depicts the red shifting that occurs under gravity whereas theirs don't.
I have done up a couple of diagrams which I was hoping to get your input on.
I have looked into the example that is given where light is sent through an elevator that is accelerating. It describes a curved path and this is used to depict the path of light under gravity due to the principle of equivalence.
I have done up some diagrams of this however mine show light as I believe it should be shown; as an oscillation through a point in space; and not as a particle as is shown in the texts.
The results are interesting. Please have a look and let me know if I can say what I think the results show.
One of the interesting results is that mine depicts the red shifting that occurs under gravity whereas theirs don't.