Light Refraction, Biconvex Lens, Diagram Help

[Bluesy]

TL;DR Summary

Refraction Diagram Help

I've read that the refraction of light at the boundary of a medium can be described as follows:

-a line of connected people marching. one side of the line enters mud, and slows down. This causes the non-mud side to pivot towards the slower side, which then causes the line to change direction.

I'm helping my 9 year old with a science fair poster diagram. It's not a super competitive science fair; it's more for kids to explore ideas and have fun. In my ignorance I don't want to lead him too far astray.

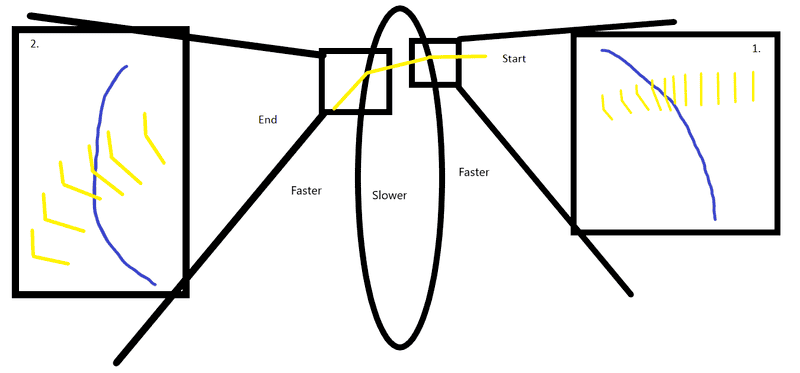
In the draft diagram below, the yellow parallel lines are supposed to be the wavefront of light.

Box 1 is supposed to illustrate the bending of the wave as it enters the medium at an angle.

Box 2 is supposed to illustrate the second redirection of the wave as it exits the medium at an agle.

Questions:

-For a simple diagram, would the waves exiting the medium (box 2) keep their "bent" shape? The diagram is supposed to reflect the exiting waves bending more at the second boundary than they do at the first boundary. We're not sure if the exiting waves should return to parrellel or keep their bent shap.

-Is the pivoting idea a good analogy to use? Is it even accurate? I guess the bending of the waves has to do with the oscillation and interference of electrons in the medium with the light, which then slows down the waves...I'm not sure if the "dashed" vertical lines below are okay to use an examples of the waves? In reality, would each dash be past the length of the lense (assuming maybe 7 inch lense?). I guess the wavelength of visible light is much smaller (?); I guess it's a microscopic boundary when the bending first starts occuring (i.e. a tiny wave, and tiny bend in the medium's surface where the bending occurs)?

Thank you!

 

[berkeman]

[Note: thread title was edited from Bicocave to Biconvex after my comment below]

Welcome to PF.

You mention a Biconcave lens, but what you have drawn is a Biconvex lens:

https://www.msnucleus.org/membership/html/jh/biological/microscopes/lesson2/microscopes2b.html

It's best to use Snell's Law to describe the bending of light by lenses, but that involves a little trigonometry which may not have been covered in your son's math classes so far.

https://en.wikipedia.org/wiki/Snell's_law

You can visualize the bending of the wavefronts as due to the different speed of the light waves in the different media (faster in air, slower in the glass lens). This visualization for water waves and their speed variation with depth is helpful:

https://www.google.com/url?sa=i&url=https://quizlet.com/244923377/chapter-15-flash-cards/&psig=AOvVaw3fu1lmLlJmlpk1ft6_vAKp&ust=1682185167922000&source=images&cd=vfe&ved=0CBAQjRxqFwoTCNjg5KzCu_4CFQAAAAAdAAAAABAF

In your drawing, you should have the incoming light waves coming in from straight at the left, not inclined. Then show how when they hit the curved glass, they are "refracted" toward the centerline of the glass.

This shows the light rays (which are perpendicular to the wavefronts) are deflected by the lens for straight horizontal incoming light rays:

https://www.msnucleus.org/membership/html/jh/biological/microscopes/lesson2/microscopes2b.html

If you want to show how an image is formed by a Biconvex lens, you can use a figure more like the first image I posted above, where light rays from the Object are coming in at slight angles. You still get the same effect of the light rays getting refracted toward the centerline of the lens.

 

[Bluesy]

Thank you for the welcome! And thank for the reply!

Yes, my son keeps correcting me--I'm continually mixing up the words "concave" and "convex" during this project. I have corrected the title.

I think we would like to combine the water diagram you posted, since I really like the lines bending at the barrier, with the second lense diagram you posted, since it shows the light redirecting at both the entrance of the lense and at the exit.

Based on the water diagram you posted, I think we may show one line bending at the barriers, so to speak, and then the rest of the lines parallel, but at an angle.

So we would have the big picture (similar to the second lense diagram you posted) with "two zoomed in" boxes (similar to the water diagram) at the entry and exit.

My Microsoft Paint skills are a little rough... :) I meant to show the movement of the light (I should have included arrows) as going from Right to Left; so incoming from the right side of the diagram (so no declination/inclination) and travelling through at an angle and then exiting with another redirection.

It does seem like most diagrams have the light travelling from left to right; that's probably more intuitive. We will change it.

I was thinking of using 2" or 3" clips of yellow coated wire and hot glueing them to the poster for the "zoomed in" light wave boxes. We will see.

Hopefully we are on track. Clearly I do not have a science background so help is much-appreciated!

 

[kuruman]

Perhaps an easier way to explain it is this.

Known facts

1. Light travels from point A to point B in the shortest possible time.
2. Light travels the fastest in vacuum, almost as fast in air and considerably slower in transparent media, e.g. water, glass, plastic, etc.

It follows that light will go from A to B in a straight line if A and B are in the same medium. The question is, what path does light follow if it starts at A which is in air and gets to B which is in glass? Would it be a straight line from A to B or something else? We can reason out the answer qualitatively by considering the lifeguard problem.

A lifeguard at point A on a sandy shore sees a swimmer at point B in water in distress (see figure below). The lifeguard knows that he can move faster on sand than in water. What kind of path should the lifeguard follow in order to reach the swimmer in the shortest possible time?

First let's examine the straight line path in which the lifeguard reaches the water at point C and then goes straight to B. Clearly, he can shorten the total time if he trades 1 yard of swimming in water for 1 yard of running on sand. This means that he saves time by jumping in the water at a point to the right of point C.

OK, but how far to the right? Well, point D is straight across from the swimmer. If he goes to the right of D, both lengths running on sand and swimming in water increase, so there can be no gain in time.

Conclusion: The lifeguard must jump in the water at some point between C and D. The exact point depends on the ratio of the speeds on sand and in water. If this ratio is 1, i.e. the lifeguard moves at the same speed in the two media and C is the point of entry. That's because any other path is longer in total distance while the speeds are the same. The larger the ratio of speeds, the closer the point of entry to point D.

The lifeguard's speed on sand is analogous to the speed of light in vacuum (or air) and the liefeguard's speed in water is analogous to the speed of light in a transparent medium. The ratio of the speeds on sand to water is analogous to the index of refraction.

The math required for a quantitative description of how to determine the point of entry can be found in post #8 here.

 

[berkeman]

My Microsoft Paint skills are a little rough... :)

Do you have access to Visio or any other slightly better drawing software?

BTW, you may already be planning on including it, but it's always good to include a list of the references that you and your son used in putting together the report/project. So if you like the water waves diagram that I posted, you could include the URL of that website in your References section, and so on.

 

[Ibix]

I'm continually mixing up the words "concave" and "convex" during this project.

"Concave curves inwards like a cave" is my mnenonic.

 

[berkeman]

BTW @Bluesy -- I did a google search on animation of light waves through lens and got lots of good hits. Maybe try that search or click on this link to see the hit list:

https://www.google.com/search?q=ani...2gEGCAEQARgU&sclient=gws-wiz-serp&bshm=foot/1

 

[Bluesy]

Do you have access to Visio or any other slightly better drawing software?

BTW, you may already be planning on including it, but it's always good to include a list of the references that you and your son used in putting together the report/project. So if you like the water waves diagram that I posted, you could include the URL of that website in your References section, and so on.

-We may trace/color the diagram directly on the poster. I'm not familiar with Visio but will check it out!

-Yes, we will include references--good call.

-Thank you for the search suggestions. I didn't even think to look at YouTube. The first video is great!

 

[Bluesy]

"Concave curves inwards like a cave" is my mnenonic.

Thank you! That is helpful.

 

[Bluesy]

Perhaps an easier way to explain it is this.

Known facts

1. Light travels from point A to point B in the shortest possible time.
2. Light travels the fastest in vacuum, almost as fast in air and considerably slower in transparent media, e.g. water, glass, plastic, etc.

It follows that light will go from A to B in a straight line if A and B are in the same medium. The question is, what path does light follow if it starts at A which is in air and gets to B which is in glass? Would it be a straight line from A to B or something else? We can reason out the answer qualitatively by considering the lifeguard problem.

A lifeguard at point A on a sandy shore sees a swimmer at point B in water in distress (see figure below). The lifeguard knows that he can move faster on sand than in water. What kind of path should the lifeguard follow in order to reach the swimmer in the shortest possible time?
View attachment 325254
First let's examine the straight line path in which the lifeguard reaches the water at point C and then goes straight to B. Clearly, he can shorten the total time if he trades 1 yard of swimming in water for 1 yard of running on sand. This means that he saves time by jumping in the water at a point to the right of point C.

OK, but how far to the right? Well, point D is straight across from the swimmer. If he goes to the right of D, both lengths running on sand and swimming in water increase, so there can be no gain in time.

Conclusion: The lifeguard must jump in the water at some point between C and D. The exact point depends on the ratio of the speeds on sand and in water. If this ratio is 1, i.e. the lifeguard moves at the same speed in the two media and C is the point of entry. That's because any other path is longer in total distance while the speeds are the same. The larger the ratio of speeds, the closer the point of entry to point D.

The lifeguard's speed on sand is analogous to the speed of light in vacuum (or air) and the liefeguard's speed in water is analogous to the speed of light in a transparent medium. The ratio of the speeds on sand to water is analogous to the index of refraction.

The math required for a quantitative description of how to determine the point of entry can be found in post #8 here.

Great explanation, thank you!