member 731016
- Homework Statement
- Please see below
- Relevant Equations
- Please see below
For this problem,
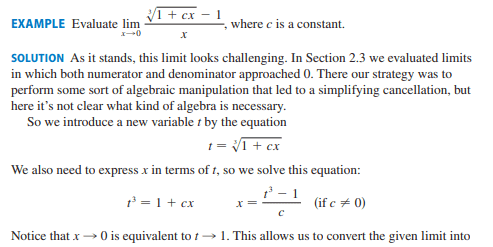
Did they get ## x## approaches one is equivalent to ##t## approaches zero because ##t ∝ (x)^{1/3} + 1##?
Many thanks!
Did they get ## x## approaches one is equivalent to ##t## approaches zero because ##t ∝ (x)^{1/3} + 1##?
Many thanks!
Last edited by a moderator: