Limit problem involving two circles and a line

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

For this problem,

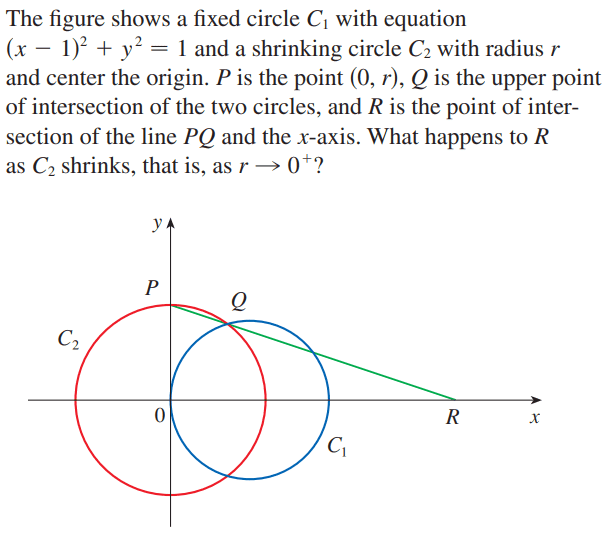
The limiting position of R is (4,0). However, I am trying to solve this problem using a method that is different to the solutions. So far I have got,

$C_1: (x - 1)^2 + y^2 = 1$
$C_2: x^2 + y^2 = r^2$

To find the equation of PQ,
$P(0,r)$ and $R(R,0)$
$y = \frac{r(x - R)}{-R}$
Then solve for $R$ to get,

$R = \frac{rx}{r - y}$
$R = \frac{rx}{r - \sqrt{r^2 + x^2}}$
$R = \lim_{r \rightarrow 0^+} \frac{rx}{r - \sqrt{r^2 + x^2}} = 0$

Can someone please give guidance to what I have done wrong?

Many thanks!

 

[fresh_42]

What did you get as coordinates for $Q$?

 

[member 731016]

What did you get as coordinates for $Q$?

Thank you for your reply @fresh_42! I missed up finding the coordinates of $Q$ in my original attempt.

But according to the solutions,

However, I am curious how to solve this problem without using Q's coordinates.

Many thanks!

 

[fresh_42]

You need the equation for the line $\overline{PQ}$ which is defined by $Q$ so you definitely need the coordinates for $Q$ somehow; if not explicitly then implicitly. Say the straight is $y=mx+b.$ Then $R$ has the coordinates $R=(-\frac{b}{m},0).$ We finally need to solve $\lim_{r \to 0^+} \frac{-b}{m}.$

I do not see how to get there without $m$ and $b$ that are determined by $P$ and $Q.$

 

[fresh_42]

Hint for the limit calculation:

a) Show that the quotient is greater than $2$ for $0<r < 2.$
b) Set the quotient equal to $L$ and solve for $r^2.$
c) Show what happens to $L$ if $r \rightarrow 0.$

 

[member 731016]

You need the equation for the line $\overline{PQ}$ which is defined by $Q$ so you definitely need the coordinates for $Q$ somehow; if not explicitly then implicitly. Say the straight is $y=mx+b.$ Then $R$ has the coordinates $R=(-\frac{b}{m},0).$ We finally need to solve $\lim_{r \to 0^+} \frac{-b}{m}.$

I do not see how to get there without $m$ and $b$ that are determined by $P$ and $Q.$

Thank you for your replies @fresh_42!

I think part of my confusion is that I think that the line $\overline{PQ} = \overline{PR}$. Do you please know why they are different?

I also think I wrongly wrote $R$ as $R(R,0)$, because I was thinking that the distance from the origin to point $R$ is $R$. I now think that is incorrect because the problem only specifies the point as $R$. Do you please know whether that is the only reason why we cannot assume that $R(R,0)$?

Many thanks!

 

[member 731016]

You need the equation for the line $\overline{PQ}$ which is defined by $Q$ so you definitely need the coordinates for $Q$ somehow; if not explicitly then implicitly. Say the straight is $y=mx+b.$ Then $R$ has the coordinates $R=(-\frac{b}{m},0).$ We finally need to solve $\lim_{r \to 0^+} \frac{-b}{m}.$

I do not see how to get there without $m$ and $b$ that are determined by $P$ and $Q.$

Sorry, do you please know what it means to find the coordinates of $Q$ explicitly or implicitly?

Would finding the coordinates of $Q$ explicitly translate to find $Q$ as a function of some variables, for example $R=(-\frac{b}{m},0)$ like you wrote?

And finding coordinates of $Q$ implicitly would translate to $Q$ as a function of some other variables?

Many thanks!

 

[fresh_42]

Thank you for your replies @fresh_42!

I think part of my confusion is that I think that the line $\overline{PQ} = \overline{PR}$. Do you please know why they are different?

They are not. $P$ and $R$ change when the left circle shrinks. They, and $Q$, depend on its radius $r.$

I also think I wrongly wrote $R$ as $R(R,0)$, because I was thinking that the distance from the origin to point $R$ is $R$.

That is both true. $R=(R,0).$ A better notation would be $R=(R(r),0)$ as the coordinate changes with $r.$

I now think that is incorrect because the problem only specifies the point as $R$. Do you please know whether that is the only reason why we cannot assume that $R(R,0)$?

Many thanks!

You are almost done. You correctly calculated $Q=\left(\dfrac{r^2}{2}\, , \,\dfrac{r}{2}\sqrt{4-r^2}\right).$

The rest is not so difficult. The equation for a line given by points $A=(x_a,y_a)$ and $B=(x_b,y_b)$ is
$$
\dfrac{y-y_a}{x-x_a}=\dfrac{y_b-y_a}{x_b-x_a}
$$
So calculate this expression with $A=P$ and $B=Q,$ bring it into the form $y=m\cdot x+ b$ and calculate $R=(x_0,0)=(x_0,m\cdot x_0+b),$ i.e. 0=m\cdot x_0+b$or$x_0=-\dfrac{b}{m}=R(r).$This gives you the$x$-coordinate of the point$R$as a function of$r,## the radius of the left circle.
Then proceed along the lines in post #5 in order to find the limit mentioned in post #4.

 

[fresh_42]

Sorry, do you please know what it means to find the coordinates of $Q$ explicitly or implicitly?

You have computed the coordinates of $Q$. Correctly calculated them! So you can work with these formulas, which would be an explicit use.

We need the information hidden in the position of $Q$ because it defines our problem. We need $Q.$ If you do not want to use the coordinates, the result of your calculation, then you have to use $Q$ otherwise. That would be an implicit use since you had to use the definition of $Q$ without calculating it. Such usage is called implicit use since you avoid the explicit solution. I have no idea how to do that and consider it unnecessary especially as you do have the coordinates already. I just answered your idea of doing it without knowing the coordinates.

E.g. The point $R$ is implicitly given. We know how to construct it since we have a description of the algorithm, but we do not know the explicit coordinates, yet.

Calculate them next: $R=\left(-\dfrac{b}{m},0\right).$ What are $m$ and $b$ as a function of $r$?

Would finding the coordinates of $Q$ explicitly translate to find $Q$ as a function of some variables, for example $R=(-\frac{b}{m},0)$ like you wrote?

And finding coordinates of $Q$ implicitly would translate to $Q$ as a function of some other variables?

Many thanks!