- #1
Faiq
- 348
- 16
What does the line in the rectangle box means? (What is the difference between limiting friction and centripetal frictional force?)
What type of motion is meant by skidding?
http://prnt.sc/c7ptm8
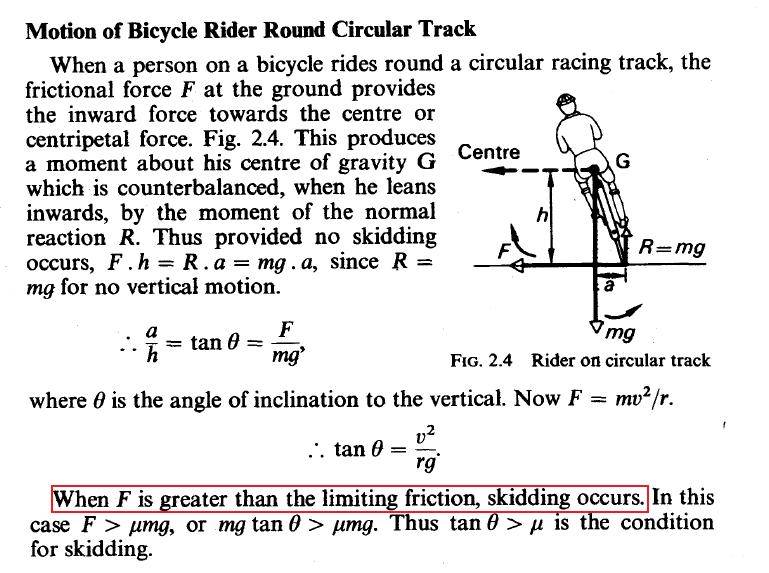
What type of motion is meant by skidding?
http://prnt.sc/c7ptm8
Last edited by a moderator: