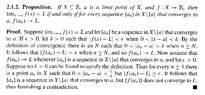Limits of Functions ....Conway, Proposition 2.1.2 .... ....

[Math Amateur]

I am reading John B. Conway's book: A First Course in Analysis and am focused on Chapter 2: Differentiation ... and in particular I am focused on Section 2.1: Limits ...

I need help with an aspect of the proof of Proposition 2.1.2 ...Proposition 2.1.2 and its proof read as follows:
View attachment 9496

In the above proof by Conway we read the following:

" ... ... Now assume that \(\displaystyle f(a_n) \to L\) whenever \(\displaystyle \{ a_n \}\) is a sequence in \(\displaystyle X \) \ \(\displaystyle \{a\}\) that converges to \(\displaystyle a\), and let \(\displaystyle \epsilon \gt 0\). Suppose no \(\displaystyle \delta \gt 0\) can be found can be found tho satisfy the definition. ... ... "
Above Conway seems to me that he is assuming that \(\displaystyle f(a_n) \to L\) and then assuming that the definition of f(a_n) \to L doesn't hold true ... which seems invalid ...

Can someone explain Conway's logic ... can someone please explain what is actually being done in this part of the proof ...

Peter

 

[jvicens]

Dear Peter,

Thank you for reaching out for help with Proposition 2.1.2 in Conway's book. I can understand how this part of the proof may seem confusing to you. Let me try to explain Conway's logic in this section.

Firstly, in this part of the proof, Conway is trying to show that if the definition of f(a_n) \to L does not hold true, then there exists no \delta \gt 0 that satisfies the definition. In other words, he is trying to prove the contrapositive of the statement.

Secondly, in order to prove the contrapositive, Conway is assuming the opposite of the statement, which is that f(a_n) \to L does not hold true. This means that there exists some \epsilon \gt 0 such that for every \delta \gt 0, there exists a sequence \{ a_n \} in X \ \{a\} that converges to a, but f(a_n) is not within \epsilon of L.

Finally, Conway is showing that this assumption leads to a contradiction, which means that the original statement must be true. He does this by showing that for any \epsilon \gt 0, there exists a \delta \gt 0 that satisfies the definition, which contradicts our assumption.

I hope this explanation helps you understand Conway's logic in this part of the proof. If you have any further questions, please do not hesitate to ask.